A parallel plate capacitor with air between the plates has a capacitance of 10 pF. The capacitance, if the distance bgetween the plates is reduced by half and the space between tehm is filled with a substance of dielectric constant 4 is
80pF
96pF
100pF
120pF
The correct Answer is:A
To solve the problem, we need to determine the new capacitance of a parallel plate capacitor when the distance between the plates is halved and the space between them is filled with a dielectric material of dielectric constant k=4.
1. Understanding the Formula for Capacitance:
The capacitance C of a parallel plate capacitor is given by the formula:
C=k⋅A⋅ϵ0d
where:
- C is the capacitance,
- k is the dielectric constant,
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
2. Initial Conditions:
We know that the initial capacitance C is 10 pF (picoFarads) with air between the plates, which means k=1 (for air). Therefore, we can write:
C=1⋅A⋅ϵ0d=10 pF
3. New Conditions:
In the new scenario, the distance d is reduced by half, so the new distance d′ is:
d′=d2
The dielectric constant of the new material is k=4.
4. Calculating the New Capacitance:
We can now calculate the new capacitance C′ using the modified distance and dielectric constant:
C′=k⋅A⋅ϵ0d′
Substituting d′:
C′=4⋅A⋅ϵ0d2=4⋅A⋅ϵ0⋅2d=8⋅A⋅ϵ0d
5. Relating New Capacitance to Initial Capacitance:
From the initial capacitance equation, we know:
A⋅ϵ0d=C
Therefore, we can express C′ in terms of C:
C′=8⋅A⋅ϵ0d=8C
Substituting C=10 pF:
C′=8⋅10 pF=80 pF
Final Answer:
The new capacitance C′ is 80 pF.
---
The capacitance C of a parallel plate capacitor is given by the formula:
C=k⋅A⋅ϵ0d
where:
- C is the capacitance,
- k is the dielectric constant,
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the distance between the plates.
We know that the initial capacitance C is 10 pF (picoFarads) with air between the plates, which means k=1 (for air). Therefore, we can write:
C=1⋅A⋅ϵ0d=10 pF
In the new scenario, the distance d is reduced by half, so the new distance d′ is:
d′=d2
The dielectric constant of the new material is k=4.
We can now calculate the new capacitance C′ using the modified distance and dielectric constant:
C′=k⋅A⋅ϵ0d′
Substituting d′:
C′=4⋅A⋅ϵ0d2=4⋅A⋅ϵ0⋅2d=8⋅A⋅ϵ0d
From the initial capacitance equation, we know:
A⋅ϵ0d=C
Therefore, we can express C′ in terms of C:
C′=8⋅A⋅ϵ0d=8C
Substituting C=10 pF:
C′=8⋅10 pF=80 pF
The new capacitance C′ is 80 pF.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
Similar Questions
A parallel plate capacitor with air between the plates has a capacitance of 8 pF.(1pF=10−12F) What will be the capacitance if the distance between the plates is reduced by half and the space between them is filled with a substance of dielectric constant 6 ?
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View Solution
Knowledge Check
As the distance between the plates of a parallel plate capacitor decreased
AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false.
The ratio between masses of two planets is 3 : 5 and the ratio between their radii is 5 : 3. The ratio between their acceleration due to gravity will be
View SolutionA parallel plate capacitor with air between the plates has capacitance of 9pF. The separation between its plates is 'd'. The space between the plates is now filled with two dielectrics. One of the dielectrics has dielectric constant k1=3 and thickness d3 while the other one has dielectric constant k2=6 and thickness 2d3. Capacitance of the capacitor is now
View SolutionA parallel plate capacitor with air between the plates has capacitance of 9pF. The separation between its plates is 'd'. The space between the plates is now filled with two dielectrics. One of the dielectrics has dielectric constant k1=3 and thickness d3 while the other one has dielectric constant k2=6 and thickness 2d3. Capacitance of the capacitor is now
View SolutionTo reduce the capacitance of parallel plate capacitor, the space between the plate is
View SolutionThe capacity of a parallel plate condenser without any dielectric is C. If the distance between the plates is doubled and the space between the plates is filled with a substance of dielectric constant 3, the capacity of the condenser becomes:
View SolutionA parallel plate capacitor with air as medium between the plates has a capacitance of 10μF. The area of capacitor is divided into two equal halves and filled with two media as shown in the figure having dielectric constnt k1=2 and k2=4. the capacitance of the system will now be
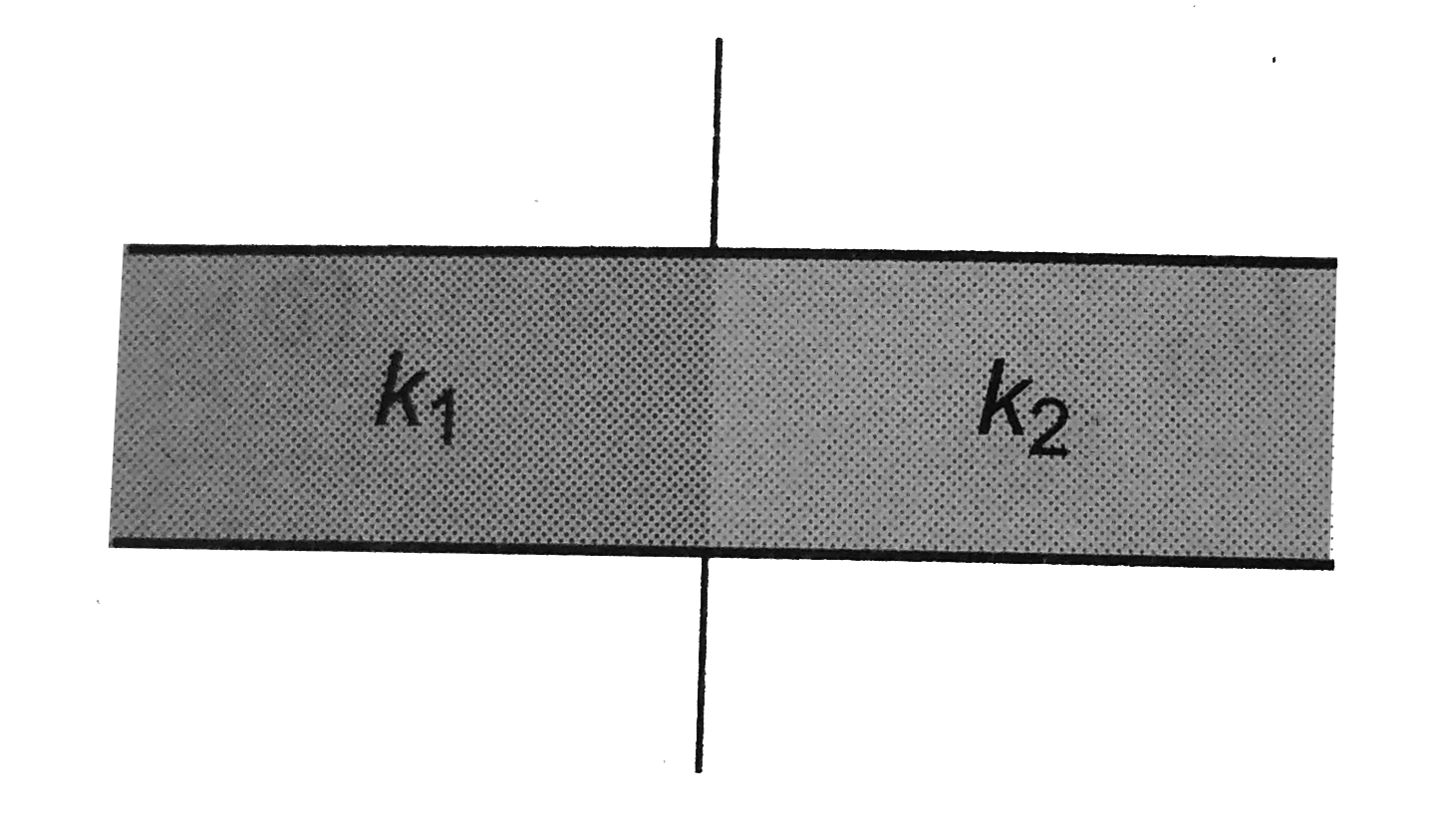
View SolutionA parallel plate capacitor of capacitance C is charged to a potential V and then disconnected with the battery. If separation between the plates is decreased by 50% and the space between the plates is filled with a dielectric slab of dielectric constant K=10. then
View Solution
