Question

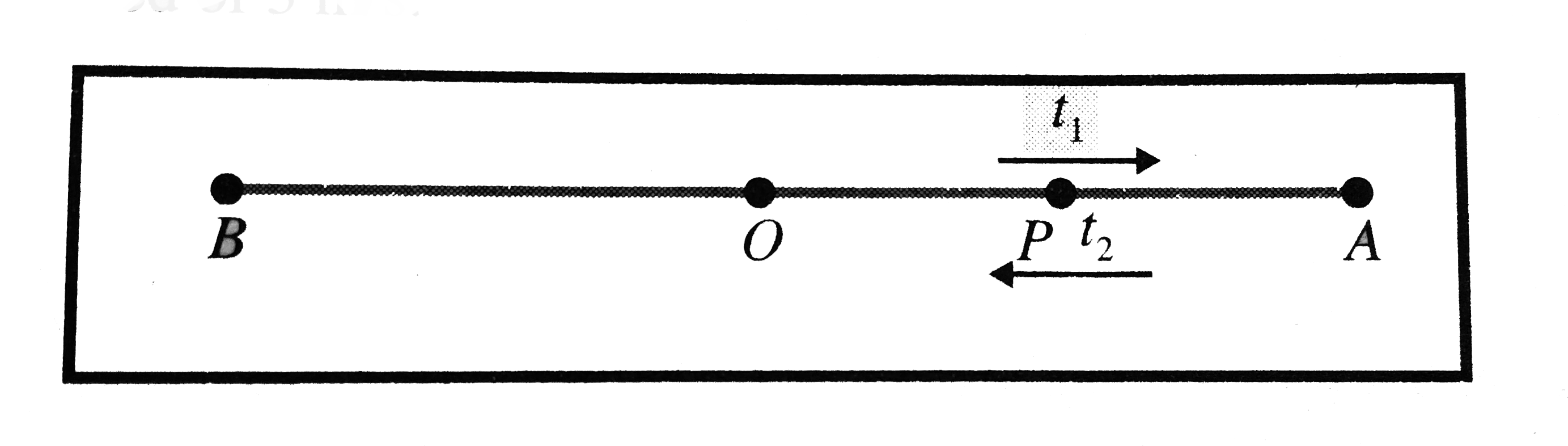
A particle is executing SHM on a straight line. A and B are two points at which its velocity is zero. It passes through a certain point P(APltBP) at successive intervals of 0.5s and 1.5 s with a speed of 3m/s.

Options

A. a. the maximum speed of particle is `3sqrt(2)`m/s
B. b. the maximum speed of particle is `sqrt(2)m/s`.
C. c. the ratio (AP/BP) is `(sqrt(2)-1)/(sqrt(2)+1)`
D. d. the ratio AP/BP is `1/sqrt(2)`

Answer

A::C

Explanation

To solve the problem step by step, we will analyze the motion of the particle executing Simple Harmonic Motion (SHM) and derive the required values.

Step 1: Understand the Motion
The particle is executing SHM, and points A and B are the extreme positions where the velocity is zero. The point P is between A and B, and the particle passes through P at intervals of 0.5 seconds and 1.5 seconds with a speed of 3 m/s.

Step 2: Determine the Time Period
The total time taken to go from P to A and back to P is 0.5 seconds, and from P to B and back to P is 1.5 seconds. Therefore, the total time period \( T \) of the SHM can be calculated as:
\( T = 0.5 + 1.5 = 2 \text{ seconds} \)

Step 3: Calculate Angular Frequency
The angular frequency \( \omega \) is given by the formula:
\( \omega = \frac{2\pi}{T} \)
Substituting the value of \( T \):
\( \omega = \frac{2\pi}{2} = \pi \text{ rad/s} \)

Step 4: Write the Velocity Equation
The velocity \( v \) in SHM is given by:
\( v = A \omega \cos(\omega t + \phi) \)
At point P, the velocity is given as 3 m/s. Therefore, we can write:
\( 3 = A \cdot \pi \cdot \cos(\phi) \)

Step 5: Analyze the Motion at Points A and B
At points A and B, the velocity is zero. This occurs when:
\( \cos(\omega t + \phi) = 0 \)
This implies:
\( \omega t + \phi = \frac{\pi}{2} + n\pi \quad (n \in \mathbb{Z}) \)

Step 6: Determine the Phase Difference
From the time taken to reach A from P (0.25 seconds), we can set up the equation:
\( \omega \cdot 0.25 + \phi = \frac{\pi}{2} \)
Substituting \( \omega = \pi \):
\( \pi \cdot 0.25 + \phi = \frac{\pi}{2} \)
This simplifies to:
\( \phi = \frac{\pi}{2} - \frac{\pi}{4} = \frac{\pi}{4} \)

Step 7: Substitute Phase Difference to Find Amplitude
Now substitute \( \phi \) back into the velocity equation:
\( 3 = A \cdot \pi \cdot \cos\left(\frac{\pi}{4}\right) \)
Since \( \cos\left(\frac{\pi}{4}\right) = \frac{1}{\sqrt{2}} \):
\( 3 = A \cdot \pi \cdot \frac{1}{\sqrt{2}} \)
Solving for \( A \):
\( A = \frac{3\sqrt{2}}{\pi} \)

Step 8: Calculate Maximum Velocity
The maximum velocity \( V_{\text{max}} \) in SHM is given by:
\( V_{\text{max}} = A \cdot \omega \)
Substituting the values of \( A \) and \( \omega \):
\( V_{\text{max}} = \left(\frac{3\sqrt{2}}{\pi}\right) \cdot \pi = 3\sqrt{2} \text{ m/s} \)

Step 9: Calculate the Ratio \( \frac{AP}{BP} \)
Using the displacement equation for SHM:
\( x = A \sin(\omega t + \phi) \)
At point P, substituting \( t = 0 \):
\( x_P = A \sin\left(\frac{\pi}{4}\right) = A \cdot \frac{1}{\sqrt{2}} = \frac{3}{\sqrt{2}} \)
Thus, \( AP = A - x_P \) and \( BP = x_P + A \):
\( AP = A - \frac{3}{\sqrt{2}}, \quad BP = A + \frac{3}{\sqrt{2}} \)
Calculating the ratio:
\( \frac{AP}{BP} = \frac{A - \frac{3}{\sqrt{2}}}{A + \frac{3}{\sqrt{2}}} \)
Substituting \( A = \frac{3\sqrt{2}}{\pi} \):
\( \frac{AP}{BP} = \frac{\frac{3\sqrt{2}}{\pi} - \frac{3}{\sqrt{2}}}{\frac{3\sqrt{2}}{\pi} + \frac{3}{\sqrt{2}}} \)
This simplifies to:
\( \frac{AP}{BP} = \frac{\sqrt{2} - 1}{\sqrt{2} + 1} \)

Final Answers
1. The maximum speed of the particle is \( 3\sqrt{2} \) m/s.
2. The ratio \( \frac{AP}{BP} = \frac{\sqrt{2} - 1}{\sqrt{2} + 1} \).