A particle is moving in a straight line along the positive x-axis such that its speed is inversely proportional to the distance from origin [v∝1x⇒v=kx where k is the proportionally constant].
The graph of motion of the particle for 1/v versus x (distance from origin) is shown in the figure.
![A particle is moving in a straight line along the positive x-axis such that its speed is inversely proportional to the distance from origin [vprop1/x impliesv=k/x where k is the proportionally constant]. The graph of motion of the particle for 1//v versus x (distance from origin) is shown in the figure.](https://d10lpgp6xz60nq.cloudfront.net/physics_images/MPP_PHY_C02_S01_110_Q01.png)
The time interval of motion from point A to point B is 12.50
The time interval of motion from point A to point B is 18.75s.
The proportionality constant k is 10m2/s
The proportionality constant k is 20m2/s
The correct Answer is:B, C
Step by step video & image solution for A particle is moving in a straight line along the positive x-axis such that its speed is inversely proportional to the distance from origin [vprop1/x impliesv=k/x where k is the proportionally constant]. The graph of motion of the particle for 1//v versus x (distance from origin) is shown in the figure. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - COMPREHENSION_TYPE
19 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - MATCH THE COLUMN
10 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
Similar Questions
A particle is moving along a straight line parallel to x-axis with constant velocity. Find angular momentum about the origin in vector form :

View SolutionA particle is moving with speed v=b√x along positive x-axis. Calculate the speed of the particle at time t=τ (assume tha the particle is at origin at t= 0).
View SolutionFind the locus of a point which moves such that its distance from the origin is three times its distance from x-axis.
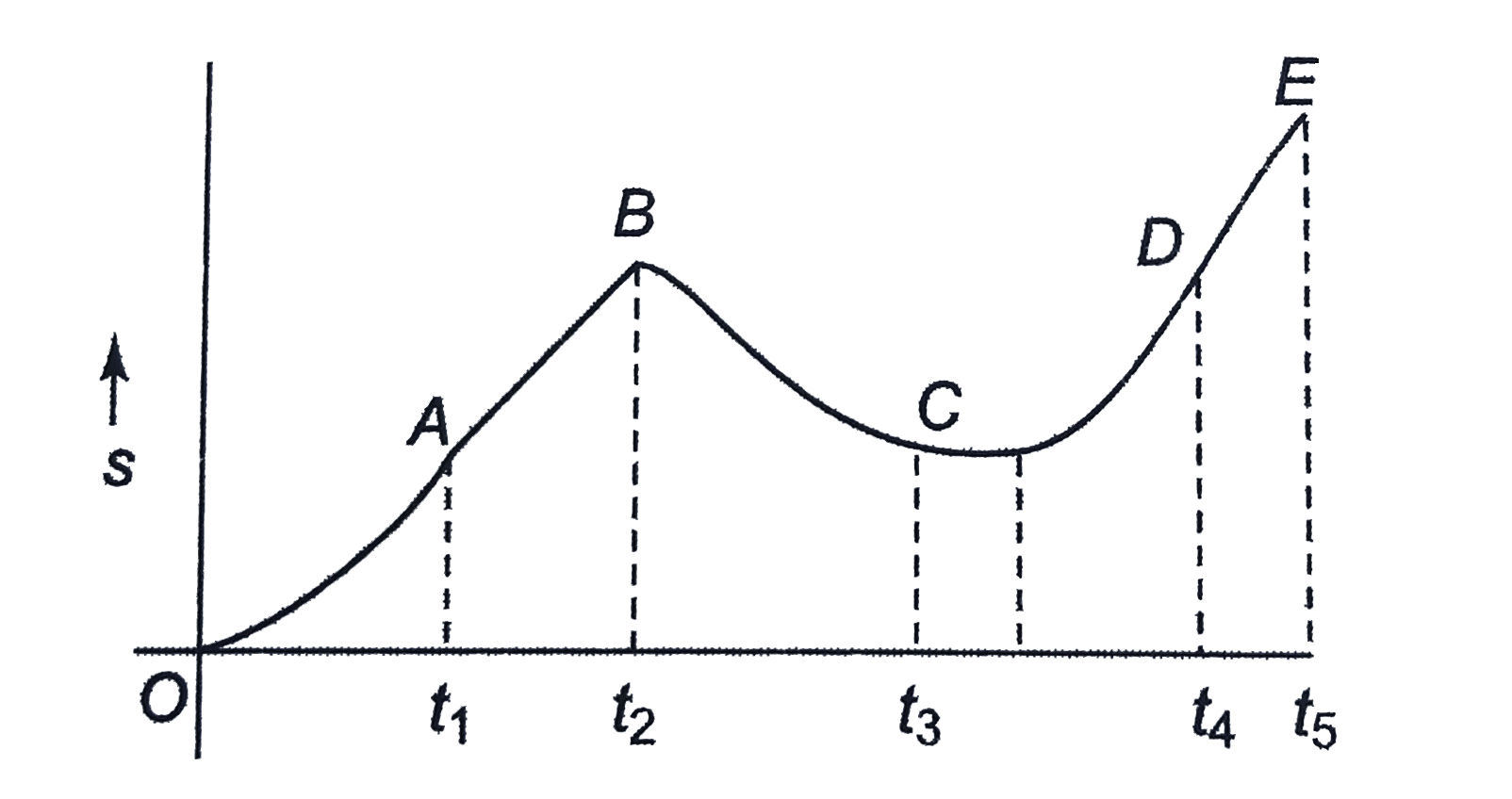
View SolutionA particle is moving along the x-axis and its position-time graph is shown. Determine the sign of acceleration.
View SolutionA particle is projected with velocity v0 along x - axis. The deceleration on the particle is proportional to the square of the distance from the origin i.e. a=−x2. The distance at which particle stops is -
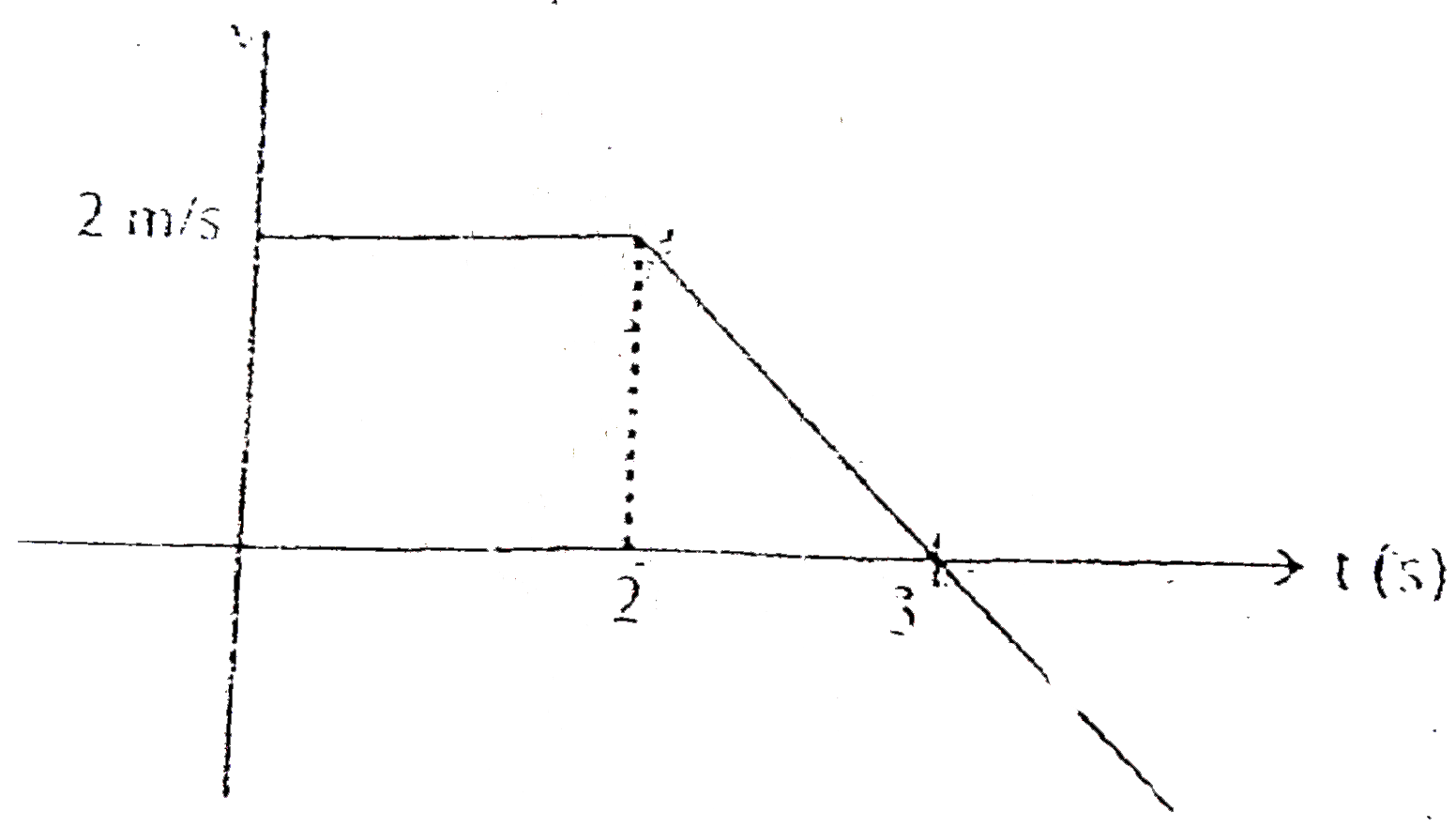
View SolutionA particle is moving on X−axis. Its velocity time graph is given in the figure. Find distance travelled by the particle in 5sec.
View SolutionA particle located at position x=0, at time t=0, starts moving along the positive x-direction with a velocity v2=αx (where alpha is a positive constant). The displacement of particle is proportional to
View SolutionA particle is moving parallel to x-axis as shown in the figure. The angular velocity of the particle about the origin is
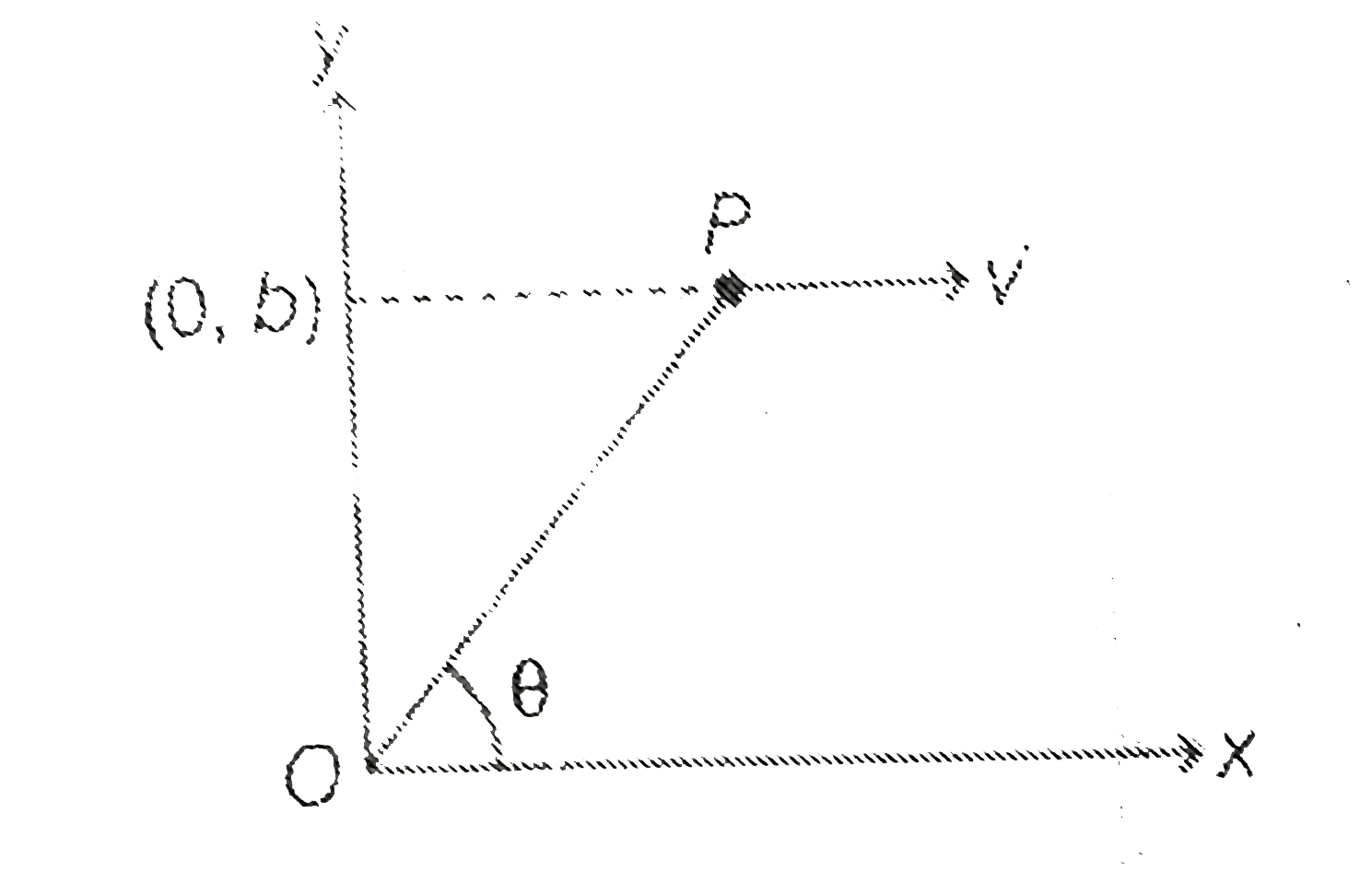
View SolutionA particle starts from the origin at time t = 0 and moves along the positive x-axis. The graph of velocity with respect to time is shown in figure. What is the position of the particle at time t = 5s ?

View SolutionA particle moves in a straight line from origin. Its velocity time curve is shown. Find the distance of particle at the =8sec. From the origin and the distance travelled by the particle during first 8 seconds.

View Solution
