A particle of mass 2×10−3 kg, charge 4×10−3C enters in an electric field of 5V/m, then its kinetic energy after 10 s is
0.1 J
1 J
10 J
100 J
The correct Answer is:C
To solve the problem step by step, we will follow the physics principles related to electric fields, forces, acceleration, and kinetic energy.
Step 1: Calculate the Force on the Particle
The force F experienced by a charged particle in an electric field is given by the formula:
F=qE
where:
- q=4×10−3C (charge of the particle)
- E=5V/m (electric field strength)
Substituting the values:
F=(4×10−3)×(5)=2×10−2N
Step 2: Calculate the Acceleration of the Particle
Using Newton's second law, the acceleration a can be calculated as:
a=Fm
where:
- m=2×10−3kg (mass of the particle)
Substituting the values:
a=2×10−22×10−3=10m/s2
Step 3: Calculate the Final Velocity after 10 seconds
Using the first equation of motion:
v=u+at
Assuming the initial velocity u=0 (the particle starts from rest), and substituting a=10m/s2 and t=10s:
v=0+(10)(10)=100m/s
Step 4: Calculate the Kinetic Energy
The kinetic energy KE of the particle is given by:
KE=12mv2
Substituting m=2×10−3kg and v=100m/s:
KE=12×(2×10−3)×(100)2
KE=12×(2×10−3)×(104)=12×20=10J
Final Answer
The kinetic energy of the particle after 10 seconds is 10J.
---
The force F experienced by a charged particle in an electric field is given by the formula:
F=qE
where:
- q=4×10−3C (charge of the particle)
- E=5V/m (electric field strength)
F=(4×10−3)×(5)=2×10−2N
Using Newton's second law, the acceleration a can be calculated as:
a=Fm
where:
- m=2×10−3kg (mass of the particle)
a=2×10−22×10−3=10m/s2
Using the first equation of motion:
v=u+at
Assuming the initial velocity u=0 (the particle starts from rest), and substituting a=10m/s2 and t=10s:
v=0+(10)(10)=100m/s
The kinetic energy KE of the particle is given by:
KE=12mv2
Substituting m=2×10−3kg and v=100m/s:
KE=12×(2×10−3)×(100)2
KE=12×(2×10−3)×(104)=12×20=10J
The kinetic energy of the particle after 10 seconds is 10J.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
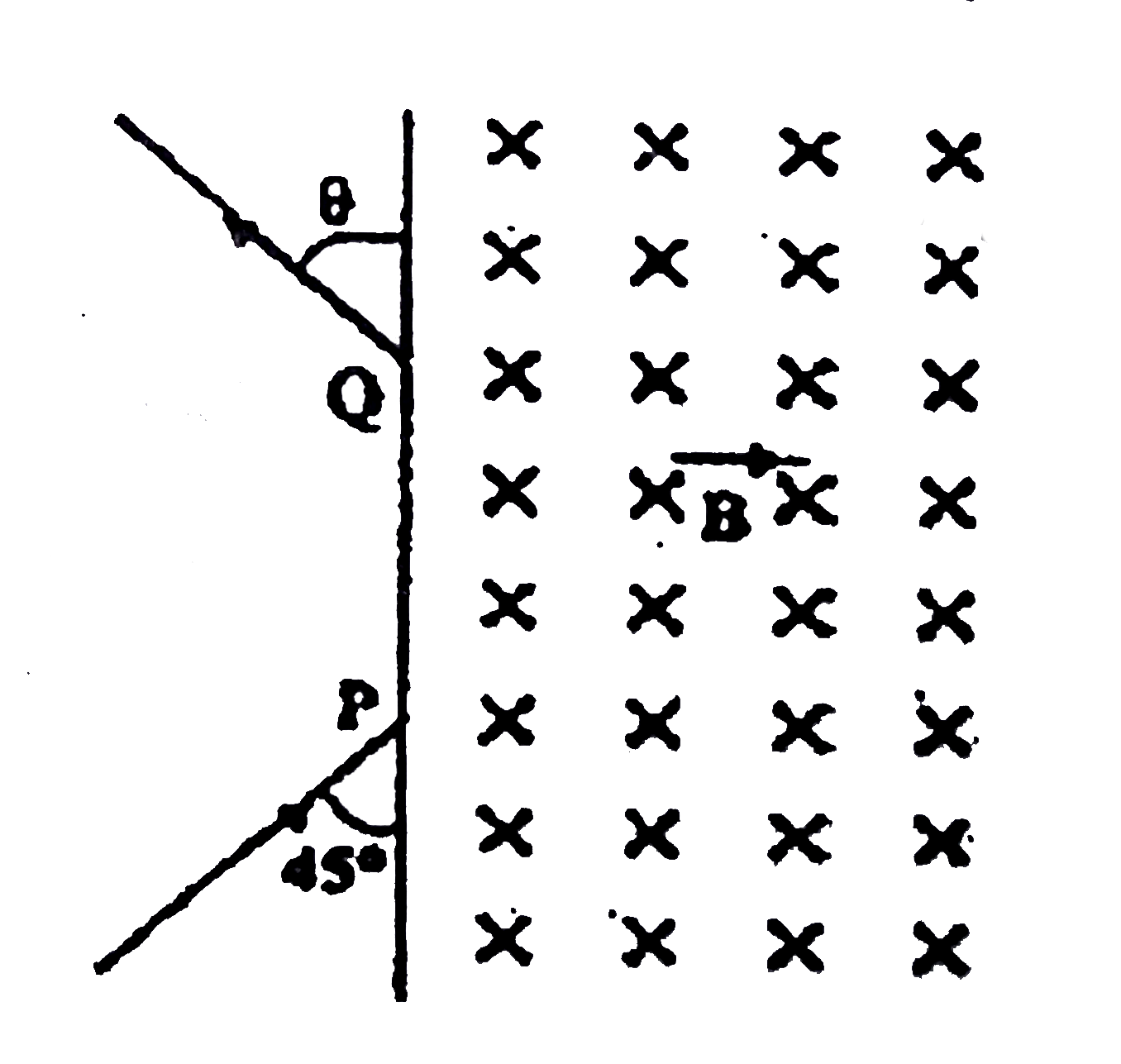
A particle of mass 1.6×10−27kg and charge 1.6×10−19 coulomb enters a uniform magnetic field of 1 Tesla as shown in the figure. The speed of the particle is 107m/s. The distance PQ will be
View SolutionA charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
View SolutionA particle of mass 1×10−26kg and charge +1.6×10−19C travelling with a velocity 1.28×106ms−1 in the +x direction enters a region in which uniform electric field E and a uniform magnetic field of induction B are present such that Ex=Ey=0,Ez=−102.4kVm−1,andBx=Bz=0,By=8×10−2. The particle enters this region at time t=0. Determine the location (x,y,z coordinates) of the particle at t=5×10−6s. If the electric field is switched off at this instant (with the magnetic field present), what will be the position of the particle at t=7.45×10−6s ?
View SolutionA particle of mass 1 g and charge 2.5×10−4C is released from rest in an electric field of 1.2×104NC−1 (a) Find the electric force and the force of gravity acting on this particle. Can one of these forces be neglected in comparison with the other for approximate analysis? (b) How long will it take for the particle to travel a distance of 40 cm? (c) What will be the speed of the particle after travelling this destance? (d) how much is the work done by electric force on the particle during this period?
View SolutionA uniform magnetic field of intensity 1T is applied in a circular region of radius 0.1m, directed into the plane of paper. A charged particle of mass 5×10−5kg and charge q=5×10−4C enters the field with velocity 1/√3m/s making an angle of ϕ with a radial line of circular region in such a way that it passes through centre of applied field the angle ϕ is
View SolutionA charged particle of mass 5×10−5kg is held stationary in space by placing it in an electric field of strength 107NC−1 directed vertically downwards. The charge on the particle is
View SolutionA proton of mass 1.67×10−27 kg and charge 1.6×10−19C is shot a uniform magnetic field, and perpendicular to the field with a velocity 5×106m/s. If the magnetic induction of the field is 1 tesla, find
(i) The radius of the circular path
View SolutionA charged particle of mass 10−3kg and charge 10−5C enters a magnetic field of induction 1 T. If g=10ms−2, for what value of velocity will it pass straight through the field without deflection?
View SolutionAn electron (mass =9.1×10−31kg, charge =1.6×10−19C) experiences no deflection if subjected to an electric field of 3.2x105Vm, and a magnetic fields of 2.0×10−3Wbm2. Both the fields are normal to the path of electron and to each other. If the electric field is removed, then the electron will revolve in an orbit of radius
View SolutionAn electron (mass =9.1×10−31kg, charge =1.6×10−19C) experiences no deflection if subjected to an electric field of 3.2x105Vm, and a magnetic fields of 2.0×10−3Wbm2. Both the fields are normal to the path of electron and to each other. If the electric field is removed, then the electron will revolve in an orbit of radius
View Solution
