A particle of mass m is attached to one end of missless rigid non-conducting rod of length l. Another particle of the same mass is attached to the other end of the rod. Two particles carry charges +q and -q . This arrangement is held in the region of uniform electric field E such that the rod makes and angle θ(<5∘) with the field direction. Find an expression for the minimum time that is needed for the rod to become parallel to the field after it is set free.
As the rod has two charges of equal magnitude (be opposite in nature), the torque acting on the rod,
τ=qE×lsinθ
or, τ=qElθ [∵θ<5∘]
We know, τ=Iα
[where I = moment of inertia and α = angular acceleration]
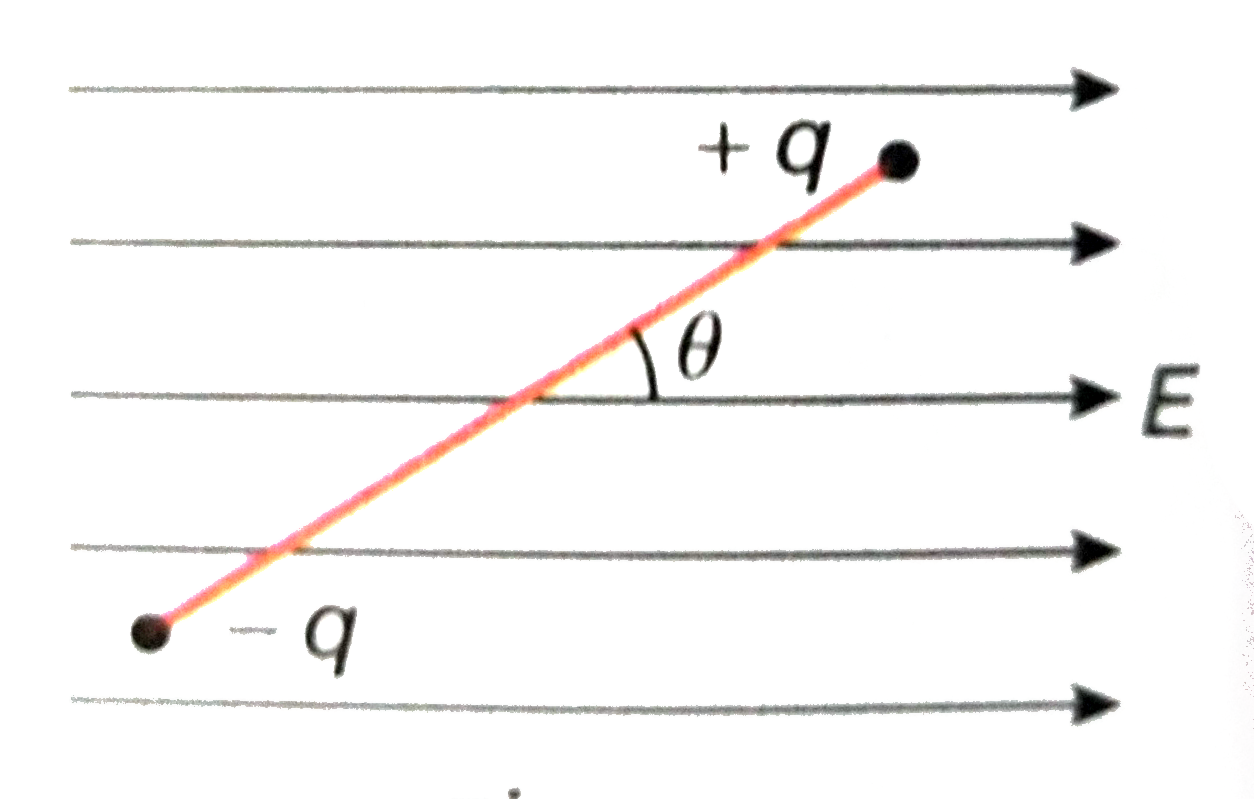
Hence, Iα=qElθor,α=qElθI
∴ Time period, T=2π√angular displacementangular acceleration=2π√IqEl
Now, moment of inertia of the dipole,
I=[(l2)2+(l2)2]m=12ml2
∴T=2π√ml22qEl=2π√ml2qE
∴ Required time, t=14×time period=T4=π2√ml2qE
Topper's Solved these Questions
- ELECTRIC FIELDBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - NCERT TEXTBOOK QUESTIONS WITH ANSWER HINT
24 Videos
- ELECTRIC FIELDBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - NCERT EXEMPLAR QUESTIONS WITH ANSWER HINT
7 Videos
- ELECTRIC FIELDBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - SECTION RELATION QUESTIONS
50 Videos
- ELECTRIC ENERGY AND POWERBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC ENERGY AND POWEREXERCISE - CBSE SCANNER
7 Videos
- ELECTRIC POTENTIAL BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC POTENTIAL EXERCISE - CBSE Scanner
13 Videos
Similar Questions
A point particle of mass M is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particles carry charges +q and −q respectively. This arrangement is held in a region of a uniform electric field E such that the rod makes a small angle θ (say of about 5 degree) with the field direction, fig. Find an expression for the minimum time needed for the rod to become parrallel to the field after it is set free.
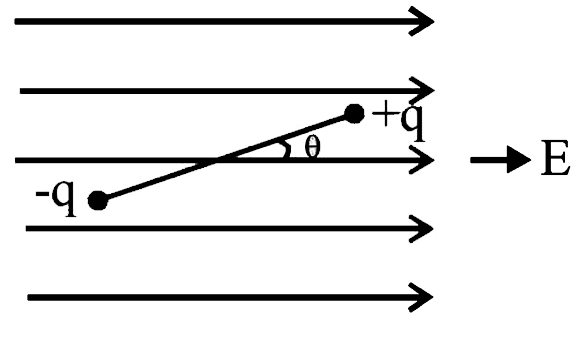
View SolutionA point particle of mass M is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particles carry charges +q and −q respectively. This arrangement is held in a region of a uniform electric field E such that the rod makes a small angle θ (say of about 5 degree) with the field direction, fig. Find an expression for the minimum time needed for the rod to become parrallel to the field after it is set free.

View Solution
Knowledge Check
A particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical circular path about its other end. The minimum speed of the particle at its highest point must be
AzeroB√glC√1.5glD√2glTwo particles of mass 5 kg and 10 kg respectively are attached to the twoends of a rigid rod of length 1 m with negligible mass. the centre of mass of the system from the 5 kg particle is nearly at a distance of :
A50 cmB67 cmC80 cmD33 cmA charged particle of charge q and mass m is shot into a uniform magnetic field of induction B at an angle θ with the field. The frequency of revolution of the particle
ADepends on the angle θBIs independent of the angle θCIs proportional to the specific charge q/m of the particleDIs inversely proportional to the value of B
A particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical ciorcular path about its other end. The minimum speed of the particle at its highest point must be
View SolutionTwo particles of mass 5 kg and 10 kg respectively are attached to the twoends of a rigid rod of length 1 m with negligible mass. the centre of mass of the system from the 5 kg particle is nearly at a distance of :
View SolutionTwo particles of mass 5 kg and 10 kg respectively are attached to the two ends of a rigid rod of length 1 m with negligible mass. the centre of mass of the system from the 5 kg particle is nearly at a distance of :
View SolutionA particle of mass m and charge q is attached to a light rod oflength L. The rod cau rotate freely in the plane of paper about the other end, which is hinged at P, the.entire assembly lies in an uniform electric field E acting in the plane of paper ·as shown in the figure-. The rod is released from rest when it makes an angle 9 with the electric field direction. Determine the speed oft he particle when the rod becomes parallel to the electric field:

View SolutionA light rigid rod of length l is hinged at one end and it is free to rotate in a vertical plane. A particle of mass m is attached to another end of the rod. The particle is released from rest at its highest point. Select the correct statement out of the following
View Solution
