A person is swimming with a speed of 10 m/s at an angle of 120° with the flow and reaches to a point directly opposite on the other side of the river. The speed of the flow is 'x m/s.
The value of 'x to the nearest integer is_________.
To solve the problem, we need to analyze the swimmer's motion relative to the river flow. The swimmer swims at an angle of 120° with respect to the flow of the river, and we need to find the speed of the river flow, denoted as 'x'.
1. Understanding the Angles:
- The swimmer swims at an angle of 120° with respect to the flow of the river.
- This means the angle between the swimmer's direction and the direction opposite to the river flow is 180°−120°=60°.
2. Components of the Swimmer's Velocity:
- The swimmer's speed is given as U=10m/s.
- We can break down the swimmer's velocity into two components:
- The component along the direction opposite to the river flow (which we will denote as Uy).
- The component perpendicular to the river flow (which we will denote as Ux).
- Using trigonometry:
- Uy=U⋅sin(60°)
- Ux=U⋅cos(60°)
3. Calculating the Components:
- We know:
- sin(60°)=√32
- cos(60°)=12
- Thus:
- Uy=10⋅sin(60°)=10⋅√32=5√3m/s
- Ux=10⋅cos(60°)=10⋅12=5m/s
4. Condition for Reaching the Opposite Bank:
- For the swimmer to reach the point directly opposite on the other side of the river, the downstream velocity of the river (denoted as x) must equal the component of the swimmer's velocity in the direction of the river flow:
- x=Uy
- Therefore, we have:
- x=5√3m/s
5. Calculating the Value of 'x':
- To find the nearest integer value of x:
- 5√3≈5⋅1.732≈8.66
- Rounding to the nearest integer gives:
- x≈9m/s
Final Answer:
The value of 'x' to the nearest integer is 9.
- The swimmer swims at an angle of 120° with respect to the flow of the river.
- This means the angle between the swimmer's direction and the direction opposite to the river flow is 180°−120°=60°.
- The swimmer's speed is given as U=10m/s.
- We can break down the swimmer's velocity into two components:
- The component along the direction opposite to the river flow (which we will denote as Uy).
- The component perpendicular to the river flow (which we will denote as Ux).
- Using trigonometry:
- Uy=U⋅sin(60°)
- Ux=U⋅cos(60°)
- We know:
- sin(60°)=√32
- cos(60°)=12
- Thus:
- Uy=10⋅sin(60°)=10⋅√32=5√3m/s
- Ux=10⋅cos(60°)=10⋅12=5m/s
- For the swimmer to reach the point directly opposite on the other side of the river, the downstream velocity of the river (denoted as x) must equal the component of the swimmer's velocity in the direction of the river flow:
- x=Uy
- Therefore, we have:
- x=5√3m/s
- To find the nearest integer value of x:
- 5√3≈5⋅1.732≈8.66
- Rounding to the nearest integer gives:
- x≈9m/s
The value of 'x' to the nearest integer is 9.
Topper's Solved these Questions
Similar Questions
A man can swim at a speed 2ms−1 in still water. He starts swimming in a river at an angle 150∘ with direction of flow of water and reaches at the exactly oppsite point on the opposite bank.
(a) Find the speed of flowing water.
(b) If width of river is 1 km then calculate the time taken alsoView Solution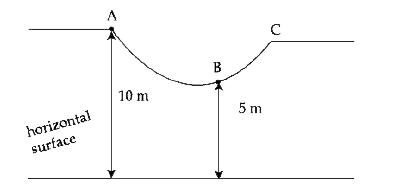
As shown in the figure, a particle of mass 10 kg is placed at a point A. When the particle is slightly displaced to its right, it starts moving and reaches the point B. The speed of the particle at B is x m/s. (Take g=10m/s2 ) The value of 'x to the nearest integer isView Solution
Knowledge Check
- Question 1 - Select One
A person, reaches a point directly opposite on the other bank of a flowing river, while swimming at a speed of 5 m/s at an angle of 120∘ with the flow. The speed of the flow must be
A2.5 m/sB3 m/sC4 m/sD1.5 m/s - Question 2 - Select One
A swimmer crosses a river of width d flowing at velocity v. While swimming, he keeps himself always at an angle of 120∘ with the river flow and on reaching the other end. He finds a drift of d/2 in the direction of flow of river. The speed of the swimmer with respect to the river is :
A(2−√3)vB2(2−√3)vC4(2−√3)vD(2+√3)v - Question 3 - Select One
A boat man could row his boat with a speed 10 m/sec. He wants to take his boat from P to a point Q just opposite on the other bank of the river flowing at a speed 4 m/sec. He should row his boat–
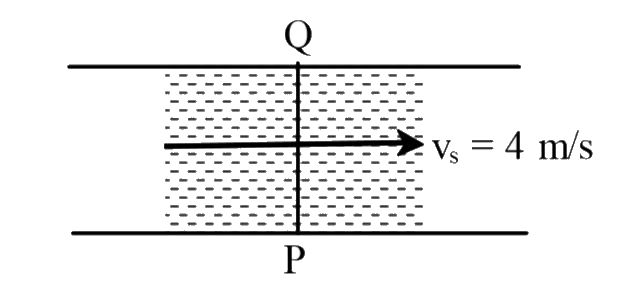 Aat right angle to the streamBat an angle of sin−1(2/5) with PQ up the streamCat an angle of sin−1(2/5) with PQ down the streamDat an angle cos−1(2/5) with PQ down the stream
Aat right angle to the streamBat an angle of sin−1(2/5) with PQ up the streamCat an angle of sin−1(2/5) with PQ down the streamDat an angle cos−1(2/5) with PQ down the stream
A swimmer swims with a speed of 10ms at angle of 1200 from direction of river flow. Find velocity of river flow such that swimmer reach exactly opposite point of bank.
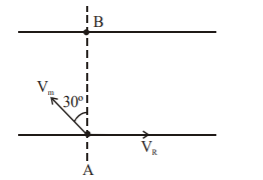 View Solution
View SolutionA person aiming to reach exactly opposite point on the bank of a stream is swimming with a speed of 0.6 m/s at an angle of 120∘ with the direction of flow of water . The speed of water in the stream is
View SolutionA boat man could row his boat with a speed 10 m/sec. He wants to take his boat from P to a point Q just opposite on the other bank of the river flowing at a speed 4 m/sec. He should row his boat–
 View Solution
View SolutionA person aiming to reach the exactly opposite point on the bank of a stream is swimming with a speed of 0.5ms at an angle of 120∘ with the direction of flow of water. The speed of water in the stream is
View SolutionA river of width 80m is flowing at 6 m/s. A motorboat crosses the river in 10s and reaches a point directly across on the other river bank. The velocity of motorboat in still water is :
View Solution

