A piece of wire is bent in the shape of a parabola (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
a/gk
a/2gk
2a/gk
a/4gk
The correct Answer is:B
To solve the problem, we need to find the new equilibrium position of the bead on the parabolic wire when the wire is accelerated parallel to the x-axis with a constant acceleration a.
1. Understand the Setup:
The wire is shaped like a parabola given by the equation y=kx2. Initially, when the wire is at rest, the bead is at the lowest point of the parabola (at the origin, (0,0)).
2. Acceleration of the Wire:
When the wire is accelerated with a constant acceleration a in the positive x-direction, the bead will move to a new position along the wire.
3. Free Body Diagram:
At the new position, we can analyze the forces acting on the bead:
- The weight of the bead mg acts downward.
- The normal force N acts perpendicular to the surface of the wire.
- A pseudo force Fpseudo=ma acts on the bead in the opposite direction of the acceleration of the wire (to the left).
4. Determine Forces:
Let θ be the angle that the tangent to the parabola makes with the horizontal at the new position of the bead. The components of the normal force can be resolved as:
- Nsinθ=ma (horizontal direction)
- Ncosθ=mg (vertical direction)
5. Relate Forces:
From the equations above, we can derive:
tanθ=ag
6. Slope of the Parabola:
The slope of the parabola at any point is given by the derivative:
dydx=2kx
Therefore, at the new position, we can also express tanθ as:
tanθ=2kx
7. Equate the Two Expressions for tanθ:
Setting the two expressions for tanθ equal gives:
2kx=ag
8. Solve for x:
Rearranging the equation to solve for x:
x=a2kg
9. Conclusion:
The distance of the new equilibrium position of the bead from the y-axis is:
x=a2kg
Final Answer:
The distance of the new equilibrium position of the bead from the y-axis is a2kg.
The wire is shaped like a parabola given by the equation y=kx2. Initially, when the wire is at rest, the bead is at the lowest point of the parabola (at the origin, (0,0)).
When the wire is accelerated with a constant acceleration a in the positive x-direction, the bead will move to a new position along the wire.
At the new position, we can analyze the forces acting on the bead:
- The weight of the bead mg acts downward.
- The normal force N acts perpendicular to the surface of the wire.
- A pseudo force Fpseudo=ma acts on the bead in the opposite direction of the acceleration of the wire (to the left).
Let θ be the angle that the tangent to the parabola makes with the horizontal at the new position of the bead. The components of the normal force can be resolved as:
- Nsinθ=ma (horizontal direction)
- Ncosθ=mg (vertical direction)
From the equations above, we can derive:
tanθ=ag
The slope of the parabola at any point is given by the derivative:
dydx=2kx
Therefore, at the new position, we can also express tanθ as:
tanθ=2kx
Setting the two expressions for tanθ equal gives:
2kx=ag
Rearranging the equation to solve for x:
x=a2kg
The distance of the new equilibrium position of the bead from the y-axis is:
x=a2kg
The distance of the new equilibrium position of the bead from the y-axis is a2kg.
Topper's Solved these Questions
- LAWS OF MOTIONBook:NCERT FINGERTPS ENGLISHChapter:LAWS OF MOTIONExercise:Exemplar Problems
Explore 9 Videos
- LAWS OF MOTIONBook:NCERT FINGERTPS ENGLISHChapter:LAWS OF MOTIONExercise:Assertion & Reason
Explore 15 Videos
- LAWS OF MOTIONBook:NCERT FINGERTPS ENGLISHChapter:LAWS OF MOTIONExercise:Assertion And Reason
Explore 15 Videos
- KINETIC THEORYBook:NCERT FINGERTPS ENGLISHChapter:KINETIC THEORYExercise:Assertion And Reason
Explore 10 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:NCERT FINGERTPS ENGLISHChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT Exemplar
Explore 5 Videos
Similar Questions
A piece of wire is bent in the shape of a parabola y=kx2 (y axis vertical) with a bead of mass mon it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated paralle to the x-axis with a constant acceleration a. The distance of the the new equilibrium position of the bead. Find the x-coordinate where the bead can stay at rest with respect to the wire ?
View SolutionA straight wire carrying current is parallel to the y-axis as shown in Fig. The
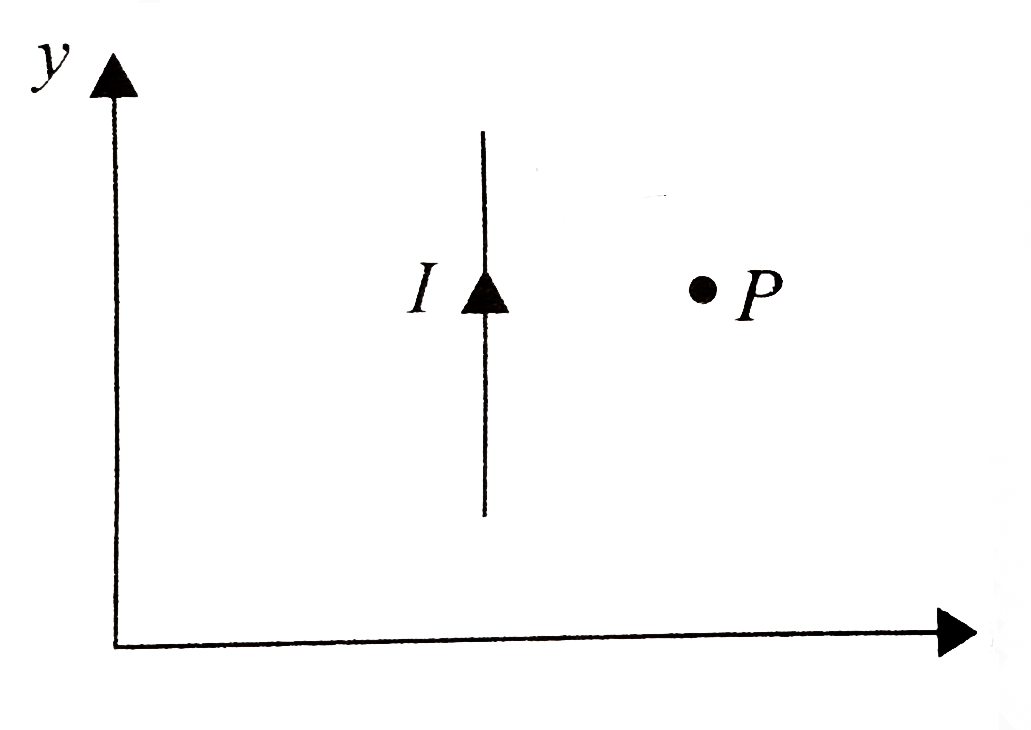
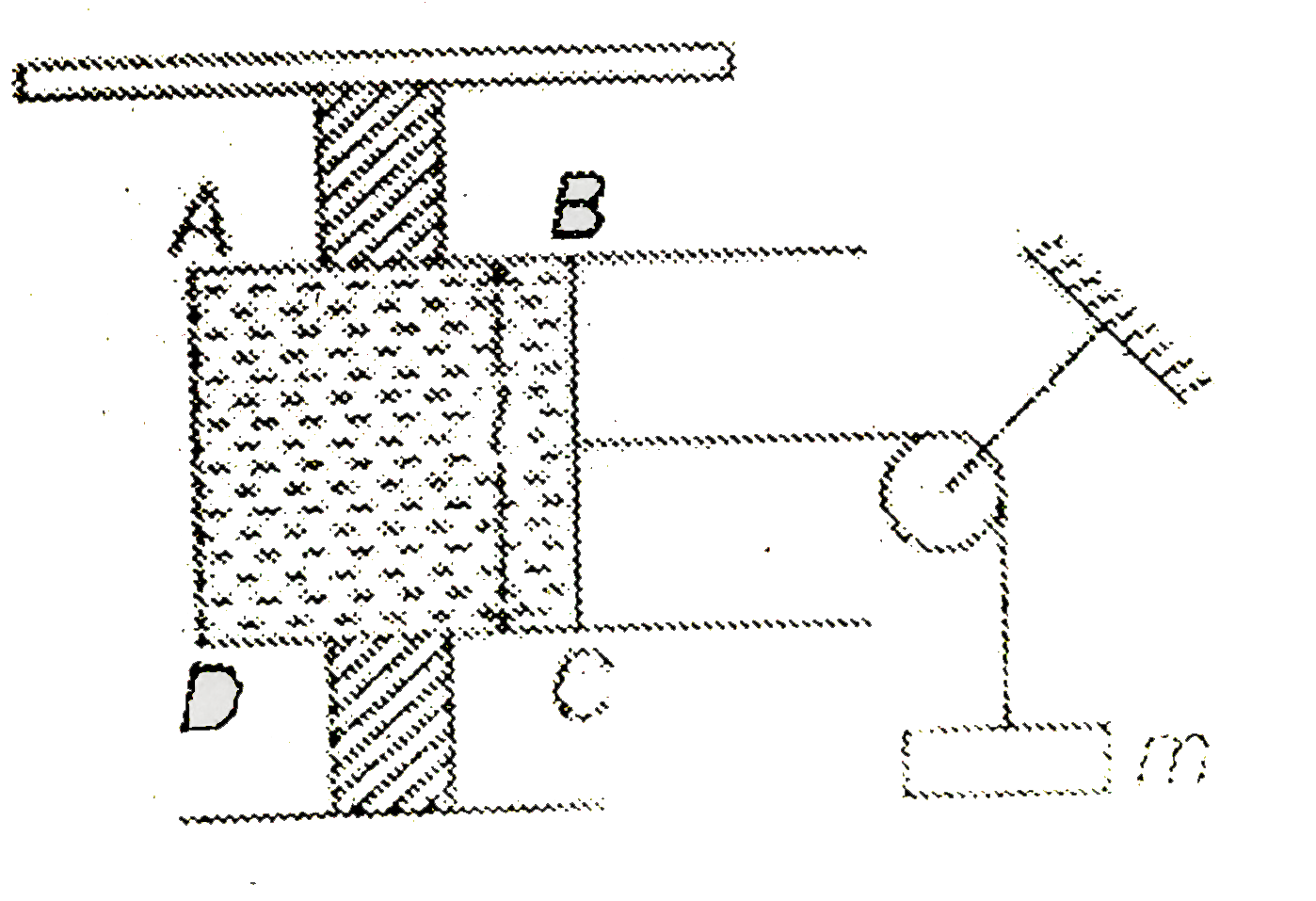
View SolutionA wire frame ABCD has a soap film. The wire BC can slide on the frame without friction and it is in equilibrium in the position shown in the figure. Find m, if T is the surface tension of the liquid.
View SolutionA bead of mass m is fitted on a rod and can move on it without friction. Initially the bead is at the middle of the rod moves transitionally in the vertical plane with an accleration a0 in direction forming angle α with the rod as shown. The acceleration of bead with respect to rod is:
z
View SolutionA thick uniform wire is bent into the shape of the letter “U” as shown. Which point indicates the location of the center of mass of this wire?
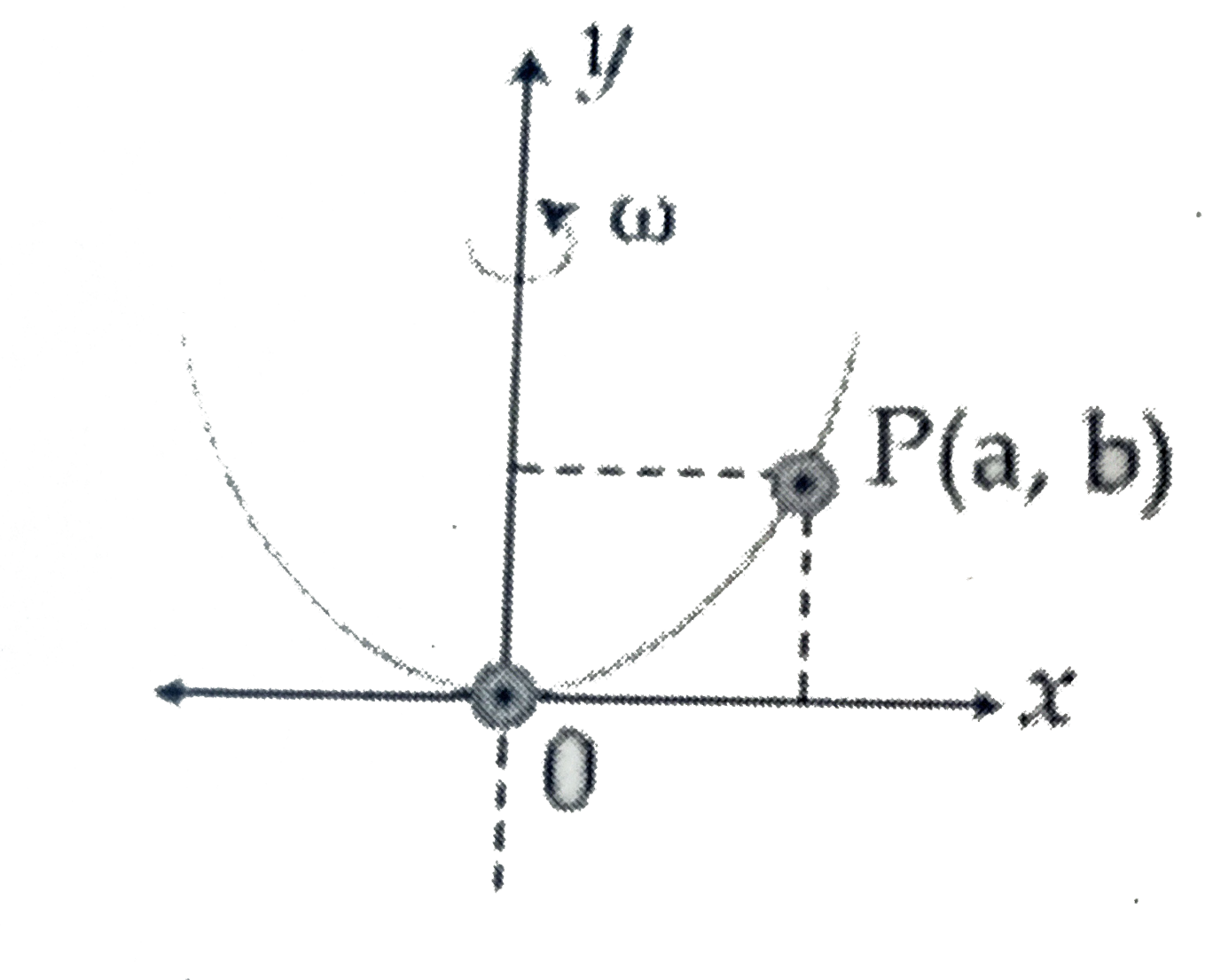
View SolutionA bead of mass m stays at point P (a,b) on a wire bent in the shape of a parabola y=4Cx2 and rotating with angular speed ω (see figure ) . The value of ω is (neglect friction),
View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is

View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is

View SolutionAngular velocity of smooth parabolic wire y = 4c(x)^(2) about axis of parabola in vertical plane if bead of mass m does not slip at (a,b) will be
View SolutionA smooth wire of length 2πr is bent into a circle and kept in a vertical plane. A bead can slide smoothly on the wire. When the circle is rotating with angular speed about the vertical diameter AB, as shown in the figure, the bead is at rest with respect to the circular ring at potion P as shown. Then the value of ω2 is equal to:
View Solution
