A projectile's launch speed is 6.00 times that of its speed at its maximum height. Find the launch angle θ0.
To solve the problem, we need to find the launch angle θ0 given that the launch speed u is 6 times the speed at maximum height.
1. Understand the Components of the Launch Speed:
The launch speed u can be broken down into horizontal and vertical components:
- Horizontal component: ux=ucosθ0
- Vertical component: uy=usinθ0
2. Determine the Speed at Maximum Height:
At maximum height, the vertical component of the velocity becomes zero, and only the horizontal component remains:
- Speed at maximum height vmax=ux=ucosθ0
3. Set Up the Relationship:
According to the problem, the launch speed u is 6 times the speed at maximum height:
u=6vmax
Substituting the expression for vmax:
u=6(ucosθ0)
4. Simplify the Equation:
Dividing both sides by u (assuming u≠0):
1=6cosθ0
5. Solve for cosθ0:
Rearranging gives:
cosθ0=16
6. Find the Launch Angle θ0:
To find θ0, take the inverse cosine:
θ0=cos−1(16)
7. Calculate the Angle:
Using a scientific calculator:
θ0≈80.41∘
Final Answer:
The launch angle θ0 is approximately 80.41∘.
The launch speed u can be broken down into horizontal and vertical components:
- Horizontal component: ux=ucosθ0
- Vertical component: uy=usinθ0
At maximum height, the vertical component of the velocity becomes zero, and only the horizontal component remains:
- Speed at maximum height vmax=ux=ucosθ0
According to the problem, the launch speed u is 6 times the speed at maximum height:
u=6vmax
Substituting the expression for vmax:
u=6(ucosθ0)
Dividing both sides by u (assuming u≠0):
1=6cosθ0
Rearranging gives:
cosθ0=16
To find θ0, take the inverse cosine:
θ0=cos−1(16)
Using a scientific calculator:
θ0≈80.41∘
The launch angle θ0 is approximately 80.41∘.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)49videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)3videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - CHECKPOINT3videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Integer Type )3videos
MOTION ALONG A STRAIGHT LINE
- BOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Practice Questions57videos
OSCILLATIONS
Similar Questions
A body is thrown at an angle θ0 with the horizontal such that it attains a speed equal to (√23 times the speed of projection when the body is at half of its maximum height. Find the angle θ0.
View Solution" For a projectile the ratio of the square of the time of flight to its maximum height is "
View Solution
Knowledge Check
- Question 1 - Select One
The speed of a projectile when it is at its greatest height is √2/5 times its speed at half the maximum height. The angle of projection is
A30∘B60∘C45∘Dtan−1(3/4) - Question 2 - Select One
The launching speed of a certain projectile is five times the speed it has at its maximum height.Its angles of projection is
Aθ=cos−1(0.2)Bθ=sin−1(0.2)Cθ=tan−1(0.2)Dθ=0∘ - Question 3 - Select One
A projectile is launched from the surface of the earth with a very high speed u at an angle θ with vertical . What is its velocity when it is at the farthest distance from the earth surface . Given that the maximum height reached when it is launched vertically from the earth with a velocity v = √GMR
Aucosθ2Businθ2C√GM2RD√GM3R
Find the average velocilty of a projectile between the instants it crosses half the maximum height. It is projected with speed u ast angle θ with the horizontal.
View SolutionIf the range of a projectile is x times its maximum height attained and also its speed at maximum height is 1/2 times its speed of projection, then the value of x is
View SolutionTwo projectiles are launched from a building of height 'h' as shown in the figure. One is launched at angle θ above horizontal and the other at angle θ below horizontal. Both the projectiles have same initial speed u. Which of the following is /are correct?
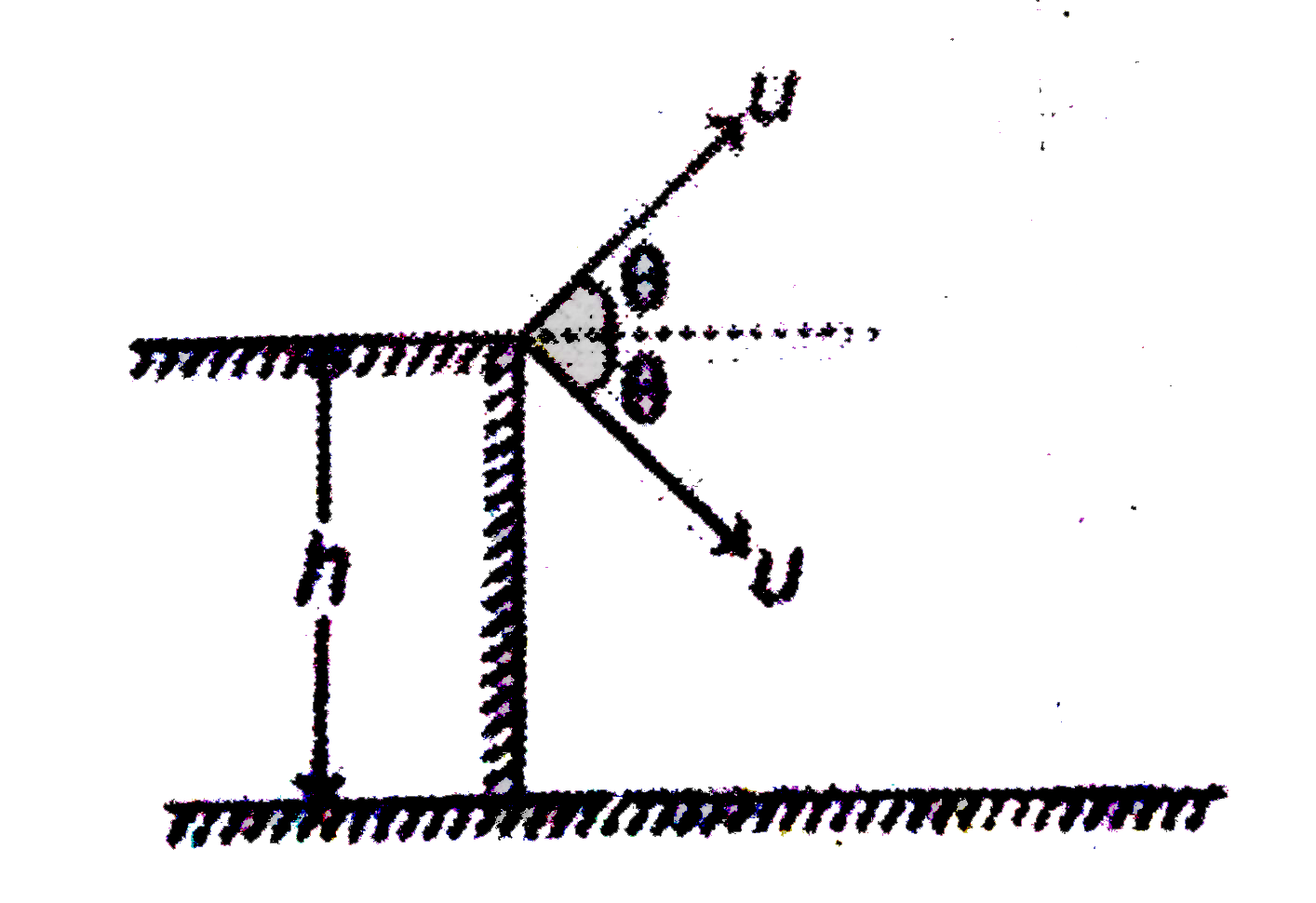 View Solution
View SolutionFor two projectiles launched with same initial velocity, the maximum heights corresponding to equal ranges are 4m and 16m. The range has a value
View SolutionThe total speed of a projectile at its greater height is √67 of its speed when it is at half of its greatest height. The angle of projection will be :-
View Solution

