A resistance of 2Ω is connected across one gap of a meter bridge (the length of the wire is 100cm) and an unknown resistance, greater than 2Ωis conneted across the other gap. When these resistances are interchanged, the balance point shifts by 20cm. Neglecting any corrections,the unknown resistance is
3Ω
4Ω
5Ω
6Ω
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Understand the Meter Bridge Setup
A meter bridge consists of a wire of length 100 cm, and we have a known resistance (2Ω) connected across one gap and an unknown resistance (R) connected across the other gap. The balance point is where the galvanometer shows zero current.
Step 2: Set Up the Balance Condition
When the resistances are in their initial positions, the balance condition can be expressed as:
2X=R100−X
where X is the initial balance length.
Step 3: Rearranging the Equation
From the balance condition, we can rearrange it to find R:
2(100−X)=R⋅X
R=2(100−X)X
Step 4: Analyze the Shift in Balance Point
When the resistances are interchanged, the balance point shifts by 20 cm. The new balance point is X+20. The new balance condition is:
RX+20=280−X
where 80−X is the new length on the opposite side.
Step 5: Rearranging the New Balance Condition
From the new balance condition, we can rearrange it:
R(80−X)=2(X+20)
Step 6: Substitute R from Step 3 into Step 5
Substituting the expression for R from Step 3 into the new balance condition:
2(100−X)X(80−X)=2(X+20)
Step 7: Simplifying the Equation
Cancelling 2 from both sides:
(100−X)(80−X)X=X+20
Cross-multiplying gives:
(100−X)(80−X)=X(X+20)
Step 8: Expanding Both Sides
Expanding both sides:
8000−100X−80X+X2=X2+20X
This simplifies to:
8000−180X=20X
Step 9: Solving for X
Combining like terms:
8000=200X
X=8000200=40 cm
Step 10: Finding the Unknown Resistance R
Now substituting X=40 cm back into the equation for R:
R=2(100−40)40=2×6040=12040=3Ω
Final Answer
The unknown resistance R is 3Ω.
---
A meter bridge consists of a wire of length 100 cm, and we have a known resistance (2Ω) connected across one gap and an unknown resistance (R) connected across the other gap. The balance point is where the galvanometer shows zero current.
When the resistances are in their initial positions, the balance condition can be expressed as:
2X=R100−X
where X is the initial balance length.
From the balance condition, we can rearrange it to find R:
2(100−X)=R⋅X
R=2(100−X)X
When the resistances are interchanged, the balance point shifts by 20 cm. The new balance point is X+20. The new balance condition is:
RX+20=280−X
where 80−X is the new length on the opposite side.
From the new balance condition, we can rearrange it:
R(80−X)=2(X+20)
Substituting the expression for R from Step 3 into the new balance condition:
2(100−X)X(80−X)=2(X+20)
Cancelling 2 from both sides:
(100−X)(80−X)X=X+20
Cross-multiplying gives:
(100−X)(80−X)=X(X+20)
Expanding both sides:
8000−100X−80X+X2=X2+20X
This simplifies to:
8000−180X=20X
Combining like terms:
8000=200X
X=8000200=40 cm
Now substituting X=40 cm back into the equation for R:
R=2(100−40)40=2×6040=12040=3Ω
The unknown resistance R is 3Ω.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise 23.12
3 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise 23.13
2 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise 23.10
2 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
A resistance of 2Ω is connected across one gap of a meter bridge (the length of the wire is 100cm and an unknown resistance, greater than 2Ω, is connected across the other gap. When these resistance are interchanged, the balance point shifts by 20cm. Neglecting any corrections, the unknown resistance is.
(a)3Ω
(b) 4Ω
( c) 5Ω
(d) 6Ω.
View SolutionResistance in the two gaps of a meter bridge are 10ohm and 30ohm respectively. If the resistances are interchanged he balance point shifts by
View Solution
Knowledge Check
Two resistances are connected in the two gaps of a meter bridge. The balance point is 20cm from the zero end. When a resistance 15Ω is connected in series with the smaller of two resistance, the null point+ shifts to 40cm. The smaller of the two resistance has the value.
A3B6C9D12
In the metre bridge, the balancing length AB = 20 cm. The unknown resistance X is equal to
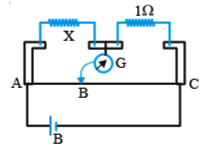
View SolutionAn unknown resistance R1 is connected is series with a resistance of 10Ω. This combination is connected to one gap of a meter bridge, while other gap is connected to another resistance R2. The balance point is at 50cm Now , when the 10Ω resistance is removed, the balanced point shifts to 40cm Then the value of R1 is.
View SolutionAn unknown resistance R1 is connected is series with a resistance of 10Ω. This combination is connected to one gap of a meter bridge, while other gap is connected to another resistance R2. The balance point is at 50cm Now , when the 10Ω resistance is removed, the balanced point shifts to 40cm Then the value of R1 is.
View SolutionIn a metre bridge, the length of the wire is 100 cm. At what position will the balance point be obtained if the two resistances are in the ratio 2 : 3?
View SolutionIn the metre bridge, the balancing length AB =l=20 cm. The unknown resistance X is equal to
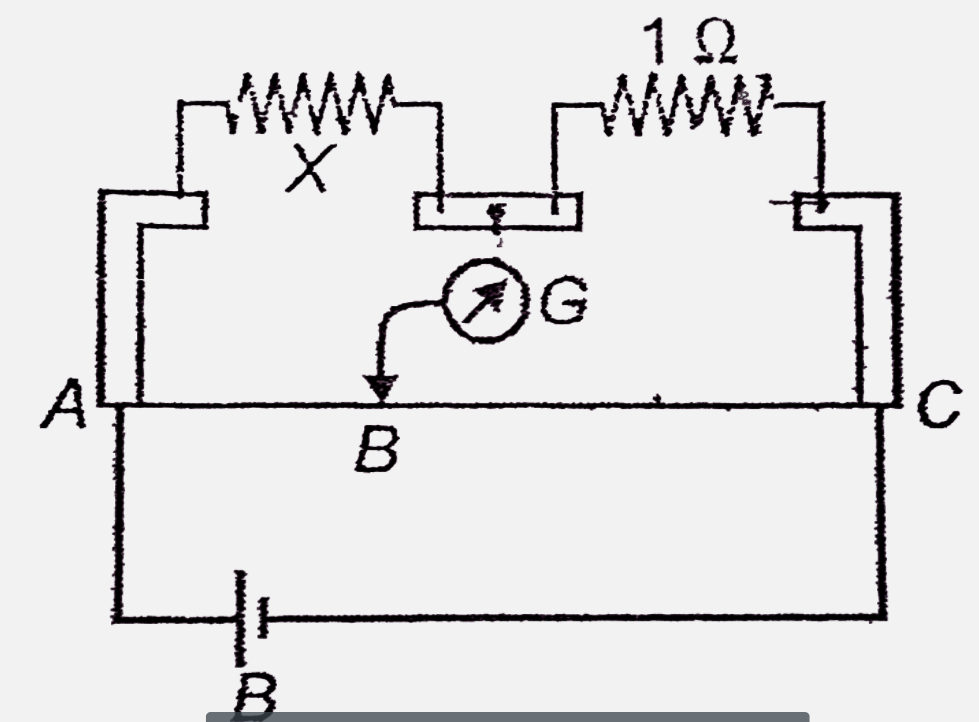
View SolutionTwo resistances are connected in the two gaps of a meter bridge. The balance point is 20cm from the zero end. When a resistance 15Ω is connected in series with the smaller of two resistance, the null point+ shifts to 40cm. The smaller of the two resistance has the value.
View SolutionTwo resistances are connected in the two gaps of a meter bridge. The balance point is 20cm from the zero end. When a resistance 15Ω is connected in series with the smaller of two resistance, the null point+ shifts to 40cm. The smaller of the two resistance has the value.
View Solution
