A satellite is in circular orbit about Earth at an altitude of 300 km. What are its (a) orbital period, (b) orbital fre quency, and (c) linear speed?
To solve the problem, we will calculate the orbital period, orbital frequency, and linear speed of a satellite in a circular orbit at an altitude of 300 km above the Earth's surface.
Given Data:
- Altitude of the satellite, h=300km=300×103m
- Radius of the Earth, RE=6.38×106m
- Gravitational constant, G=6.67×10−11m3/kg⋅s2
- Mass of the Earth, ME=5.98×1024kg
Step 1: Calculate the distance from the center of the Earth to the satellite
The total distance r from the center of the Earth to the satellite is given by:
r=RE+h=6.38×106m+300×103m=6.68×106m
Step 2: Calculate the orbital period T
Using Kepler's third law, the formula for the orbital period T is:
T=2π√r3GME
Substituting the values:
T=2π√(6.68×106)36.67×10−11×5.98×1024
Calculating the above expression:
1. Calculate r3:
r3=(6.68×106)3≈2.99×1020m3
2. Calculate GME:
GME=6.67×10−11×5.98×1024≈3.986×1014m3/s2
3. Now calculate T:
T=2π√2.99×10203.986×1014≈5421.36s
Converting seconds to minutes:
T≈5421.3660≈90.35minutes
Step 3: Calculate the orbital frequency f
The orbital frequency f is the reciprocal of the orbital period:
f=1T=15421.36≈1.845×10−4s−1
Step 4: Calculate the linear speed v
The linear speed v of the satellite is given by:
v=2πrT
Substituting the values:
v=2π(6.68×106)5421.36≈7738.07m/s
Converting to kilometers per second:
v≈7.738km/s
Final Answers:
(a) Orbital Period T≈90.35minutes
(b) Orbital Frequency f≈1.845×10−4s−1
(c) Linear Speed v≈7.738km/s
- Altitude of the satellite, h=300km=300×103m
- Radius of the Earth, RE=6.38×106m
- Gravitational constant, G=6.67×10−11m3/kg⋅s2
- Mass of the Earth, ME=5.98×1024kg
The total distance r from the center of the Earth to the satellite is given by:
r=RE+h=6.38×106m+300×103m=6.68×106m
Using Kepler's third law, the formula for the orbital period T is:
T=2π√r3GME
Substituting the values:
T=2π√(6.68×106)36.67×10−11×5.98×1024
Calculating the above expression:
1. Calculate r3:
r3=(6.68×106)3≈2.99×1020m3
2. Calculate GME:
GME=6.67×10−11×5.98×1024≈3.986×1014m3/s2
3. Now calculate T:
T=2π√2.99×10203.986×1014≈5421.36s
Converting seconds to minutes:
T≈5421.3660≈90.35minutes
The orbital frequency f is the reciprocal of the orbital period:
f=1T=15421.36≈1.845×10−4s−1
The linear speed v of the satellite is given by:
v=2πrT
Substituting the values:
v=2π(6.68×106)5421.36≈7738.07m/s
Converting to kilometers per second:
v≈7.738km/s
(a) Orbital Period T≈90.35minutes
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)16videos
GRAVITATION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)7videos
GRAVITATION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - CHECKPOINT5videos
GRAVITATION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(Integers)4videos
GEOMETRICAL OPTICS : REFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - HEAT-MEASUREMENT AND TRANSFEREXERCISE - PRACTICE QUESTIONS( INTEGER TYPE)5videos
HEAT-MEASUREMENT AND TRANSFER
Similar Questions
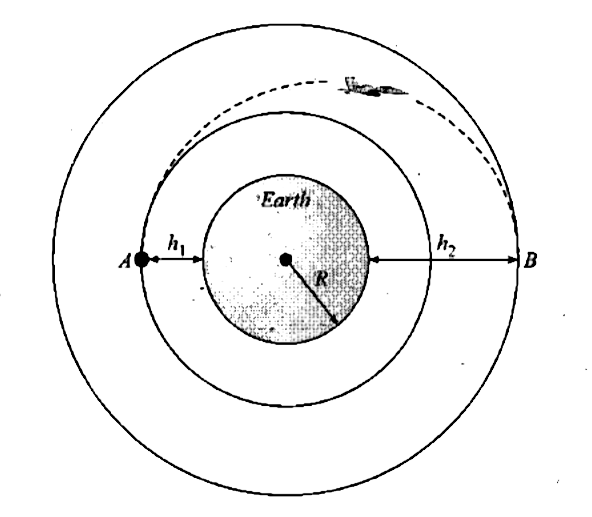
The optimal way of transferring a space vehicle from an inner circular orbit to an outer coplanar circular orbit is to fire its engines as it passes through A to increase its speed and place it in an elliptic transfer orbit. Another increase in speed as it passes through B will place it in the desired circular orbit. For a vehicle in a circular orbit about the earth at an altitude h1=320km, which is to be transferred to a circular orbit at an altitude h2=800km, determine :
(a) The required increases in speed at A and B.
(b) The total energy per unit mass required to execute the
 View Solution
View SolutionA satellite is put in a circular orbit about Earth with a radius equal to two-thirds the radius of the Moon's orbit. What is its period of revolution in lunar months? (A lunar month is the period of revolution of the Moon)
View Solution
Knowledge Check
- Question 1 - Select One
A satellite of mass is in a stable circular orbit around the earth at an altitude of about 100 km. If M is the mass of the earth, R its radius and g the acceleration due to gravity, then the time period T of the revolution of the satellite is
AT=2π√RgBT=2π√gRCT=2π√MRmgDT=2π√mgMg - Question 2 - Select One
A satellite is orbiting the earth in a circular orbit of radius r. Its
Akinetic energy veries as rBangular momentum varies as r−1Clinear momentum varies as r2Dfrequency of revolution varies as r−3/2 - Question 3 - Select One
A satellite is placed in a circular orbit about Earth with radius equal to half the radius of the moon· s orbit. Period of rotation of the satellite is n times that of the lunar month (lunar month is the period of revolution of the. moon). The value of n is
A2−3/2B23/2C21/2D2−1/2
A remote sensing satellite of the Earth in a circular orbit at a height of 400km above the surface of Earth. What is the (a) orbital speed, and (b) period of revolution of satellite ? Radius of Earth =6×106m and acceleration due to gravity the surface of Earth is 10m/s2.
View SolutionTwo Earth satellites, A and B, each of mass m, are to be launched into circular orbits about Earth's center. Satellite A is to orbit at an altitude of 6370 km. Satellite B is to orbit at an altitude of 19 850 km. The radius of Earth R, is 6370 km. (a) What is the ratio of the potential energy of satellite B tu trial of satellite A, in orbit? (b) What is the ratio of the kinetic energy of satellite R to that of satellite A, in orbit? (C) Which satellite has the greater total energy if each has a mass of 14.6 kg? (d) By how much?
View SolutionA remote sensing satellite of the earth revolves in a circular orbit at a height of 250 km above the earth's surface. What is the (i) orbital speed and (ii) period of revolution of the satellite ? Radius of the earth, R=6.38×106 m, and acceleration due to gravity on the surface of the earth, g=9.8ms−2.
View SolutionA satellite is in a circular orbit round the earth at an altitude R above the earth's surface, where R is the radius of the earth. If g is the acceleration due to gravity on the surface of the earth, the speed of the satellite is
View SolutionA satellite is revolving round the earth in circular orbit
View Solution

