A small sleeve of mass m=1/10kg can move along the diameter of a horizontal disc, which slides without friction along a guide rode. The sleeve is tied to the end of the rod with the aid of a massless spring whose force constant k=10N/m. When the spring is not under tension, the sleeve is at the oscillations of the sleeve when the disc rotates about its axis at the angular speed ω equal to a) 6 rad/s, b) 15 rad/s.
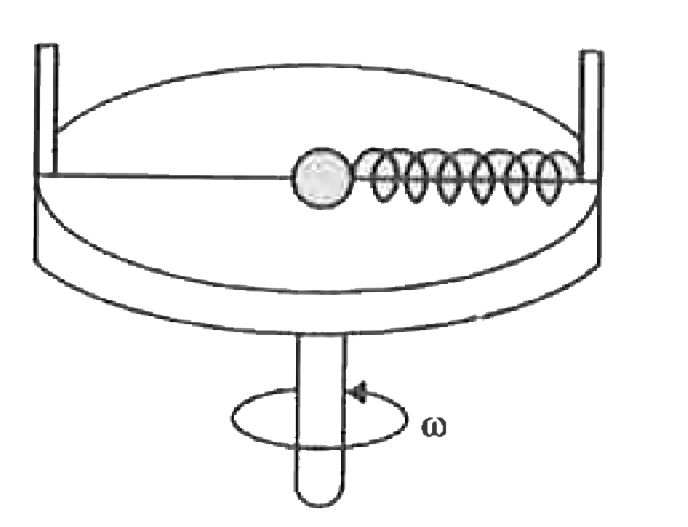
The correct Answer is:v=1.27s−1,(b) v=0, the sleeve does not oscillate
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:NN GHOSHChapter:SIMPLE HARMONIC MOTIONExercise:Eexercies
Explore 39 Videos
- SIMPLE AND COMPOUND PENDULUMBook:NN GHOSHChapter:SIMPLE AND COMPOUND PENDULUMExercise:Exercise
Explore 24 Videos
- SPECIFIC HEAT CAPACITY OF GASESBook:NN GHOSHChapter:SPECIFIC HEAT CAPACITY OF GASESExercise:All Questions
Explore 17 Videos
Similar Questions
What type of the friction arises when an axle rotates in sleeve ?
View SolutionA light rod AB is fitted with a small sleeve of mass m which can slide smoothly over it. The sleeve is connected to the two ends of the rod using two springs of force constant 2k and k (see fig). The ends of the springs at A and B are fixed and the other ends (connected to sleeve) can move along with the sleeve. The natural length of spring connected to A is l0. Now the rod is rotated with angular velocity w about an axis passing through end A that is perpendicular to the rod. Take kmω2=η and express the change in length of each spring (in equilibrium position of the sleeve relative to the rod) in terms of l0 and η.
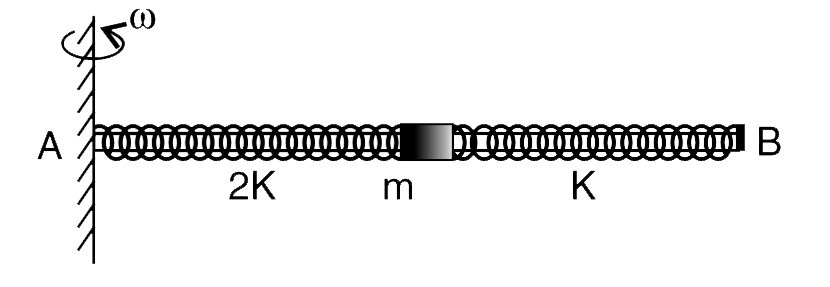
View Solution
Knowledge Check
to have something up one's sleeves
Ahaving a practical planBhaving an important projectChaving an ambitious planDhaving a secret planA 1.2kg mass is oscillating without friction on a spring whose spring constant is 3400N/m. When the mass's displacement is 7.2cm. What is its acceleration?
A−3.8m/s2B−200m/s2C−240m/s2D−2.0×104m/s2A rod of mass 'M' and length'
L' is hinged about one end and a
particle of mass 'M' is
attached to its other end If it is
kept horizontal with the help of
a spring of spring constant 'K'
as shown in fig. find the
extension in spring :-
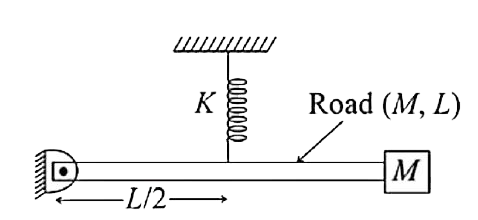 A3MgKBMgKC2MgKD4MgK
A3MgKBMgKC2MgKD4MgK
A wheel is mounted on frictionless central pivot and it can rotate freely in the vertical plane. There is a horizontal light rod fixed to the wheel below the pivot. There is a small sleeve of mass m which can slide along the rod without friction. The sleeve is connected to a light spring. The other end of the spring is fixed to the rim as shown. The sleeve is at the centre of the rod and the spring is relaxed. Now the wheel is held at rest and the sleeve is moved towards left so as to compress the sprig by some distance. The sleeve and the wheel are released simultaneously from this position.
(a) Is it possible that the wheel does not rotate as the sleeve perform SHM on the rod ?
(b) Find the value of spring constant k for situation described in (a) to be possible. The distance of rod from centre of the wheel is d.
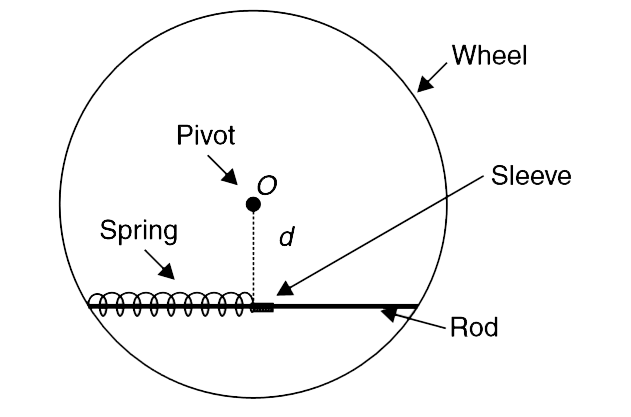
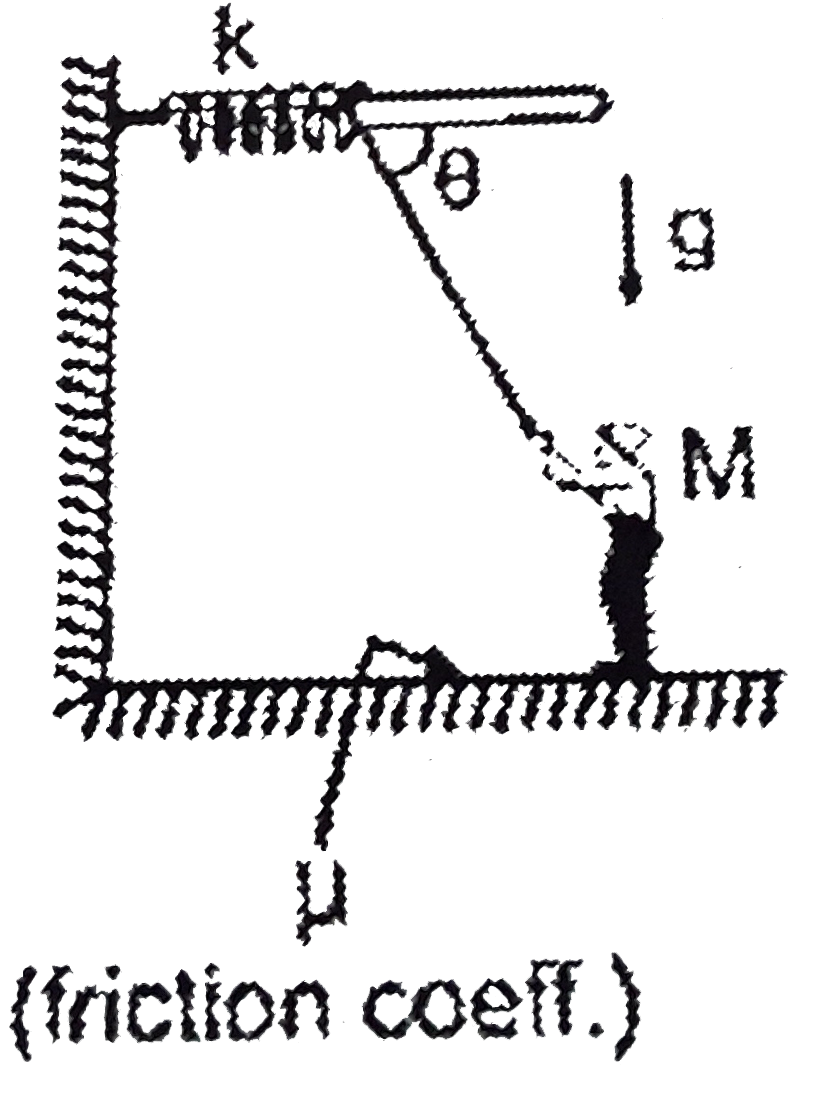
View SolutionA dog with mass M has its string attached to one end of a spring which runs without friction along a horizontal overhead rod. The other end of the springs is fixed to a wall The spring constant is K. The string is massless and inextensible and it maintains a constant angle θ with the overhead rod, even when the dog moves. There is friction with coefficient mu between the dog and the gound What is the maximum distance (in cm) that the dog moving slowly can stretch the spring beyond its natural length? Use M=30 kg,θ=400N/mandμ=13
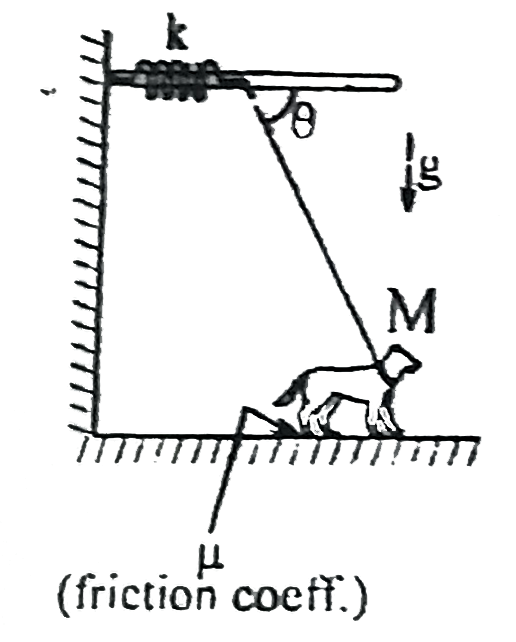
View SolutionA man with mass M has its string'.attached to one end. of a spring which can move without friction along a horizontal, overhead fixed rod. The other end of the spring is fixed to a, wall. The spring constant is k. The string is massless and inextensible and it maintains a constant angle θ with the, overhead rod, even when the man moves. There is friction, iwith coefficient μ between the man and the ground. What is, the maximum distance (in m ) that the man moving slowly can stretch the spring beyond its natural length?
View SolutionA uniform disc of mass m is attached to a spring of spring constant k as shown in figure and there is sufficient friction to prevent slipping of disc. Time period of small oscillations of disc is:
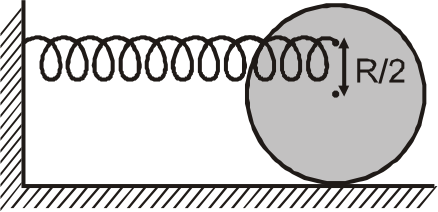
View SolutionA uniform disc of mass m is attached to a spring of spring constant k as shown in figure and there is sufficient friction to prevent slipping of disc. Time period of small oscillations of disc is:

View Solution
