A solenoid is placed inside another solenoid, the length of both being equal carrying same magnitude of current. The parameters like radius and number of turns are in the ratio 1:2 for the two solenoids. The mutual inductance on each other would be
M12=M21
M12=2M21
2M12=M21
M12=4M21
The correct Answer is:A
To find the mutual inductance between two solenoids placed inside each other, we can follow these steps:
Step 1: Understand the parameters
We have two solenoids:
- Solenoid 1 (inner):
- Radius r1
- Number of turns n1
- Length L
- Solenoid 2 (outer):
- Radius r2
- Number of turns n2
- Length L
Given:
- The radius ratio r1r2=12
- The number of turns ratio n1n2=12
Step 2: Write the formula for mutual inductance
The mutual inductance M between two solenoids can be expressed as:
M=μ0⋅n1⋅n2⋅πr21L
where μ0 is the permeability of free space.
Step 3: Substitute the ratios into the formula
Since n2=2n1 and r2=2r1, we can substitute these into the formula for mutual inductance:
M12=μ0⋅n1⋅(2n1)⋅πr21L
This simplifies to:
M12=μ0⋅2n21⋅πr21L
Step 4: Calculate mutual inductance for the second solenoid
Now, we can calculate the mutual inductance from the perspective of solenoid 2 on solenoid 1:
M21=μ0⋅(2n1)⋅n2⋅π(r22)L
Substituting n2=2n1 and r2=2r1:
M21=μ0⋅(2n1)⋅(2n1)⋅π(2r1)2L
This simplifies to:
M21=μ0⋅8n21⋅πr21L
Step 5: Compare mutual inductance
From the above calculations, we can see that:
- M12=μ0⋅2n21⋅πr21L
- M21=μ0⋅8n21⋅πr21L
However, since the mutual inductance is defined as the same for both solenoids, we conclude that:
M12=M21
Thus, the mutual inductance on each other would be equal.
Conclusion
The mutual inductance between the two solenoids is the same, and the final answer is:
M12=M21
We have two solenoids:
- Solenoid 1 (inner):
- Radius r1
- Number of turns n1
- Length L
- Solenoid 2 (outer):
- Radius r2
- Number of turns n2
- Length L
- The radius ratio r1r2=12
- The number of turns ratio n1n2=12
The mutual inductance M between two solenoids can be expressed as:
M=μ0⋅n1⋅n2⋅πr21L
where μ0 is the permeability of free space.
Since n2=2n1 and r2=2r1, we can substitute these into the formula for mutual inductance:
M12=μ0⋅n1⋅(2n1)⋅πr21L
This simplifies to:
M12=μ0⋅2n21⋅πr21L
Now, we can calculate the mutual inductance from the perspective of solenoid 2 on solenoid 1:
M21=μ0⋅(2n1)⋅n2⋅π(r22)L
Substituting n2=2n1 and r2=2r1:
M21=μ0⋅(2n1)⋅(2n1)⋅π(2r1)2L
This simplifies to:
M21=μ0⋅8n21⋅πr21L
From the above calculations, we can see that:
- M12=μ0⋅2n21⋅πr21L
- M21=μ0⋅8n21⋅πr21L
M12=M21
Thus, the mutual inductance on each other would be equal.
The mutual inductance between the two solenoids is the same, and the final answer is:
M12=M21
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together119videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrance special format questions17videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Example71videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
A solenoid S1 is placed inside another solenoid S2 as shown in the fig. The radii of the inner and the outer solenoids are r1 and r2 respectively and the numbers of turns per unit length are n1 and n2 respectively. Consider a length I of each solenoid. Calculate the mutual inductance between them.
 View Solution
View SolutionA tightly- wound, long solenoid is kept with its axis parallel to a large metal sheet carrying a surface current. The surgace current through a width dl of the sheet is Kdl and the number of turns per unit length of the solenoid is n. The magnetic field near the centre of the solenoid is found to be zero. (a) find the current in the solenoid. (b) If the solenoid is rotated to make its axis perpendicular to the metal sheet, what would be the magnitude of the magnetic field near its centre?
View Solution
Knowledge Check
- Question 1 - Select One
Two solenoids of equal number of turns have their lengths and the radii in the same ratio 1:2. The ratio of their self inductances will be
A1:2B2:1C1:1D1:4 - Question 2 - Select One
A short soleniod of radius a, number of turns per unit length n1, and length L is kept coaxially inside a very long solenoid of radius b, number of turns per unit length n2. What is the mutual inductance of the system?
Aμ0πb2n1n2LBμ0πa2n1n2L2Cμ0πa2n1n2LDμ0πb2n1n2L2
A short solenoid of length 4 cm, radius 2 cm and 100 turns is placed inside and on the axis of a long solenoid of length 80 cm and 1500 turns. A current of 3 A flows through the short solenoid. The mutual inductance of two solenoid is
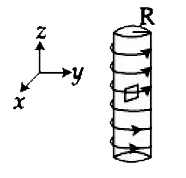
View SolutionAn electron gun is placed inside a long solenoid of radius R on its exis. The solenoid has n turns/Length and carries a current I. The electron gun schoots an electron along the radius of the solenoid with speed v.If the electron does not hit the surface of the solenoid, maximum possible value of v is (all symbols have their standard meaning):
 View Solution
View SolutionDefine mutual inductance between two long coaxial solenoids. Find out the expression for the mutual of inner solenoid of length 1 having the radius r1 and the number of turns n1 per unit length due to the second other solenoid of same length and n2 number of turns per unit length.
View SolutionA straight solenoid of length 1m has 5000 turns in the primary and 200 turns in the secondary. If the area of cross section is 4cm2, the mutual inductance will be
View SolutionWhen the number of turns and the length of the solenoid are doubled keeping the area of cross-section same, the inductance
View SolutionThe expression for magnetic induction inside a solenoid of length L carrying a current I and having N number of turns is
View Solution

