A spring of spring constant K is cut equal parts of which t part are p

A spring of spring constant k is cut into n equal parts, out of which r parts are placed in parallel and connected with mass M as shown in figure. The time period of oscillatory motion of mass M is:
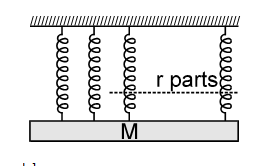A $T = 2 π \sqrt{\frac{M}{r n K}}$
B $T = 2 π \sqrt{\frac{n r M}{K}}$
C $T = 2 π \sqrt{\frac{n M}{n K}}$
D $T = 2 π \sqrt{\frac{n M}{r K}}$
A spring of spring constant k is cut in three equal pieces. The spring constant of each part will be
A $\frac{k}{3}$
B $3 k$
Ck
D $\frac{k}{6}$
Two springs of force constant k and 2k are connected to a mass shown in figure the frequency of oscillation of the mass is
A $\frac{1}{2 π} \sqrt{\frac{m}{k}}$
B $\frac{1}{2 π} \sqrt{2 \frac{k}{m}}$
C $\frac{1}{2 π} \sqrt{3 \frac{k}{m}}$
D $\frac{1}{2 π} \sqrt{\frac{m}{k}}$

A spring of spring constant $K$ is cut equal parts of which t part are places in particle and connected will mass $m$ shown in figure The time period of oscilation motion of mass $m$ is