A straight conductor 0.1 m long moves in a uniform magnetic field 0.1 T. The velocity of the conductor is 15ms−1 and is directed perpendicular to the field. The emf induced between the two ends of the conductor is
0.10 V
0.15 V
1.50 V
15 V
The correct Answer is:B
To find the induced electromotive force (emf) in a straight conductor moving in a magnetic field, we can use the formula:
emf(E)=L⋅v⋅B⋅sin(θ)
Where:
- E is the induced emf,
- L is the length of the conductor,
- v is the velocity of the conductor,
- B is the magnetic field strength,
- θ is the angle between the velocity vector and the magnetic field vector.
1. Identify the given values:
- Length of the conductor, L=0.1m
- Magnetic field strength, B=0.1T
- Velocity of the conductor, v=15m/s
- Angle θ=90∘ (since the conductor moves perpendicular to the magnetic field)
2. Calculate sin(θ):
- Since θ=90∘, we have:
sin(90∘)=1
3. Substitute the values into the emf formula:
E=L⋅v⋅B⋅sin(θ)
E=0.1m⋅15m/s⋅0.1T⋅1
4. Perform the multiplication:
E=0.1⋅15⋅0.1=0.15V
5. Conclusion:
The induced emf between the two ends of the conductor is 0.15V.
Final Answer:
The emf induced between the two ends of the conductor is 0.15 volts.
- E is the induced emf,
- L is the length of the conductor,
- v is the velocity of the conductor,
- B is the magnetic field strength,
- θ is the angle between the velocity vector and the magnetic field vector.
- Length of the conductor, L=0.1m
- Magnetic field strength, B=0.1T
- Velocity of the conductor, v=15m/s
- Angle θ=90∘ (since the conductor moves perpendicular to the magnetic field)
- Since θ=90∘, we have:
sin(90∘)=1
E=L⋅v⋅B⋅sin(θ)
E=0.1m⋅15m/s⋅0.1T⋅1
E=0.1⋅15⋅0.1=0.15V
The induced emf between the two ends of the conductor is 0.15V.
The emf induced between the two ends of the conductor is 0.15 volts.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Match the columns5videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
A straight wire of length L is bent into a semicircle. It is moved in a uniform magnetic field with speed v with diameter perpendicular to the field. The induced emf between the ends of the wire is
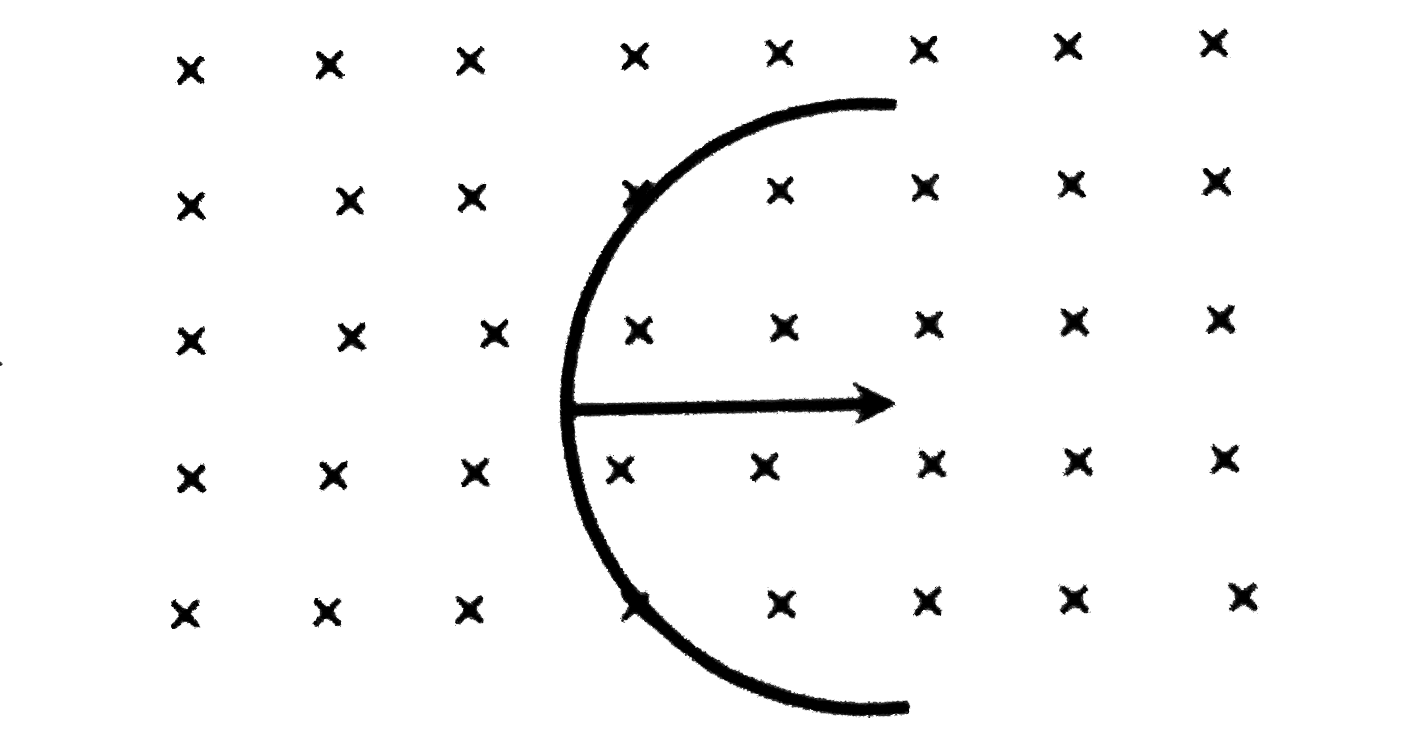 View Solution
View SolutionA copper disc of radius 0.1 m is rotated about its natural axis with 10 rps in a uniform magnetic field of 0.1 T with its plane perpendicular to the field, The emf induced across the radius of the disc is
View SolutionA rectangular conductor LMNO is place in a uniform magnetic field of 0.5 T Fig. The field is directed perpendicular to the plane to the conductor. When the arm MN of length 20 cm is moved with a velocity of 10 m/s calculate the emf induced in the arm. Find the value of induced current if resistance of arm MN is 5 Ohm
 View Solution
View SolutionA copper disc of radius 0.1 m is roated about its centre with 20 revolutions per second in a uniform magnetic field of 0.1 T with its plane perpendicular the field. The emf induced across the radius of disc is
View SolutionA conducting rod of unit length moves with a velocity of 5m/s in a direction perpendicular to its length and perpendicular to a uniform magnetic field of magnitude 0.4T. Find the emf induced between the ends of the stick.
View SolutionA metal rod of length 15×10−2m rotates about an axis passing through one end with a uniform angular velocity of 60 rad s−1. A uniform magnetic field of 0.1 Tesla exisits in the direction of the axis of rotationn. Calculate the EMF induced between the ends of the rod.
View SolutionThe conductor AD moves to the right in a magnetic field directed into the paper. Then
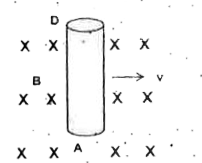 View Solution
View SolutionA metal rod of length 2 m is rotating with an angualr velocity of 100rads−1 in plane perpendicular to a uniform magnetic field of 0.3 T. The potential difference between the ends of the rod is
View SolutionA rectangular coil of area A rotates in a uniform magnetic field B with angular velocity about an axis perpendicular to the field, initially the plane of coil is perpendicular to the field,then the avarage induced e.m.f. after rotating by 90° is
View SolutionA conductor of length 0.1m is moving with a velocity of 4m/ s in a uniform magnetic field of 2T as shown in the figure. Find the emf induced ?
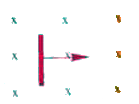 View Solution
View Solution

