A straight , long wire carries a current a current of 20A. Another wire carrying equal current is placed parallel to it. If the force acting on a length of 10cm of the second wire is , what is the separation between them?
To solve the problem, we need to find the separation between two parallel wires carrying equal currents. We can use the formula for the magnetic force between two parallel wires.
1. Identify the Given Values:
- Current in each wire, I=20A
- Length of the second wire, L=10cm=0.1m
- Force acting on the second wire, F=2.0×10−5N
2. Use the Formula for Magnetic Force:
The magnetic force F on a length L of a wire carrying current I in a magnetic field B is given by:
F=I⋅L⋅B
where B is the magnetic field created by the first wire at the location of the second wire.
3. Calculate the Magnetic Field B:
The magnetic field B at a distance d from a long straight wire carrying current I1 is given by:
B=μ0I12πd
where μ0=4π×10−7T m/A is the permeability of free space.
4. Substitute B into the Force Equation:
Substituting the expression for B into the force equation:
F=I⋅L⋅μ0I12πd
Here, I1=I=20A.
5. Rearranging the Equation:
Rearranging the equation to solve for d:
d=μ0I2L2πF
6. Substituting the Known Values:
Now substitute the known values into the equation:
d=(4π×10−7)⋅(20)2⋅(0.1)2π⋅(2.0×10−5)
7. Calculating the Separation d:
Simplifying this expression:
d=(4⋅202⋅0.1)2⋅(2.0×10−5)
d=(4⋅400⋅0.1)4×10−5=1604×10−5=40×102=0.4m
8. Final Result:
Thus, the separation between the two wires is:
d=0.4mor40cm
- Current in each wire, I=20A
- Length of the second wire, L=10cm=0.1m
- Force acting on the second wire, F=2.0×10−5N
The magnetic force F on a length L of a wire carrying current I in a magnetic field B is given by:
F=I⋅L⋅B
where B is the magnetic field created by the first wire at the location of the second wire.
The magnetic field B at a distance d from a long straight wire carrying current I1 is given by:
B=μ0I12πd
where μ0=4π×10−7T m/A is the permeability of free space.
Substituting the expression for B into the force equation:
F=I⋅L⋅μ0I12πd
Here, I1=I=20A.
Rearranging the equation to solve for d:
d=μ0I2L2πF
Now substitute the known values into the equation:
d=(4π×10−7)⋅(20)2⋅(0.1)2π⋅(2.0×10−5)
Simplifying this expression:
d=(4⋅202⋅0.1)2⋅(2.0×10−5)
d=(4⋅400⋅0.1)4×10−5=1604×10−5=40×102=0.4m
Thus, the separation between the two wires is:
d=0.4mor40cm
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - questions for short answer12videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 27videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELDEXERCISE - objective29videos
MAGNETIC FIELD
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Short Answer6videos
MAGNETIC PROPERTIES OF MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
Two long and parallel straight wires A and B are carrying currents of 4A and 7A in the same directions are separated by a distance of 5cm. The force acting on a 8cm section of wire A is
A3×10−6NB6×10−6NC9×10−6ND12×10−6N - Question 2 - Select One
If a long straight wire carries a current of 40 A, then the magnitude of the field B at a point 15 cm away from the wire is
A5.34×10−5TB8.34×10−5TC9.6×10−5TD10.2×10−5T
A long straight wire is carrying a current of 25 A. A squre loop of side length 10 cm carrying a current of 15 A, is placed 2 cm from the wire as shown in figure. The force on the loop is
 View Solution
View SolutionA straight wire carries a current of 10A. An electron moving at 103 m/s is at distance 2.0 cm from the wire. Find the force acting on the electron if its velocity is directed toward the wire?
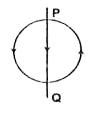
View SolutionIn the figure PQ is a long current carrying wire which is placed near a current carrying coil. The direction of the force acting on PQ will be
 View Solution
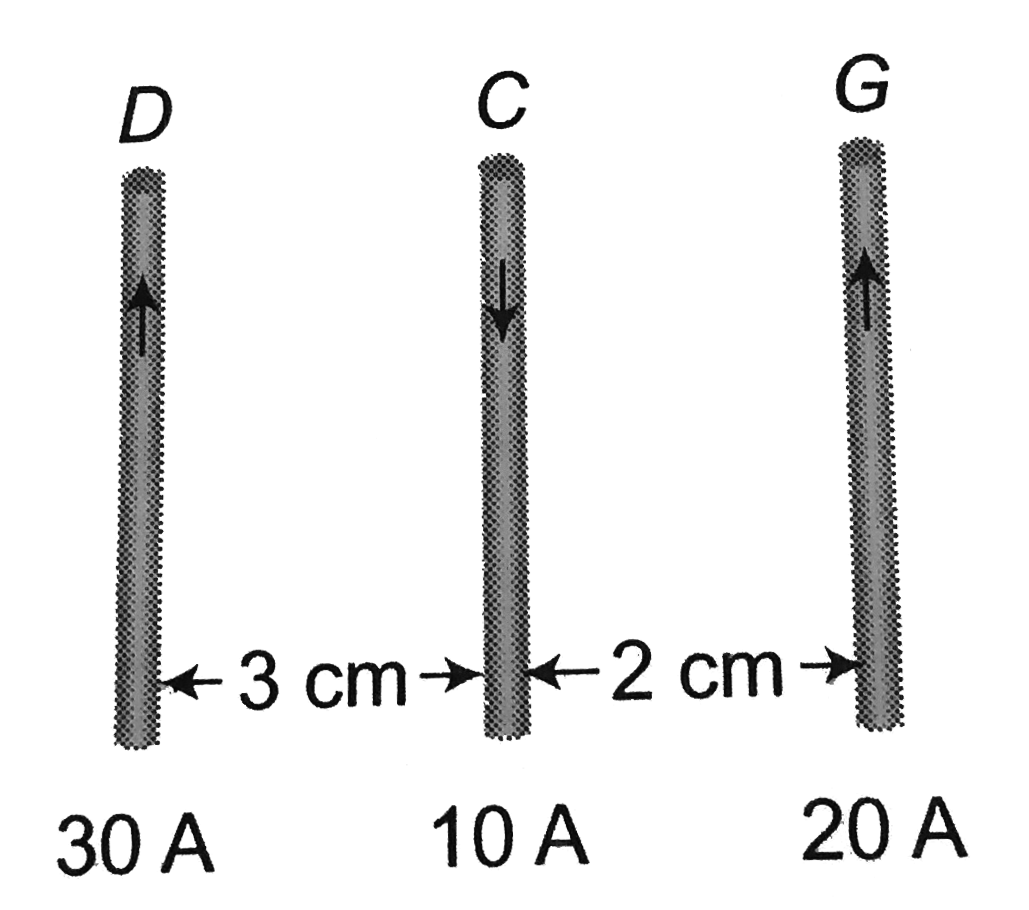
View SolutionThree long, straight parallel wires carrying current, are arranged as shown in figure. The force experienced by a 25cm length of wire C is
 View Solution
View SolutionA long straight wire carries a current of 35A. What is the magnitude of the field →B at a point 20cm from the wire?
View SolutionA negative test charge is moving mear a long straight wire carrying a current. The force acting on the test charge is parallel to the direction of the current. The motion of the charge is:
View Solution