A piece of wire carrying a current of 6.00 A is bent in the form of a circular arc of radius 10.0 cm, and it subtends an angle of 120∘ at the centre. Find the magnetic field B due to this piece of wire at the centre.
To find the magnetic field B at the center of a circular arc of wire carrying a current, we can use the formula:
B=μ0Iθ4πR
where:
- μ0 is the permeability of free space, approximately 4π×10−7T m/A
- I is the current in amperes
- θ is the angle in radians
- R is the radius in meters
Step 1: Convert the given values to appropriate units
1. Current I: Given as 6.00A.
2. Radius R: Given as 10.0cm, which we convert to meters:
R=10.0cm=10.0×10−2m=0.1m
3. Angle θ: Given as 120∘. We convert this to radians:
θ=120∘×π180∘=2π3radians
Step 2: Substitute the values into the formula
Now we can substitute the values into the formula for the magnetic field:
B=(4π×10−7)⋅(6.00)⋅(2π3)4π⋅(0.1)
Step 3: Simplify the expression
1. The 4π in the numerator and denominator cancels out:
B=(10−7)⋅(6.00)⋅(2π3)0.1
2. Simplifying further:
B=(10−7)⋅(6.00)⋅(2π)0.3
Step 4: Calculate the numerical value
1. Calculate 6.00⋅2=12.00.
2. Now, we can calculate:
B=(12.00⋅π)⋅(10−7)0.3
3. Using π≈3.14:
B≈(12.00⋅3.14)⋅(10−7)0.3=37.68⋅10−70.3≈125.6×10−7T
4. Converting to standard form:
B≈1.256×10−5T
Final Answer
The magnetic field B at the center of the arc is approximately:
B≈1.25×10−5T
- μ0 is the permeability of free space, approximately 4π×10−7T m/A
- I is the current in amperes
- θ is the angle in radians
- R is the radius in meters
1. Current I: Given as 6.00A.
2. Radius R: Given as 10.0cm, which we convert to meters:
R=10.0cm=10.0×10−2m=0.1m
3. Angle θ: Given as 120∘. We convert this to radians:
θ=120∘×π180∘=2π3radians
Now we can substitute the values into the formula for the magnetic field:
B=(4π×10−7)⋅(6.00)⋅(2π3)4π⋅(0.1)
1. The 4π in the numerator and denominator cancels out:
B=(10−7)⋅(6.00)⋅(2π3)0.1
2. Simplifying further:
B=(10−7)⋅(6.00)⋅(2π)0.3
1. Calculate 6.00⋅2=12.00.
2. Now, we can calculate:
B=(12.00⋅π)⋅(10−7)0.3
3. Using π≈3.14:
B≈(12.00⋅3.14)⋅(10−7)0.3=37.68⋅10−70.3≈125.6×10−7T
4. Converting to standard form:
B≈1.256×10−5T
The magnetic field B at the center of the arc is approximately:
B≈1.25×10−5T
Topper's Solved these Questions
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - questions for short answer
12 Videos
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 2
7 Videos
- MAGNETIC FIELDBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC FIELDEXERCISE - objective2
9 Videos
- MAGNETIC PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Short Answer
6 Videos
Similar Questions
In a circle of radius 21 cm, an arc subtends an angle of 60o at the centre. Find: (i) length of the arc
View SolutionFind the length of an arc of a circle of radius 10cm subtending an angle of 300 at the centre
View SolutionIn a circle of radius 35 cm, an arc subtends an angle of 72o at the centre. Find the length of the arc and area of the sector.
View SolutionFind the length of an arc of circle of radius 6cm subtending an angle of 15∘ at the centre.
View SolutionA straight wire carrying a current of 13 A is bent into a semi-circular arc of radius 2 cm as shown in figure. The magnetic field is 1.5×10−4T at the centre of arc, then the magnetic field due to straight segment is

View SolutionA piece of wire 20 cm long is bent into the form of an arc of a circle, subtending an angle of 60∘ at its centre. Find the radius of the circle.
View SolutionA wire is bent in the form of a circular arc of radius r with a straight portion AB. If the current in the wire is i, then the magnetic induction at point O is
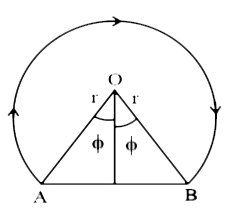
View SolutionAn arc of a circle of raduis R subtends an angle π2 at the centre. It carriers a current i. The magnetic field at the centre will be
View SolutionA current i ampere flows in a circular arc of wire whose radius is R, which subtend an angle 3π/2 radian at its centre. The magnetic induction B at the centre is

View SolutionA current i ampere flows in a circular arc of wire whose radius is R, which subtend an angle 3π/2 radian at its centre. The magnetic induction B at the centre is

View Solution
