A thin uniform disc of mass m and radius R suspended by an elastic thread in the horizontal plane performs torsional oscillations in a liquid. The moment of leastic forces emerging in the thread is equal to N=αφ, where α is a constant and φ is the angle of rotation from the equilibrium position. The resistance force acting on a unit area of the disc is equal to F1ηv, whereη is a constant and v is the velocity of the given element of the disc relative to the liquid. Find the frequency of small oscillation.
Let us calculate the moment G1 of all the resistive forces on the disc. When the disc rotates an element (rdrdθ) with coordinates (r,θ) has a velocity r.φ where φ is the intantaneious angle of rotation from the equilibrium position and r is measured from the centre. Then
G1=∫2π0dθ∫R0dr.r.(F1×r)
=∫R0ηr.φr2dγ×2π=ηπR42.φ
Also moment of inertia =mR22
Thus mR22..φ+πηR42.φ+αφ=0
or..φ+2πηR22m.φ+2αmR2φ=0
Hence ω20=2αmR2 and β=πηR22m
and angular frequency ω=√(2αmR2)−(πηR22m)2
Note :− normally by frequency we mean ω2π.
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electric Oscillations
56 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
If the elctron ( charge of each electron =−e) are shifted by a small distance x,a net +ve charge density ( per unit area ) is induced on the surface. This will result in an electric field E=nex/ε0 in the direction of x and a restoring force on an electron of
−ne2xε0,
Thus m..x=−ne2xε0
or ..x+ne2mε0x=0
This gives ωp=√ne2mε0=1.645×1016s−1
as the plasma frequency for the problem,.
View SolutionHow long will it take sound waves to travel a distance l between points A and B if the air temperature between them varies linearly from T1 and T2? (The velocity of sound in air at temperature T is given by v=α√T, where α is a constant)
View SolutionA person walks at a velocity v in a straight line forming an angle α with the plane of a mirror. Determine the velocity vrel which he approaches his image, assuming that the object and its image are symmetric relative to the plane of the mirror.
View SolutionA rod moves lengthwise with a constant velocity v relative to the inertial reference frame K. At what value of v will the length of the rod in this frame be η=0.5% less than its proper length?
View SolutionA uniform disc of mass m and radius r is suspended through a wire attached to its centre. If the time period of the torsional oscillations be T, what is the torsional constant of the wire?
View SolutionA uniform disc of mass m and radius R is rolling up a rough inclined plane which makes an angle of 30^@ with the horizontal. If the coefficients of static and kinetic friction are each equal to mu and the only force acting are gravitational and frictional, then the magnitude of the frictional force acting on the disc is and its direction is .(write up or down) the inclined plane.
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View SolutionThe ends of thin threads tightly wound on the axle of radius r of the Maxwell disc are attached to a horizontal bar. When the disc unwinds, the bar is raised to keep the disc at the same height. The mass of the disc with the axle is equal to m, the moment of inertia of the arrangement relative to its axis is I. Find the tension of each thread and the acceleration of the bar.
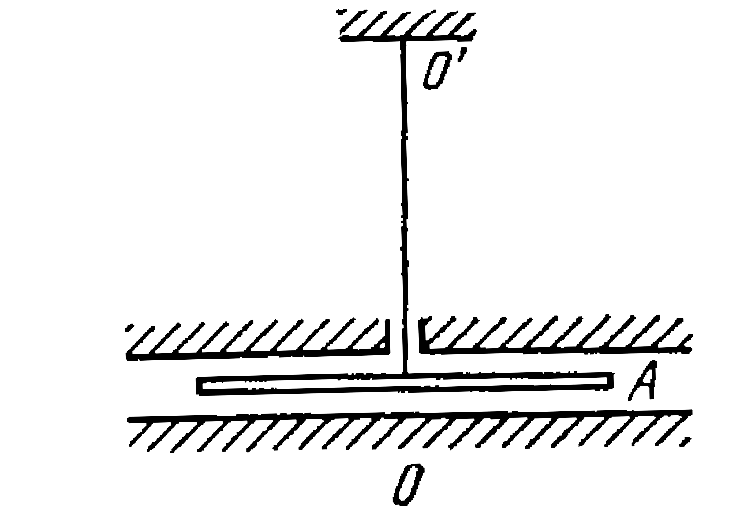
View SolutionA disc A of radius R suspended by an elastic threat between two stationary plane ( figure ) performs torsional oscillations about its axis OO′ . The moment of inertia of the disc relative to that axis is equal to I, the clearance between the disc and each of the planes is equal to h, with h<<R. Find the viscosity of the gas surrounding the disc A if the oscilaltion period of the disc equals T and the logarithmic damping decrement, λ.
View SolutionA uniform horizontal disc fixed at its centre to an elastic vertical rod performs forced torsional oscillations dur to the moment of forces Nz=Nmcosωt. The oscialltions obey the law φ=φmcos(ωt−α).
(a) the work performed by friction forces acting on the disc during one oscillation period ,
(b) the quality factor of the given oscillator if th emoment of inertia of the disc relative to the axis is equal to I.
View SolutionA force F acts tangentially on a part of a uniform disc of mass 'm' and radius 'R' hinged at point O. The force exerted by hinge on the portion of disc initially.
View SolutionTwo equal and opposite forces are allplied tangentially to a uniform disc of mass M and radius R as shown in the figure. If the disc is pivoted at its centre and free to rotate in its plane, the angular acceleration of the disc is :

View Solutionएक समरूप डिस्क के केंद्र से एक तार बाँधकर इसे किसी बिंदु से लटकाया गया है। डिस्क की त्रिज्या r तथा द्रव्यमान m है और यह क्षैतिज तल में रहती है। इसे मरोड़ी दोलन (torsional oscillations) देने पर इसका आवर्तकाल T होता है। तार का मरोड़ी स्थिरांक निकालें।
View SolutionThe moment of Inertia of a uniform semicircular disc of mass M and radius 'r' about a line perpendicular to the plane of the disc through the centre is :
View SolutionA uniform disc of mass m and radius r is suspended through a wire attached to its Centre. If the time period of the torsional oscillations be T, what is the torsional constant of the wire?
View SolutionA uniform disc of mass m and radius R is rolling up a rough inclined plane which makes an angle of 30∘ with the horizontal. If the coefficients of static and kinetic friction are each equal to μ and the only force acting are gravitational and frictional, then the magnitude of the frictional force acting on the disc is and its direction is .(write up or down) the inclined plane.
View Solution
