A thin wire ring of radius a carrying a charge q approcahes the observation point P so that its centre moves rectilinearly with a constant velocity v. The plane of the ring remians perpendicular to the motion direction. At what distance xm from the point P will the ring be located at the moment when the displacement current density at the point P becomes maximum? What is the magnitude of this maximum density ?
We have, EP=qx4πε0(a2+x2)3/2
then j4=∂D∂t=ε0∂E∂t=qv4π(a2+x2)5/2(a2−2x2)
This is maximum, when x=xm=0, and minimum at some other value. The maximum displacement current density is
(jd)max=qv4πa3
To check this we calculate ∂jd∂x,
∂j4∂x=qv4π[(−4x(a2+x2)−5x(a2−2x2)]
This vanishes for x=0 and for x=√32a. The latter is easily shown to be a smaller local minimum (negative maximum).
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field36videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics69videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions6videos
ELECTRICITY AND MAGNETISM
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions24videos
ELECTROMAGNETISM
Similar Questions
Knowledge Check
- Question 1 - Select One
A ring of radius R is charged uniformly with a charge +Q. The potential at a point on its axis at a distance 'r' from any point on ring will be-
AkQ√R2+r2BkQrCkQ(r2+R2)3/2DkQr2(r2−R2)1/2 - Question 2 - Select One or More
A ring rolls without slipping on the ground. Its centre C moves with a constant speed u.P is any point on the ring. The speed of P with respect to the ground is v.
A0≤v≤2uBv=u, if CP is horizontalCv=u, if CP makes an angle of 30∘ with the horizontal and P is below the horizontal level of C.Dv=√2u, if CP is horizontal. - Question 3 - Select One
A ring of radius R is rolling on a horizontal plane with constant velocity v. There is a constant and uniform magnetic field B which is perpendicular to the plane of the ring. Emf across the lowest point A and right-most point C as shown in the figure will
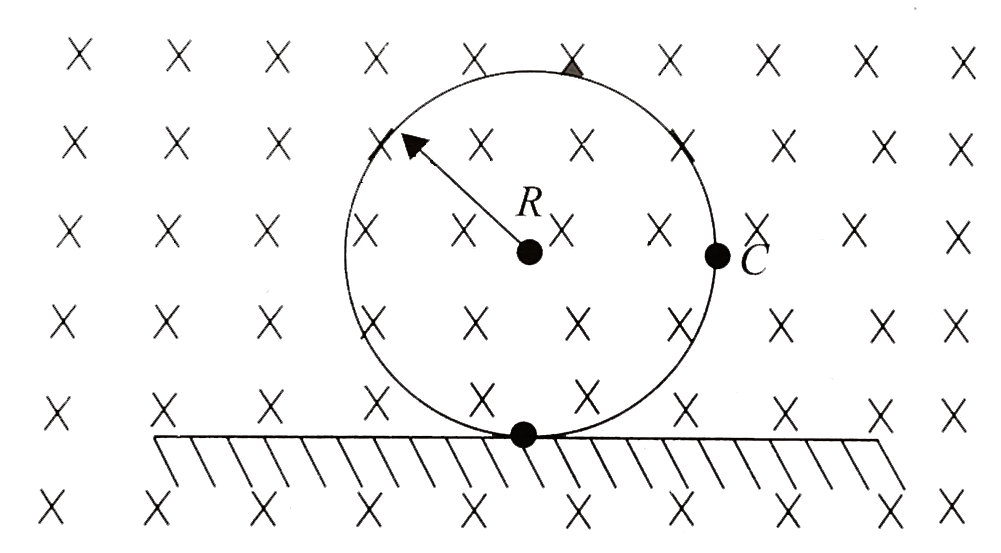 AIncrease with timeBdecrease with timeCremains constant and equal to √2BvRDremains constant and equal to BvR
AIncrease with timeBdecrease with timeCremains constant and equal to √2BvRDremains constant and equal to BvR
A thin wire ring of radius R carries a charge q. The ring si oriented parallel to an infinite conducting plane and is spearated by a distance l from it. Find :
(a) the surface chagre density at that point fo the plane symmetrical with respect to the ring,
(b) the strength and the potential of the electric field at the centre of the ring.View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .
 View Solution
View SolutionA thin circular wire of radius r has a charge Q. If a point charge q is placed at the centre of the ring, then find the increase in tension in the wire.
View SolutionThe mass of a circular ring is M and its radius is R. Its moment of inertia about an axis in the plane of ring at perpendicular distance R/2 from centre of ring is
View SolutionA thin conducting ring of radius R is given a charge +Q. The electric field at the centre O of the ring due to the charge on the part ABCD of the ring is
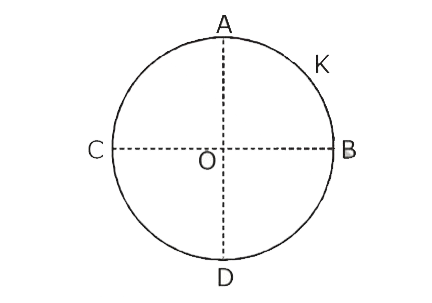 View Solution
View Solution

