A vertical capillary is brought in contact with the water surface (surface tension = T). The radius of the capillary is r and the contact angle θ=0∘. The increase in potential energy of the water (density =ρ) is
independent of ρ
independent of r
independent of T
zero
The correct Answer is:B
To solve the problem step by step, we will analyze the situation involving a vertical capillary tube in contact with water and calculate the increase in potential energy of the water.
Step 1: Understand the Given Parameters
- Surface tension of water, T
- Radius of the capillary tube, r
- Contact angle, θ=0∘
- Density of water, ρ
Step 2: Determine the Height of Water Rise in the Capillary
The height h to which water rises in a capillary tube can be calculated using the formula:
h=2Tcosθrρg
Since the contact angle θ=0∘, we have:
cos(0∘)=1
Thus, the formula simplifies to:
h=2Trρg
Step 3: Calculate the Volume of Water in the Capillary
The volume V of water that has risen in the capillary tube can be expressed as:
V=A⋅h
where A is the cross-sectional area of the capillary tube. The area A for a circular cross-section is given by:
A=πr2
Therefore, the volume becomes:
V=πr2⋅h=πr2⋅2Trρg=2πrTρg
Step 4: Calculate the Mass of Water
The mass m of the water that has risen in the capillary can be calculated using the density:
m=ρV=ρ⋅2πrTρg=2πrTg
Step 5: Calculate the Increase in Potential Energy
The increase in potential energy PE of the water is given by:
PE=mgh
Substituting the values of m and h:
PE=(2πrTg)⋅g⋅(2Trρg)
This simplifies to:
PE=2πrTg⋅g⋅2Trρg=4πT2ρg
Conclusion
The increase in potential energy of the water in the capillary tube is:
PE=4πT2ρg
- Surface tension of water, T
- Radius of the capillary tube, r
- Contact angle, θ=0∘
- Density of water, ρ
The height h to which water rises in a capillary tube can be calculated using the formula:
h=2Tcosθrρg
Since the contact angle θ=0∘, we have:
cos(0∘)=1
Thus, the formula simplifies to:
h=2Trρg
The volume V of water that has risen in the capillary tube can be expressed as:
V=A⋅h
where A is the cross-sectional area of the capillary tube. The area A for a circular cross-section is given by:
A=πr2
Therefore, the volume becomes:
V=πr2⋅h=πr2⋅2Trρg=2πrTρg
The mass m of the water that has risen in the capillary can be calculated using the density:
m=ρV=ρ⋅2πrTρg=2πrTg
The increase in potential energy PE of the water is given by:
PE=mgh
Substituting the values of m and h:
PE=(2πrTg)⋅g⋅(2Trρg)
This simplifies to:
PE=2πrTg⋅g⋅2Trρg=4πT2ρg
The increase in potential energy of the water in the capillary tube is:
PE=4πT2ρg
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Comprehension
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
Assertion :The contact angle between water and glass is acute.
Reason :The surface of water in the capillary is convex .
View SolutionA capillary tube of radius r is lowered into a liquid of surface tension T and density ρ. Given angle of contact =0∘.
What is the potential energy acquired by the liquid in the capillary?
View Solution
Knowledge Check
If the surface tension of water is 0.06 N/m, then the capillary rise in a tube of diameter 1mm is (θ=0∘)
A1.22 cmB2.44 cmC3.12 cmD3.86 cmAssertion :The contact angle between water and glass is acute.
Reason :The surface of water in the capillary is convex .AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true and reason is false.DIf both assertion and reason are false.A glass capillary tube is of the shape of a truncated cone with an apex angle α so that its two ends have cross sections of different radii. When dipped in water vertically, water rises in it to a high h, where the radius of its cross section is b. If the surface tension of water is S, its density if ρ, and its contact angle with glass is θ, the value of h will be (g is the acceleration due to gravity)
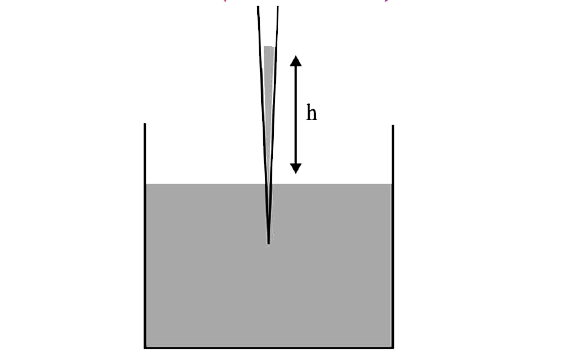 A2Sbρgcos(θ−α)B2Sbρgcos(θ+α)C2Sbρgcos(θ−α/2)D2Sbρgcos(θ+α)
A2Sbρgcos(θ−α)B2Sbρgcos(θ+α)C2Sbρgcos(θ−α/2)D2Sbρgcos(θ+α)
When a vertical capillary of length with the sealed upper end was brought in contact with the surface of a liquid, the level of this liquid rose to the height h. The liquid density is ρ, the inside diameter the capillary is d, the contact angle is θ, the atmospheric pressure is ρ0. Find the surface tension of the liquid. (Temperature this process remains constant.)
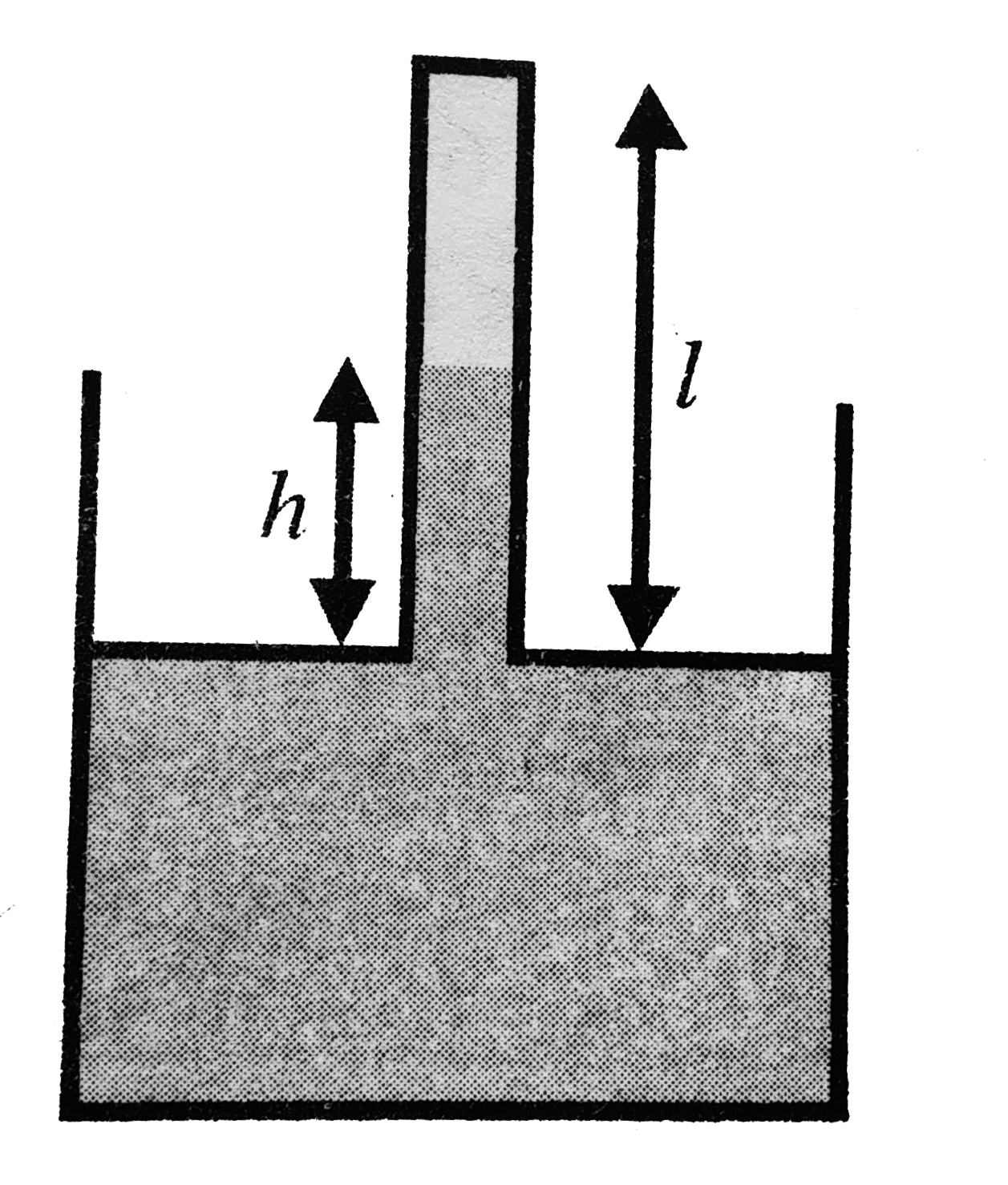
View SolutionWhen a vertical capillary of length l with a sealed upper end was brought in contact with the surface of a liquid, the level of this liquid rose to the height h. The liquid density is ρ, the inside diameter the capillary is d, the contact angle is θ, the atmospheric pressure is ρ0. Find the surface tension of the liquid. (Temperature in this process remains constant.)

View SolutionA capillary tube with inner cross-section in the form of a square of side a is dipped vertically in a liquid of density ρ and surface tension ρ which wet the surface of capillary tube with angle of contact θ . The approximate height to which liquid will be raised in the tube is : (Neglect the effect of surface tension at the corners of capillary tube)
View SolutionA uniform capillary tube of inner radius r is dipped vertically into a beaker filled with water. The water rises to a height h in the capillary tube above the water surface in the beaker. The surface tension of water is σ. The angle of contact between water and the wall of the capillary tube is θ. Ignore the mass of water in the meniscus. Which of the following statements is (are) true?
View SolutionA glass capillary tube is of the shape of a truncated cone with an apex angle α so that its two ends have cross sections of different radii. When dipped in water vertically, water rises in it to a high h, where the radius of its cross section is b. If the surface tension of water is S, its density if ρ, and its contact angle with glass is θ, the value of h will be (g is the acceleration due to gravity)

View Solution
