Among two interfering sources, let S1 be ahead of the phase by 90∘ relative to S2 . If an observation point P is such that PS1−PS2=1.5λ , the phase difference between the waves from S1andS2 reaching P is
(a)3π
(b)5π2
(c)7π2
(d)4π
The correct Answer is:B
To solve the problem, we need to determine the phase difference between the waves from two sources S1 and S2 at an observation point P, given that S1 is ahead in phase by 90∘ (or π2 radians) relative to S2 and that the path difference PS1−PS2=1.5λ.
1. Identify the phase of the sources:
- The phase of source S1 is given as ϕ1=π2 (90 degrees).
- The phase of source S2 is ϕ2, which we need to calculate based on the path difference.
2. Calculate the phase difference due to path difference:
- The phase difference Δϕ due to the path difference Δx=PS1−PS2 can be calculated using the formula:
Δϕ=2πλΔx
- Substituting Δx=1.5λ:
Δϕ=2πλ×1.5λ=3π
3. Determine the total phase of S2:
- Since S1 is ahead by π2, the phase of S2 can be expressed as:
ϕ2=Δϕ+ϕ1=3π+π2
4. Combine the phases:
- To combine the phases, convert 3π into a fraction with a common denominator:
3π=6π2
- Now, adding ϕ1:
ϕ2=6π2+π2=7π2
5. Calculate the net phase difference:
- The net phase difference ΔΦ between the waves from S1 and S2 at point P is:
ΔΦ=ϕ2−ϕ1=7π2−π2=6π2=3π
6. Final Result:
- The phase difference between the waves from S1 and S2 reaching point P is 3π.
Conclusion:
The phase difference between the waves from S1 and S2 reaching point P is 3π.
- The phase of source S1 is given as ϕ1=π2 (90 degrees).
- The phase of source S2 is ϕ2, which we need to calculate based on the path difference.
- The phase difference Δϕ due to the path difference Δx=PS1−PS2 can be calculated using the formula:
Δϕ=2πλΔx
- Substituting Δx=1.5λ:
Δϕ=2πλ×1.5λ=3π
- Since S1 is ahead by π2, the phase of S2 can be expressed as:
ϕ2=Δϕ+ϕ1=3π+π2
- To combine the phases, convert 3π into a fraction with a common denominator:
3π=6π2
- Now, adding ϕ1:
ϕ2=6π2+π2=7π2
- The net phase difference ΔΦ between the waves from S1 and S2 at point P is:
ΔΦ=ϕ2−ϕ1=7π2−π2=6π2=3π
- The phase difference between the waves from S1 and S2 reaching point P is 3π.
The phase difference between the waves from S1 and S2 reaching point P is 3π.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 2 More Than One Correct6videos
SUPERPOSITION OF WAVES
- BOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - More than One correct Options1videos
SUPERPOSITION OF WAVES
- BOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Subjective Questions6videos
SUPERPOSITION OF WAVES
- BOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise 19.74videos
SOUND WAVES
- BOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary30videos
THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASES
Similar Questions
Among the two interfering monochromatic sources A and B, A is ahead of B in phase by 66∘. If the observation be taken from point P, such that PB−PA=λ/4. Then the phase difference between the waves from A and B reaching P is
View SolutionTwo waves of equal amplitude a from two coherent sources (S1&S2) interfere at a point R such that S2R−S1R=1.5λThe intensity at point R Would be
View SolutionSounds from two identical sources S1 and S2 reach a point P. When the sounds reach directly, and in the same phase, the intensity at P is I0. The power of S1 is now reduced by 64% and the phase difference between S1 and S2 is varied continuously. The maximum and minimum intensities recorded at P are now Imax and Imin
View SolutionWhat is the shape of 1s and 2s orbital .Give two point of difference between 1s and 2s orbital
View SolutionA particle is observed from two frames S1andS2. Lthe frame S2 moves with respect toS1 with an acceleration a. Let F1andF2 be the pseudo forces on the particle when seen from S1andS2 respectively. Which of the following are not possible/
View SolutionP is a variable point on the ellipse with foci S1 and S2. If A is the area of the the triangle PS1S2, the maximum value of A is
View SolutionIn the arrangement shown, a screen is placed normal to the line joining the two point coherent sources S1 and S2. The interference pattern consist of concentric circles. λ is the wavelength of light (D>>d).
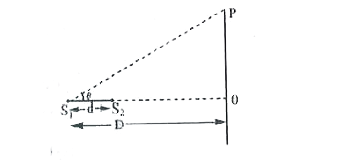
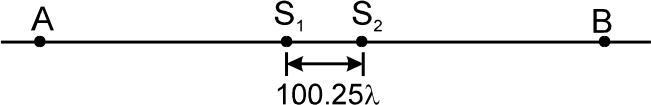
The phase difference of the interfering light at point P isView SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
 View Solution
View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
 View Solution
View SolutionTwo points are located at a distance of 10m and 15m from the source of oscillation. The period of oscillation is 0.05s and the velocity of the wave is 300m/s. What is the phase difference between the oscillation of two points?
View Solution

