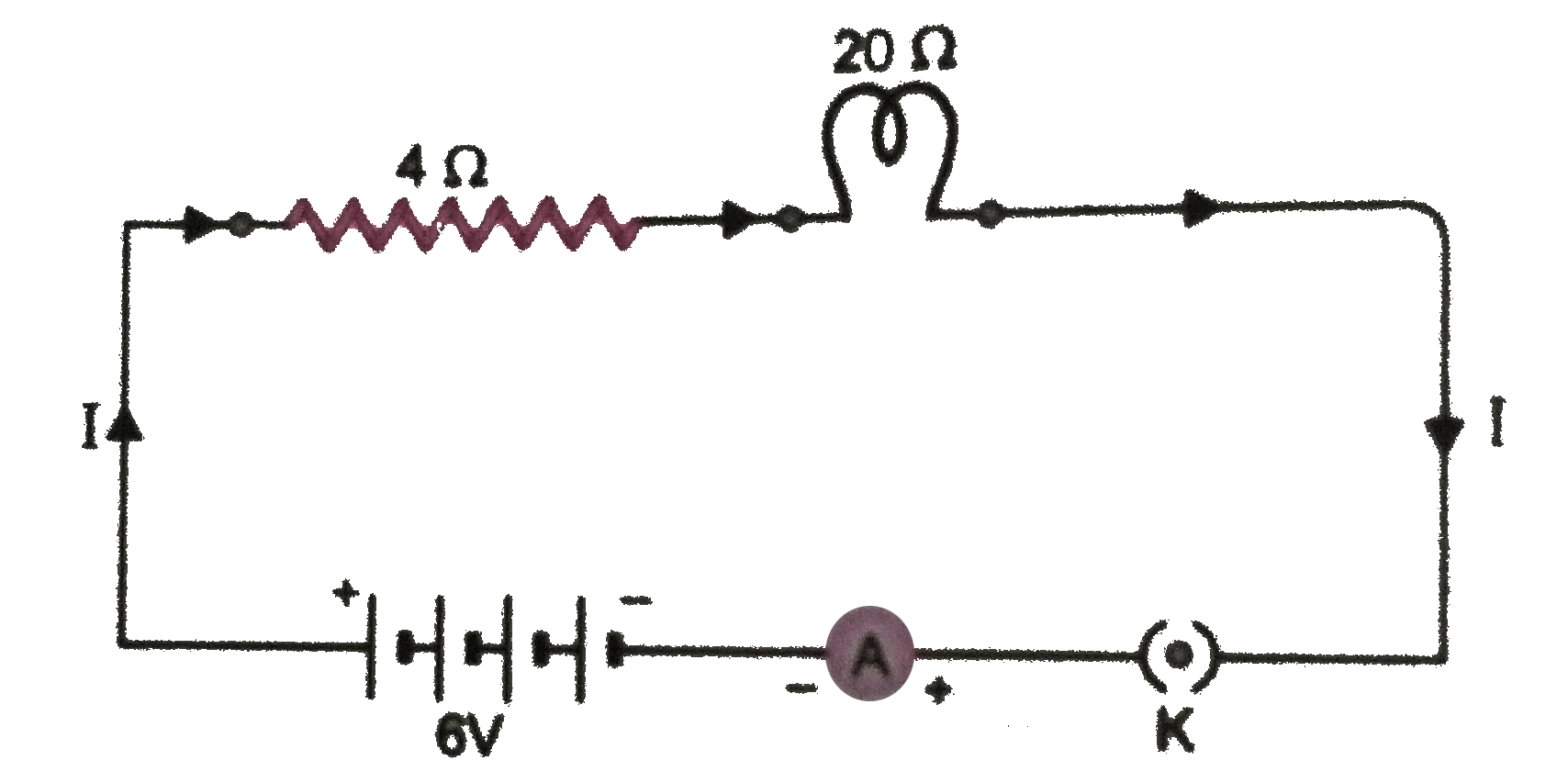
An electric lamp, whose resistance is 20Ω and a conductor of 4Ω resistance are connected to a 6V battery as shown in (Fig. 3.18) Calculate.
(a) the total resistance of the circuit,
(b) the current through the circuit, and
( c) the potential difference across the electric lamp and the conductor.
 .
.
The resistance of electric lamp, R1=20Ω,
The resistance of the conductor connected in series, R2=4Ω.
Then the total resistance in the circuit
R=R1+R2
RS=20Ω+4Ω=24Ω.
The total potential difference across the two terminals of the battery
V = 6 V.
Now by Ohm's law, the current through the circuit is given by
I=C/Rs
=6V/24Ω
=0.25A.
Applying Ohm's law to the electric lamp and conductor separately. we get potential difference across the electric lamp.
V1=20Ω×0.25A
=5V,
and,
That across the conductor,V2=4Ω×0.25A
1V.
Suppose that we like to replace the series combination of electric lamp and conductor by a single and equivalent resistor. Its resistance much be such that a potential difference of 6V across the battery terminals will cause a current of 0.25 A in the ciruit. The resistance R of this equivalent resistor would be
R=VB/I
=6V/0.25A
=24Ω.
This is the total resistance of the series circuit, it is equal to the sum of the resistances.
Topper's Solved these Questions
Similar Questions
An electric lamp of resistance 2Ω and a conductor of resistance 4Ωare connected to a
6V Battery as shown in the circuit. Calculate:
(a) the totak resistance of the circuit,
(b) the current through the circuit,
(c) the potential difference across the (i) electric lamp
and (ii) conductor, and
(d) power of the lamp.
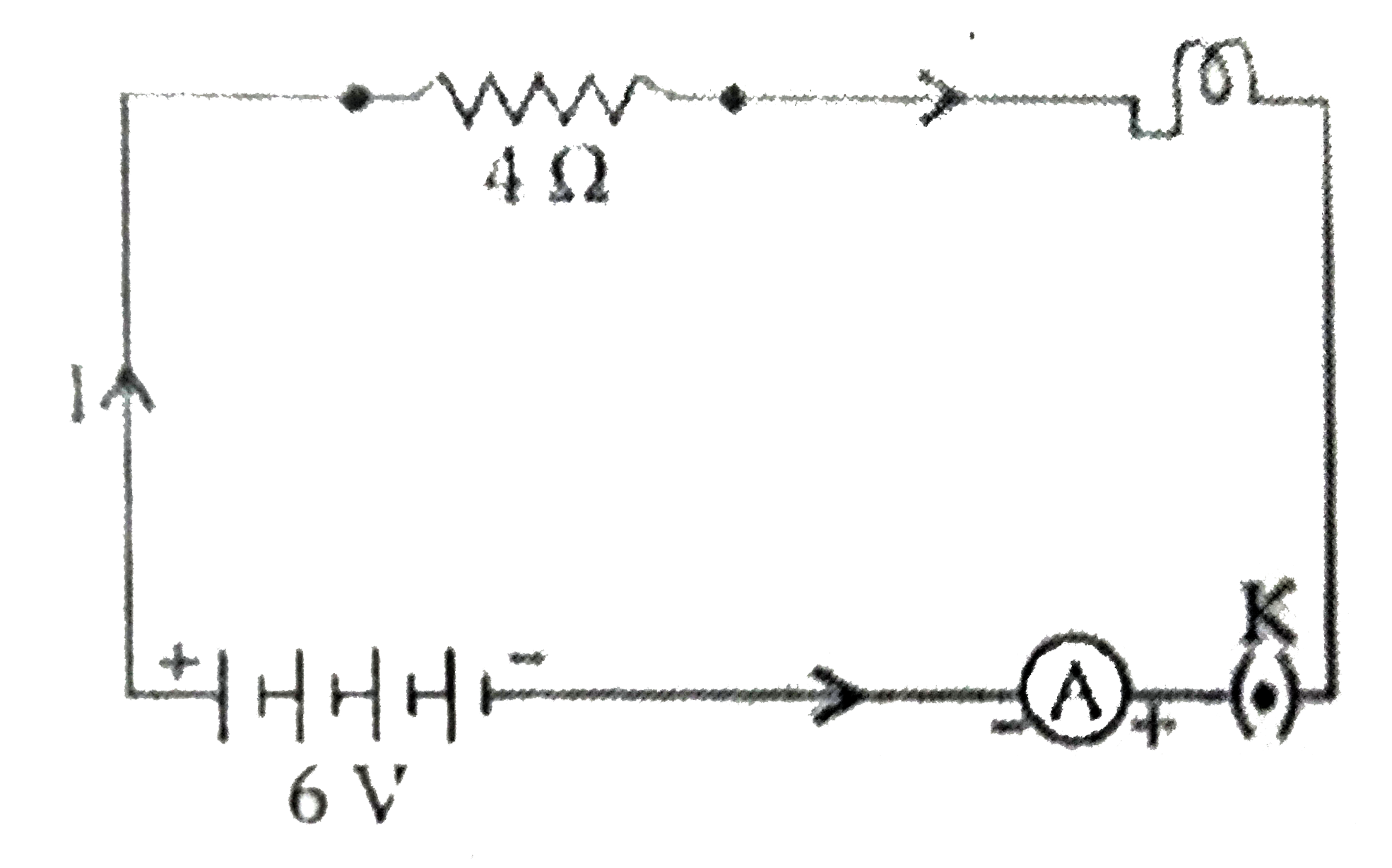
View SolutionIn the given circuit, calculate -
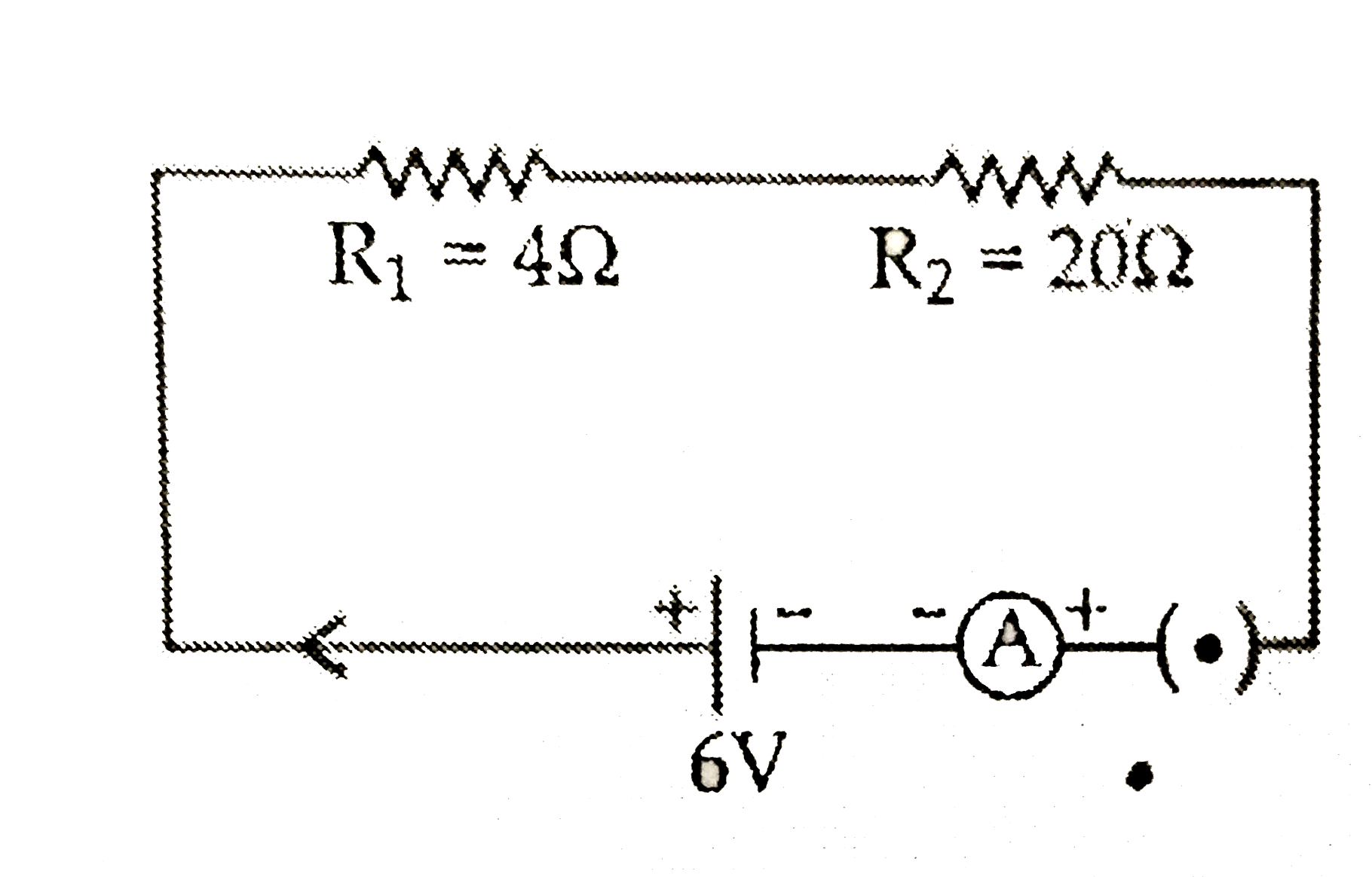
(a) the total resistance of the circuit,
(b) the current through the circuit, and
(c) the potential differences across R1andR2
View SolutionThe diagram below in Fig. shows the arrangement of five different resistances connected to a battery of e.m.f. 1.8 V. Calculate :
the total resistance of the circuit,
View SolutionThree resistors of6.0Ω,2.0Ω and 4.0Ω are joined to an ammeter A and a cell of e.m.f. 6-0 V as shown in Fig. Calculate :
the effective resistance of the circuit,
View SolutionIn the circuit shown, the current in 3Ω resistance is
View SolutionAs electric lamp and a conductor of resistance 4Ω are connected in series to a 6 V battery. The current drawn by the lamp is 0.25 A. Find the resistance of the electric lamp.
View SolutionIn the circuit Fig the final current through 30Ω resistance when circuit is completed is
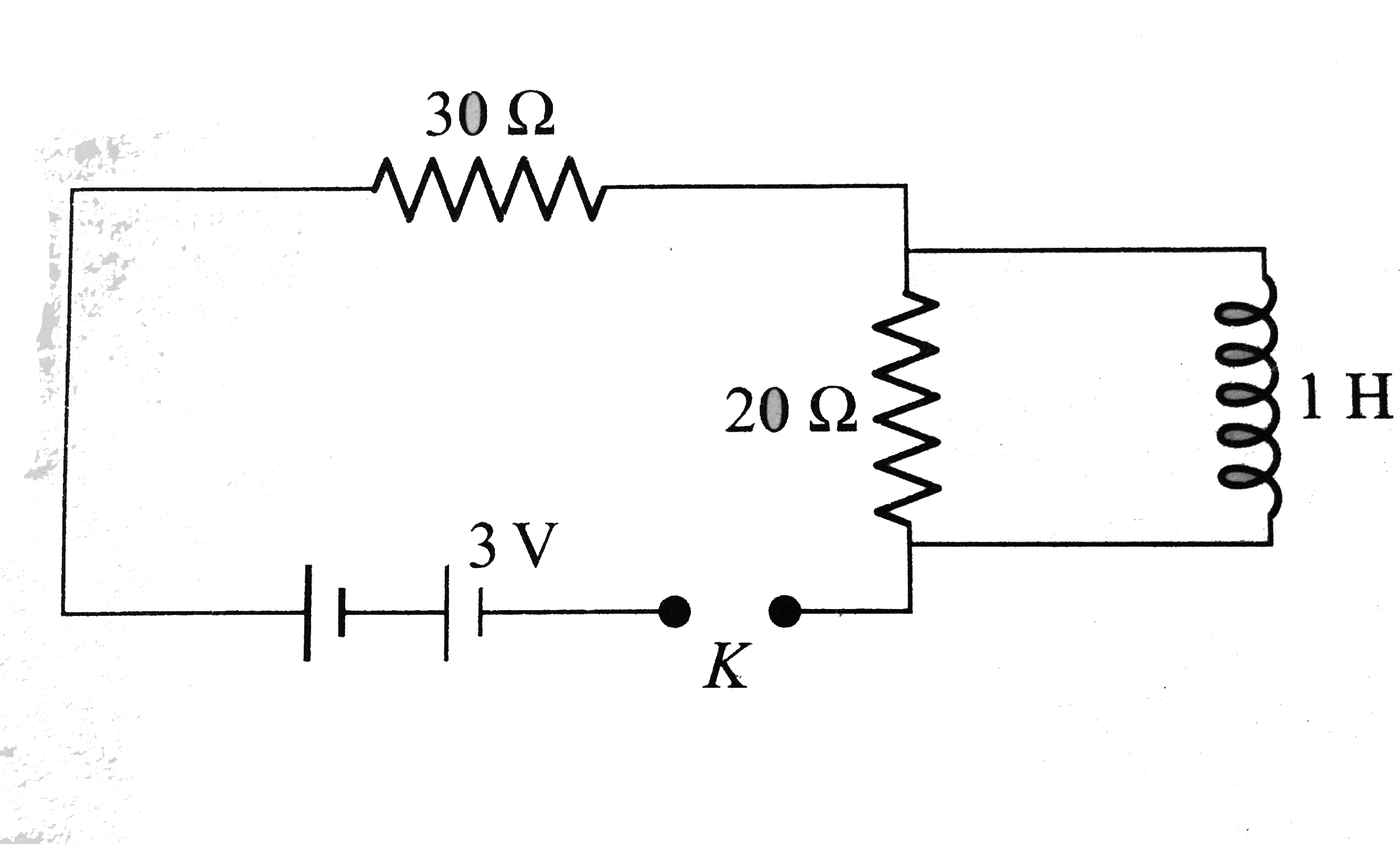
View Solution(a) A current of 1A flows in a series curcuit containing an electric lamp and a conductor of 5Ω when connected to a 10V battery. Calculate the resistance of the electric lamp.
(b) Now if a resistance of 10Ω is connected in parallel with this series combination, what change (if any) in current flowing through 5Ω conductor and potential difference across the lamp will take place ? Give reason.
View SolutionTwo resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate : (a) the total resistance of the circuit,
View SolutionTwo resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate :the current through the battery
View Solution
