An infinitely long thin straight wire has uniform linear charge density of 1/3coulm−1 .Then the magnitude of the electric intensity at a point 18cm away is
0.33×1011NC−1
3×1011NC−1
0.66×1011NC−1
1.32×1011NC−1
The correct Answer is:A
To find the electric field intensity (E) at a point 18 cm away from an infinitely long thin straight wire with a uniform linear charge density (λ) of 13C/m, we can use the formula for the electric field due to an infinitely long charged wire:
E=λ2πϵ0R
where:
- E is the electric field intensity,
- λ is the linear charge density,
- ϵ0 is the permittivity of free space (ϵ0≈8.85×10−12C2/N m2),
- R is the distance from the wire.
Step 1: Convert the distance from centimeters to meters
Given distance R=18cm:
R=18cm=18×10−2m=0.18m
Step 2: Substitute the values into the formula
Given:
- λ=13C/m
- ϵ0≈8.85×10−12C2/N m2
Now, substitute these values into the electric field formula:
E=132π(8.85×10−12)(0.18)
Step 3: Calculate the denominator
First, calculate 2πϵ0R:
2πϵ0R=2π(8.85×10−12)(0.18)
Calculating this gives:
2π(8.85×10−12)(0.18)≈1.00×10−12C2/N m2
Step 4: Calculate the electric field intensity
Now substitute back into the formula:
E=131.00×10−12≈0.3331.00×10−12≈3.33×1011N/m2
Final Answer
The magnitude of the electric field intensity at a point 18 cm away from the wire is approximately:
E≈3.33×1011N/m2
- E is the electric field intensity,
- λ is the linear charge density,
- ϵ0 is the permittivity of free space (ϵ0≈8.85×10−12C2/N m2),
- R is the distance from the wire.
Given distance R=18cm:
R=18cm=18×10−2m=0.18m
Given:
- λ=13C/m
- ϵ0≈8.85×10−12C2/N m2
E=132π(8.85×10−12)(0.18)
First, calculate 2πϵ0R:
2πϵ0R=2π(8.85×10−12)(0.18)
Calculating this gives:
2π(8.85×10−12)(0.18)≈1.00×10−12C2/N m2
Now substitute back into the formula:
E=131.00×10−12≈0.3331.00×10−12≈3.33×1011N/m2
The magnitude of the electric field intensity at a point 18 cm away from the wire is approximately:
E≈3.33×1011N/m2
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Assertion and Reason
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
An infinitely long thin straight wire has uniform linear charge density of 1/4 coul m−1. Then the magnitude of the electric intensity at a point 18 cm away is
View SolutionA long thin flat sheet has a uniform surface charge density σ. The magnitude of the electric field at a distance .r. from it is given by
View Solution
Knowledge Check
If a long straight wire carries a current of 40 A, then the magnitude of the field B at a point 15 cm away from the wire is
A5.34×10−5TB8.34×10−5TC9.6×10−5TD10.2×10−5T
If the linear charge density of a cylinder is 4μCm−6 ,then electric field intensity at point 3.6 cm from axis is :
View SolutionTwo infinitely long thin straight wires having uniform linear charge densities λ and 2λ are arranged parallel to each other at a distance r apart.The intensity of the electric field at a point midway between them is
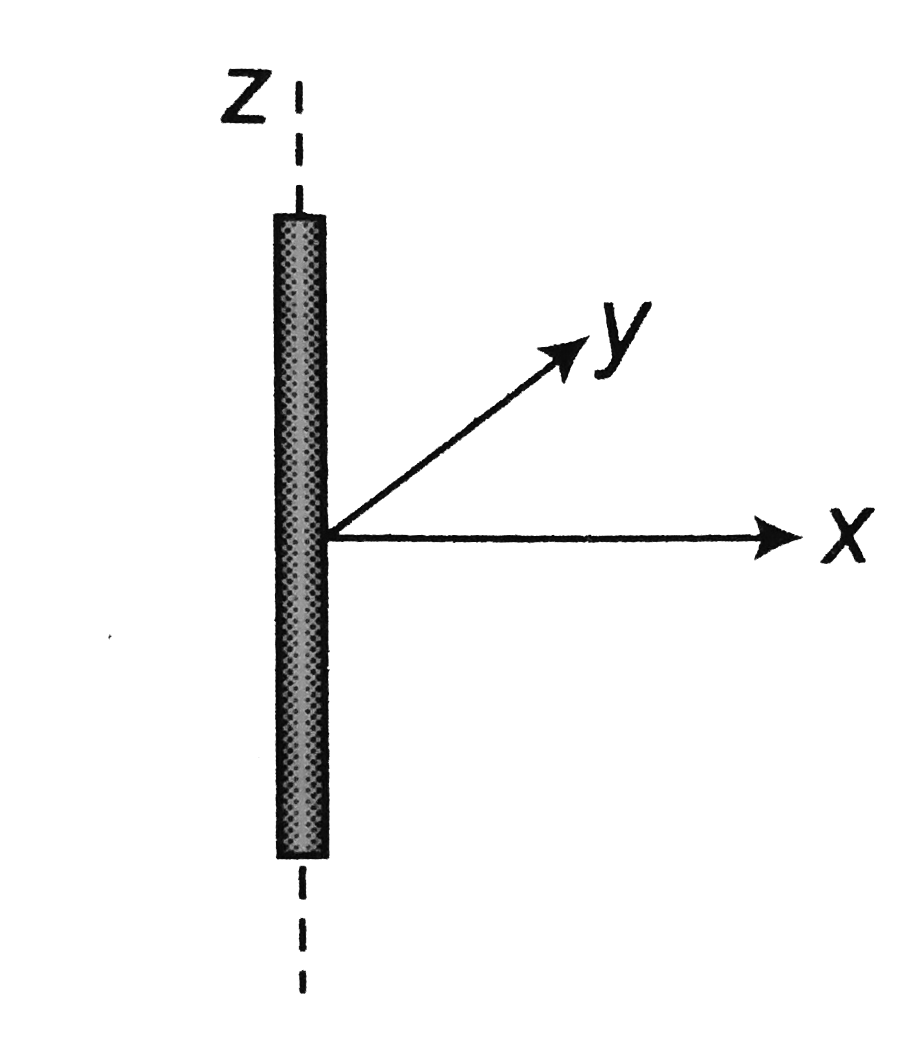
View SolutionAn infinitely long wire is kept along z-axis from z=−∞ to z=∞, having uniform linear charge density 109nC/m. The electric field at point (6cm,8cm,10cm) will be
View SolutionFigure shows a long wire having uniform charge density λ as shown in figure. Calculate electric field intensity at point P.
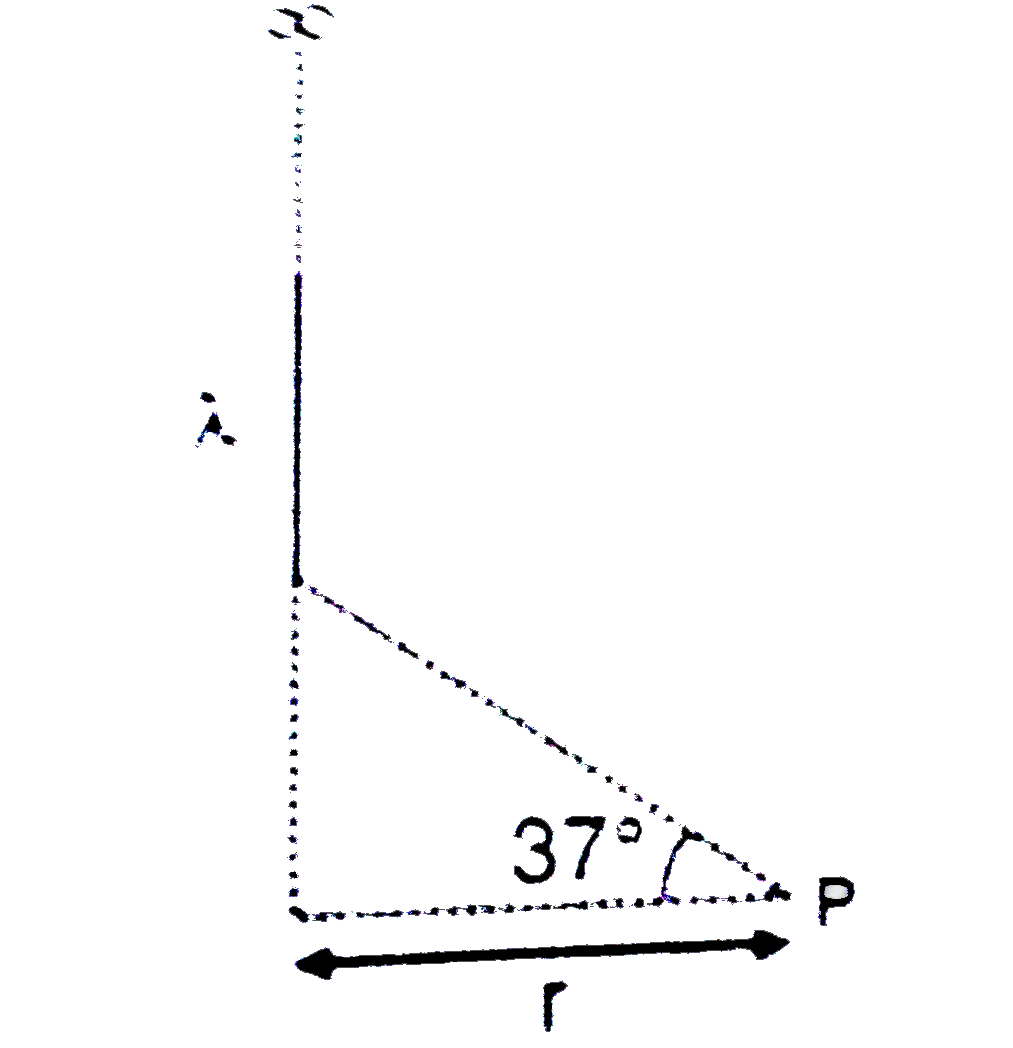
View SolutionA long wire with a uniform charge density λ is bent in two configurations shown in fig. Determine the electric field intensity at point O.
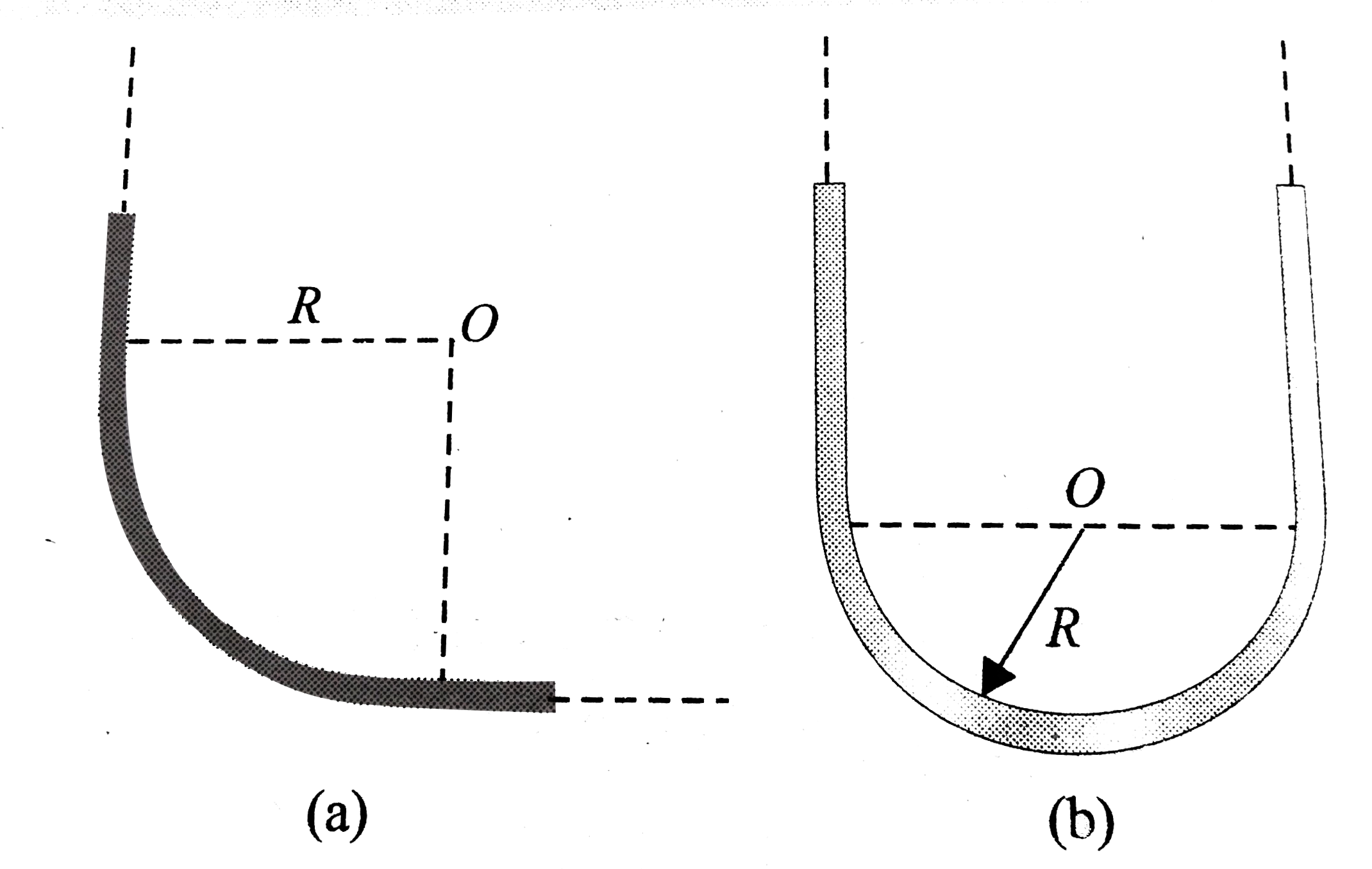
View SolutionAn infinitely long wire is kept along z-axis from z=∞ to z=∞, having uniform linear cahrges density 109nC/m. The electric field at point (6cm,8cm,10cm) will be

View SolutionAn infinitely long wire is kept along z-axis from z=∞ to z=∞, having uniform linear cahrges density 109nC/m. The electric field at point (6cm,8cm,10cm) will be

View Solution
