As the position of an object (u) reflected from a concave mirror is varied, the position of the image (v) also varies. By latting the u changes from 0 to +∞ the graph between v versus u will be
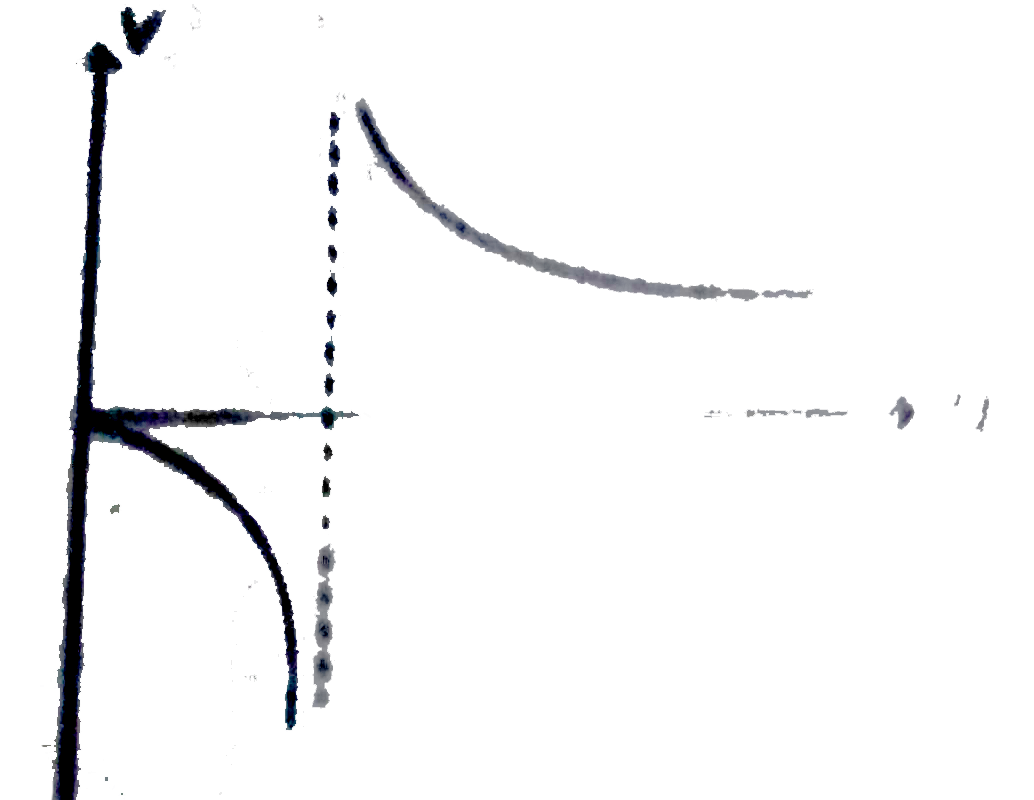
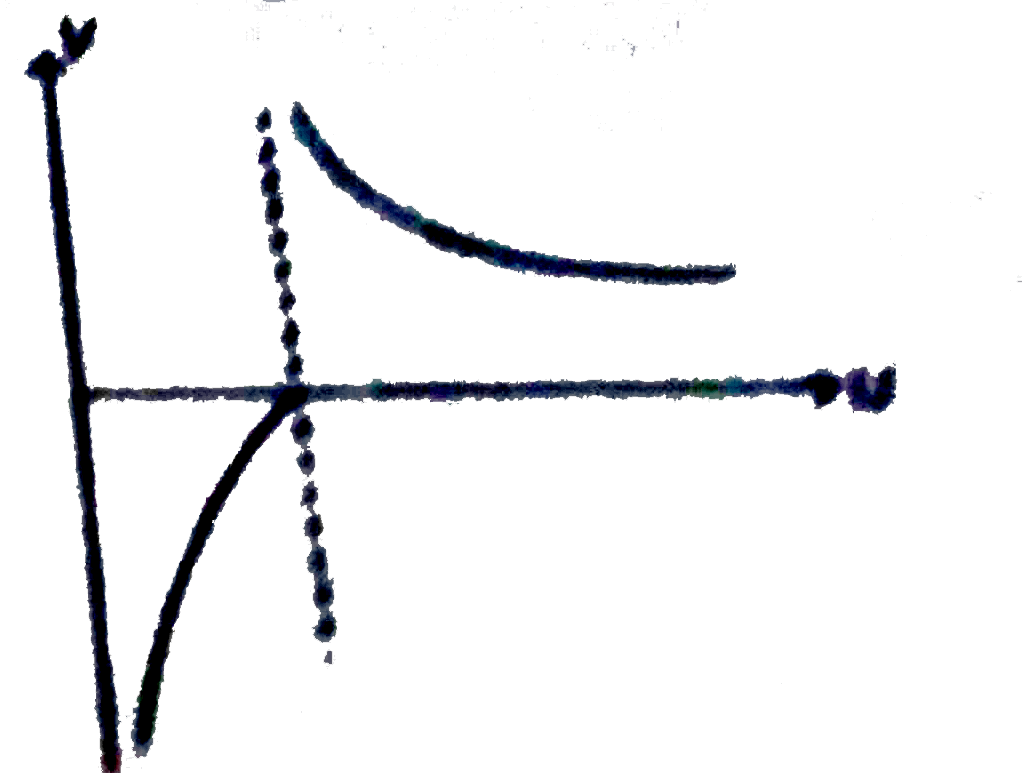


The correct Answer is:A
To solve the problem of finding the relationship between the object distance (u) and the image distance (v) for a concave mirror, we will use the mirror formula and analyze the behavior of the graph as u varies from 0 to +∞.
1. Understand the Mirror Formula:
The mirror formula for a concave mirror is given by:
1f=1v+1u
Rearranging this gives:
1v=1f−1u
or equivalently,
v=ufu−f
2. Analyze the Behavior as u Approaches 0:
- When u=0:
v=0⋅f0−f=0
- Thus, when the object is at the pole of the mirror, the image is also at the pole.
3. Analyze the Behavior as u Approaches +∞:
- When u approaches +∞:
v=u⋅fu−f≈f(since u is much larger than f)
- Therefore, as the object moves far away, the image distance approaches the focal length f.
4. Identify Key Points:
- At u=0, v=0.
- As u→+∞, v→f.
5. Graphing the Relationship:
- The graph starts at the origin (0,0) and approaches the line v=f as u increases.
- The curve will be hyperbolic in nature, reflecting that as u increases, v approaches a constant value (the focal length).
6. Conclusion:
- The graph of v versus u will start from the origin and will asymptotically approach the line v=f as u increases.
The mirror formula for a concave mirror is given by:
1f=1v+1u
Rearranging this gives:
1v=1f−1u
or equivalently,
v=ufu−f
- When u=0:
v=0⋅f0−f=0
- Thus, when the object is at the pole of the mirror, the image is also at the pole.
- When u approaches +∞:
v=u⋅fu−f≈f(since u is much larger than f)
- Therefore, as the object moves far away, the image distance approaches the focal length f.
- At u=0, v=0.
- As u→+∞, v→f.
- The graph starts at the origin (0,0) and approaches the line v=f as u increases.
- The curve will be hyperbolic in nature, reflecting that as u increases, v approaches a constant value (the focal length).
- The graph of v versus u will start from the origin and will asymptotically approach the line v=f as u increases.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - B. More than one option is correct
20 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - C. comprehension type question
14 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
Similar Questions
The graph between u and v for a convex mirrorr is
View SolutionThe erect image formed by a concave mirror is of size double the size of object. How are u and v related?
View SolutionThe graph between 1v and 1u for a concave mirror looks like.
View SolutionThe graph between 1v and 1u for a concave mirror looks like.
View SolutionA real inverted image in a concave mirror is represented by (u,v, f are corrdinates)
View SolutionAn object is brought from a far distance towards a concave mirror. How do the nature, position and size of image change?
View SolutionIn an optics experiment, with the position of the object fixed, a student varies the position of a convex lens and for each position, the screen is adjusted to get a clear image of the object. A graph between the object distance u and the image distance v, from the lens, is plitted using the same scale for the two axes. A straight line passing through the origin and making an angle of 45∘ with x-axis meets the experimental curve at P. The coordinates of P will be.
View SolutionAn object is placed at a distance u from a concave mirror and its real image is received on a screen placed at a distance of v from the mirror. If f is the focal length of the mirror, then the graph between 1/v versus 1/u is
View SolutionFor an object placed at a distance 20 cm from a concave mirror, the image is formed at the same position. What is the focal length of the mirror?
View SolutionAn object 2 mm high is placed 150 mm from a concave mirror of focal length 5 cm. Find the position (in mm) and size (in mm) of the image.
View Solution
