Assertion : In series L−C−R circuit, if a ferromagnetic rod is inserted inside an inductor, current in the circuit may increase or decrease.
Reason : By doing so XL will increase.
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:A, B
To solve the question, we need to analyze both the assertion and the reason provided:
Step 1: Understand the Assertion
The assertion states that in a series L-C-R circuit, if a ferromagnetic rod is inserted inside an inductor, the current in the circuit may either increase or decrease.
Hint: Consider how the insertion of a ferromagnetic material affects the inductance of the inductor.
Step 2: Understand the Reason
The reason states that by inserting a ferromagnetic rod, the inductive reactance XL will increase.
Hint: Recall the relationship between inductance and inductive reactance.
Step 3: Analyze the Effect on Inductance
When a ferromagnetic material is inserted into an inductor, the inductance L of the inductor increases. The inductive reactance XL is given by the formula:
XL=ωL
where ω is the angular frequency of the AC source. Therefore, if L increases, XL will also increase.
Hint: Remember that XL is directly proportional to inductance.
Step 4: Consider the Impedance in the Circuit
The total impedance Z in a series L-C-R circuit is given by:
Z=√R2+(XL−XC)2
where XC is the capacitive reactance.
Hint: Think about how changes in XL and XC affect the overall impedance Z.
Step 5: Evaluate Current in the Circuit
The current I in the circuit can be expressed as:
I=VZ
where V is the voltage across the circuit.
Hint: Consider how changes in Z affect the current I.
Step 6: Analyze Different Scenarios
1. Case A: If XC is greater than XL initially, and XL increases after inserting the rod, the difference XL−XC could decrease, leading to an increase in impedance Z and thus a decrease in current I.
2. Case B: If XL is initially greater than XC, and XL increases further, the difference could increase, leading to an increase in impedance Z and a decrease in current I.
Hint: Think about how the relationship between XL and XC determines the behavior of the current.
Step 7: Conclusion
Since the impedance Z can either increase or decrease depending on the values of XL and XC, the current I can also either increase or decrease. Thus, the assertion is true. However, the reason provided does not adequately explain the assertion, as it only states that XL increases without considering the effect of XC.
Final Answer: Both the assertion and reason are true, but the reason is not the correct explanation of the assertion.
The assertion states that in a series L-C-R circuit, if a ferromagnetic rod is inserted inside an inductor, the current in the circuit may either increase or decrease.
The reason states that by inserting a ferromagnetic rod, the inductive reactance XL will increase.
When a ferromagnetic material is inserted into an inductor, the inductance L of the inductor increases. The inductive reactance XL is given by the formula:
XL=ωL
where ω is the angular frequency of the AC source. Therefore, if L increases, XL will also increase.
The total impedance Z in a series L-C-R circuit is given by:
Z=√R2+(XL−XC)2
where XC is the capacitive reactance.
The current I in the circuit can be expressed as:
I=VZ
where V is the voltage across the circuit.
1. Case A: If XC is greater than XL initially, and XL increases after inserting the rod, the difference XL−XC could decrease, leading to an increase in impedance Z and thus a decrease in current I.
2. Case B: If XL is initially greater than XC, and XL increases further, the difference could increase, leading to an increase in impedance Z and a decrease in current I.
Since the impedance Z can either increase or decrease depending on the values of XL and XC, the current I can also either increase or decrease. Thus, the assertion is true. However, the reason provided does not adequately explain the assertion, as it only states that XL increases without considering the effect of XC.
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level - 1 Objective
30 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level - 1 Subjective
6 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Exercise 28.2
2 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
Similar Questions
In L−C−R series AC circuit,
View SolutionIn an L-R circuit, if an iron core is inserted inside the coil
View SolutionAt resonance in series R-L-C circuit
_PHY_E06_308_Q01.png)
View SolutionAssertion: When a ferromagnetic rod is inserted inside an inductor, then current in L-C-R, alternating circuit will decrease.
Reason: By inserting the ferromagnetic rod inside the inductor, coefficient of self induction and hence the net impedance will increases.
View SolutionWhen a material is inserted inside the inductor the current in the circuit increases, then the nature of the material is
View SolutionIn L−C−R series circuit
View SolutionA series L-C-R circuit is operated at resonance . Then
View SolutionFor a series L C R circuit, the power loss at resonance is
View SolutionIn the series L-C-R circuit , the voltmeter and ammeter readings are
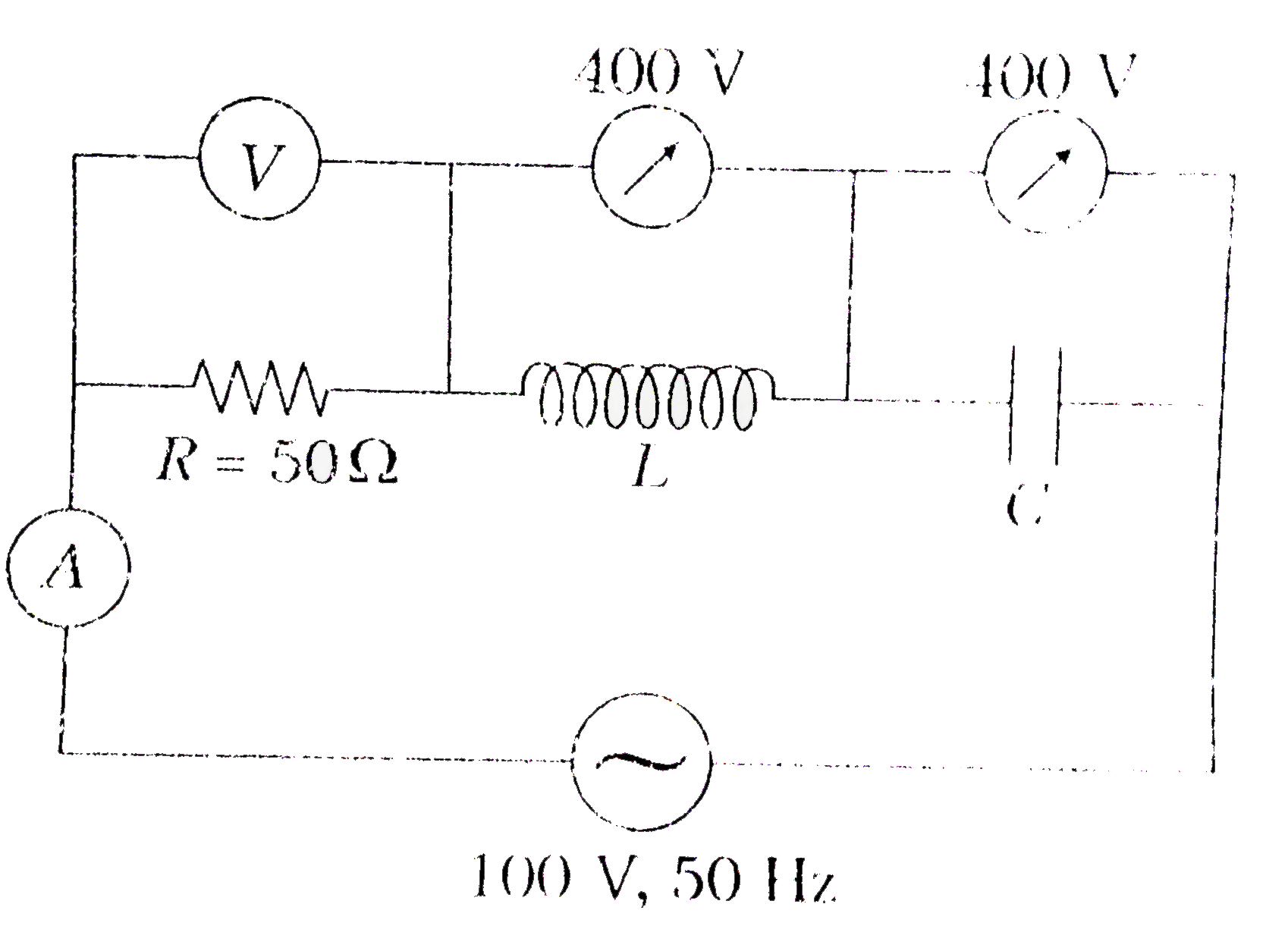View SolutionIn the series L-C-R circuit , the voltmeter and ammeter readings are
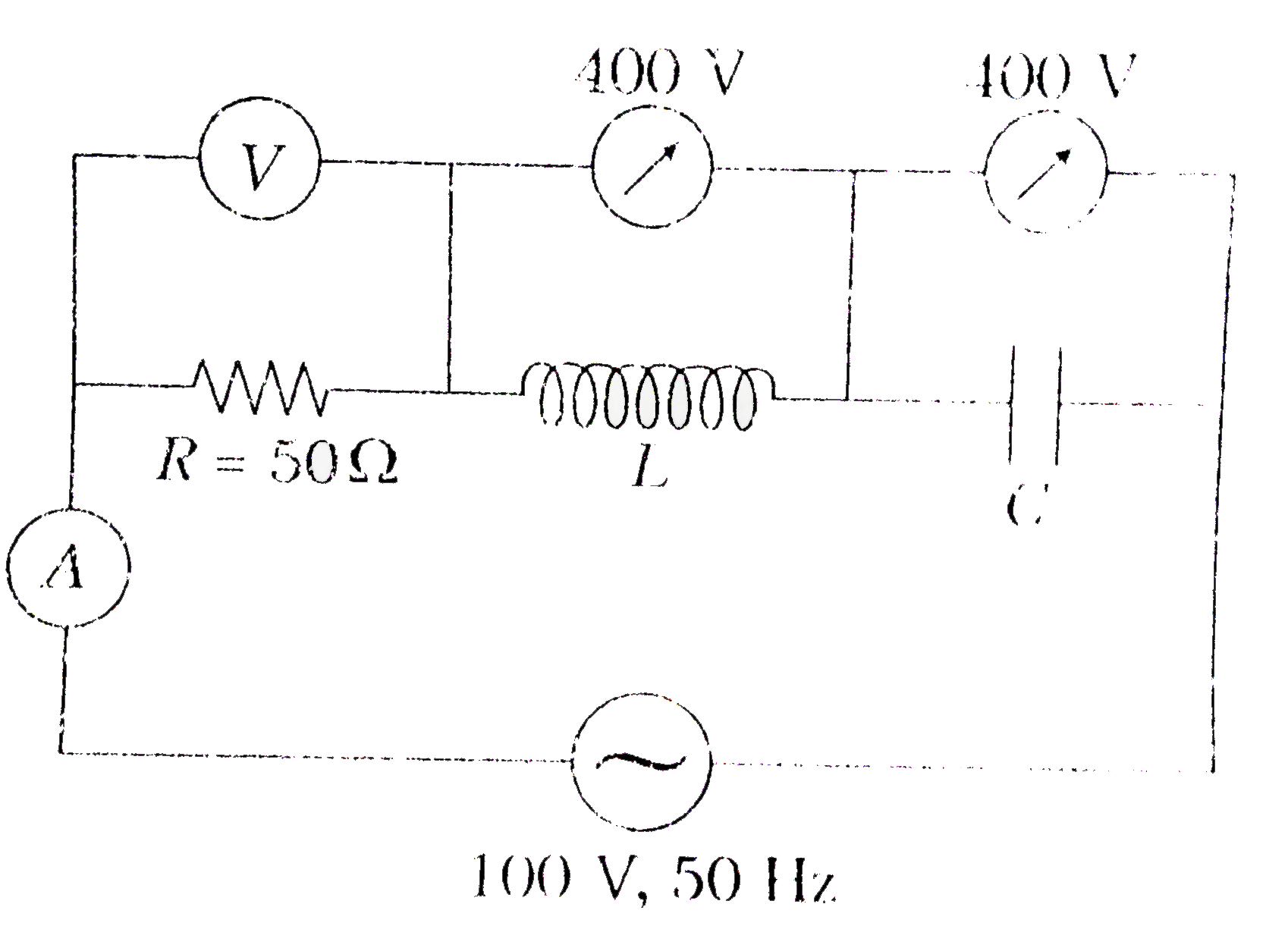View Solution