Assertion : Peak value of current in AC through a resistance of 10Ω is 2A. Then, power consumed by the resistance should be 20W.
Reason : Power in AC is P=I2rmsR
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:A, B
To solve the problem, we will analyze the assertion and reason provided in the question step-by-step.
Step 1: Understand the Assertion
The assertion states that the peak value of the current (I₀) in an AC circuit through a resistance of 10 ohms is 2 A. We need to determine if the power consumed by the resistance is indeed 20 W.
Step 2: Calculate RMS Current
The RMS (Root Mean Square) value of the current (I_rms) can be calculated from the peak current (I₀) using the formula:
Irms=I0√2
Substituting the given peak current:
Irms=2A√2=21.414≈1.414A
Step 3: Use the Power Formula
The power consumed by the resistor (P) can be calculated using the formula:
P=I2rms⋅R
Substituting the values we have:
P=(1.414A)2⋅10Ω
Calculating (1.414)2:
(1.414)2≈2
Thus:
P≈2⋅10=20W
Step 4: Conclusion
The assertion is correct as the power consumed by the resistance is indeed 20 W. The reason provided, which states the formula for power in AC circuits, is also correct. Therefore, both the assertion and reason are true, and the reason correctly explains the assertion.
Final Answer
Both the assertion and reason are true, and the reason is the correct explanation of the assertion.
---
The assertion states that the peak value of the current (I₀) in an AC circuit through a resistance of 10 ohms is 2 A. We need to determine if the power consumed by the resistance is indeed 20 W.
The RMS (Root Mean Square) value of the current (I_rms) can be calculated from the peak current (I₀) using the formula:
Irms=I0√2
Substituting the given peak current:
Irms=2A√2=21.414≈1.414A
The power consumed by the resistor (P) can be calculated using the formula:
P=I2rms⋅R
Substituting the values we have:
P=(1.414A)2⋅10Ω
Calculating (1.414)2:
(1.414)2≈2
Thus:
P≈2⋅10=20W
The assertion is correct as the power consumed by the resistance is indeed 20 W. The reason provided, which states the formula for power in AC circuits, is also correct. Therefore, both the assertion and reason are true, and the reason correctly explains the assertion.
Both the assertion and reason are true, and the reason is the correct explanation of the assertion.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level - 1 Objective30videos
ALTERNATING CURRENT
- BOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Level - 1 Subjective6videos
ALTERNATING CURRENT
- BOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Exercise 28.22videos
ALTERNATING CURRENT
- BOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY42videos
ATOMS
Similar Questions
Find the current through 2Ω and 4Ω resistance.
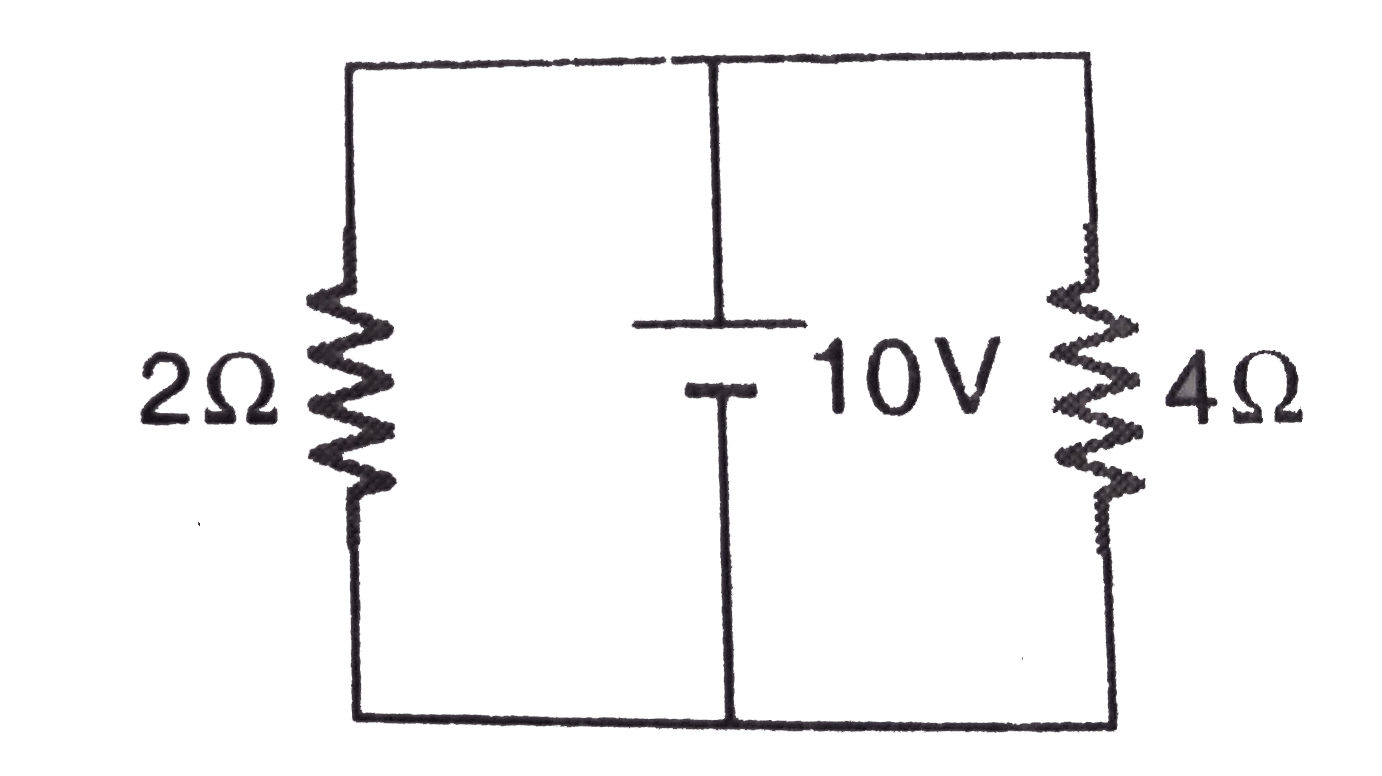 View Solution
View SolutionThe internal resistance of a 2.1V cell which gives a current 0.2A through a resistance of 10Ω
View SolutionThe internal resistance of a 2.1 V cell which gives a current of 0.2A through a resistance of 10Ω is
View SolutionIn the circuit shown, current through the resistance2Ω isi1 and current through the resistance30Ω is i2. The ratio i1i2 is equal to
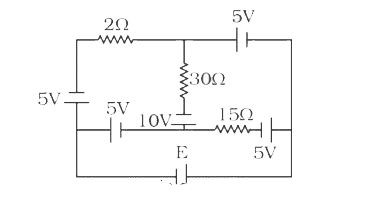 View Solution
View SolutionA sinusoidal ac current flows through a resistor of resistance R . If the peak current is Ip , then the power dissipated is
View SolutionA sinusoidal ac current flows through a resistor of resistance R . If the peak current is Ip , then the power dissipated is
View SolutionIf the cell of emf 5 volt shown in the figure gives a power of 10W. Q, find the powers consumed by the resistors 2Ω and 1Ω
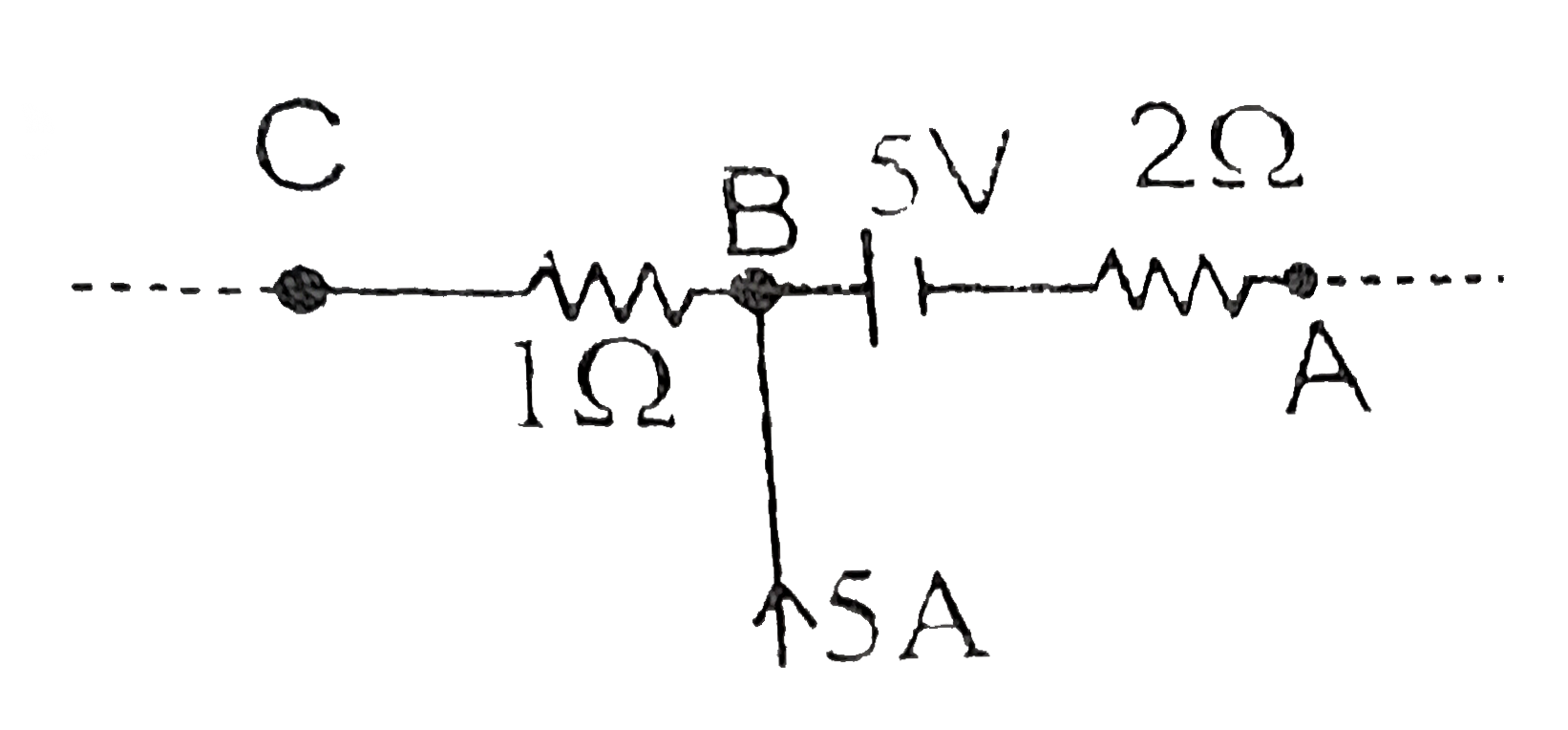 View Solution
View SolutionIf the cell of emf 5 volt shown in the figure gives a power of 10W. Q, find the powers consumed by the resistors 2Ω and 1Ω
 View Solution
View Solution110Vrms is applied across a series circuit having resistance 11Ω and impedance 22Ω . The power consumed is
View SolutionStatement I: When an external resistor of resistance R (connected across a cell to internal resistance r ) is varied, power consumed by resistance R is maximum when R = r.
Statement II: Power consumed by a resistor of constant resistance R is maximum when current through it is maximum.View Solution

