Choose the correct option:
A capillary tube of radius r is lowered into a liquid of surface tension T and density ρ. Given angle of contact =0∘.
The work done by surface tension will be
(a) πT2ρg
(b) 4πT2ρg
(c) T2ρg
(d) 2T2ρg
The correct Answer is:B
To solve the problem step by step, we will derive the work done by surface tension when a capillary tube is immersed in a liquid.
Step 1: Understand the situation
We have a capillary tube of radius r immersed in a liquid with surface tension T and density ρ. The angle of contact is given as 0∘.
Step 2: Determine the height of liquid rise in the capillary tube
The height H to which the liquid rises in the capillary tube can be calculated using the formula:
H=2Tcosθrρg
Given that the angle of contact θ=0∘, we know that:
cos(0∘)=1
Thus, the formula simplifies to:
H=2Trρg
Step 3: Calculate the work done by surface tension
The work done W by the surface tension when the liquid rises can be expressed as:
W=Force×Distance
The force due to surface tension acting around the circumference of the tube is given by:
Force=T×Circumference=T×(2πr)
The distance that the liquid rises is H. Therefore, the work done can be calculated as:
W=T×(2πr)×H
Substituting the expression for H:
W=T×(2πr)×(2Trρg)
Step 4: Simplify the expression
Now, simplifying the expression:
W=T×(2πr)×(2Trρg)=4πT2ρg
Step 5: Identify the correct option
From the derived expression, we see that:
W=4πT2ρg
Thus, the correct option is:
b. 4πT2ρg.
Summary of Steps:
1. Identify the parameters (radius r, surface tension T, density ρ, angle of contact).
2. Use the formula for height of liquid rise in capillary action.
3. Calculate the work done using the force due to surface tension and the height.
4. Simplify the expression to find the final work done.
5. Match the result with the provided options.
We have a capillary tube of radius r immersed in a liquid with surface tension T and density ρ. The angle of contact is given as 0∘.
The height H to which the liquid rises in the capillary tube can be calculated using the formula:
H=2Tcosθrρg
Given that the angle of contact θ=0∘, we know that:
cos(0∘)=1
Thus, the formula simplifies to:
H=2Trρg
The work done W by the surface tension when the liquid rises can be expressed as:
W=Force×Distance
The force due to surface tension acting around the circumference of the tube is given by:
Force=T×Circumference=T×(2πr)
The distance that the liquid rises is H. Therefore, the work done can be calculated as:
W=T×(2πr)×H
Substituting the expression for H:
W=T×(2πr)×(2Trρg)
Now, simplifying the expression:
W=T×(2πr)×(2Trρg)=4πT2ρg
From the derived expression, we see that:
W=4πT2ρg
Thus, the correct option is:
b. 4πT2ρg.
1. Identify the parameters (radius r, surface tension T, density ρ, angle of contact).
2. Use the formula for height of liquid rise in capillary action.
3. Calculate the work done using the force due to surface tension and the height.
4. Simplify the expression to find the final work done.
5. Match the result with the provided options.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Matrix Matching
12 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A capillary tube of radius r is lowered into a liquid of surface tension T and density ρ. Given angle of contact =0∘.
What is the potential energy acquired by the liquid in the capillary?
View SolutionWhat is the force of surface tension on a light ring of radius r floating on a liquid of surface tension γ ?
View SolutionWhat is the surface energy of an air bubble of radius r inside a soap solution with surface tension T ?
View SolutionA student writes the equation for the capillary rise of a liquid in a tube as h=ρg2Tcosθ, where r is the radius of the capillary tube, ρ is the density of liquid, T is the surface tension and θ is the angle of contact. Check the correctness of the equation using dimensional analysis?
View SolutionA tube of radius r and sufficient height is dipped in a liquid of surface tension S and density ρ. Heat developed upto steady state will be
Note: θ is the contact angle
View SolutionA long glass capillary (radius R) is taken out of liquid (surface tension S and density ρ) in vertical position. Angle of contact everywhere is zero. Atmospheric pressure is P0. It is observed that the capillary retains some liquid.
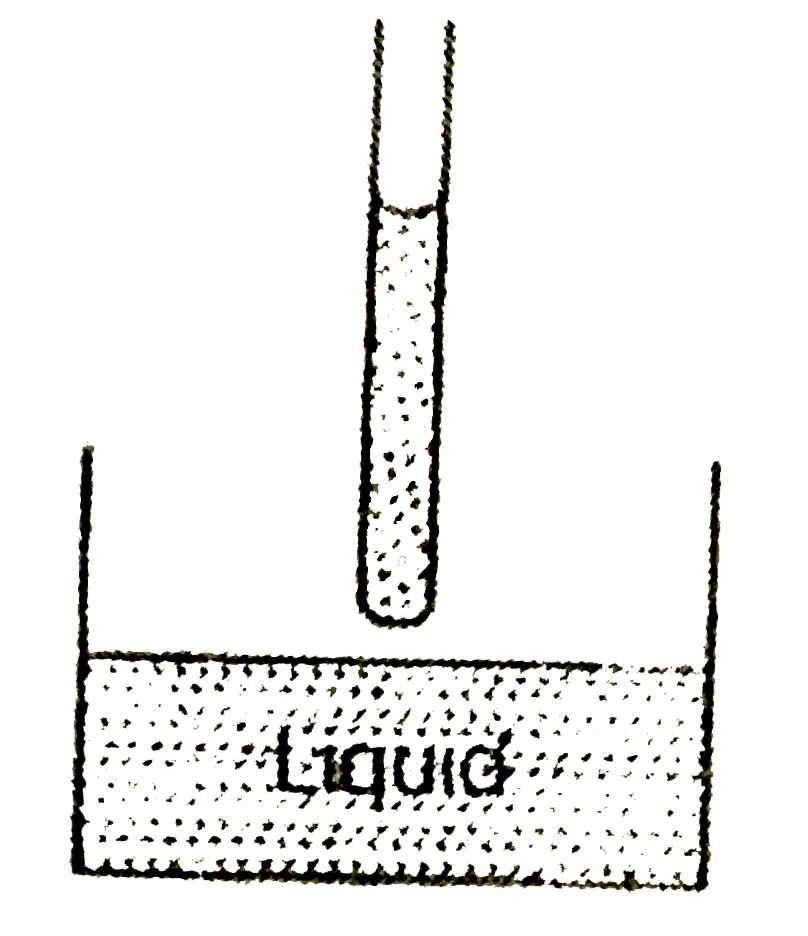
The radius of curvature of meniscus at the bottom of tube is
View SolutionA long glass capillary (radius R) is taken out of liquid (surface tension S and density ρ) in vertical position. Angle of contact everywhere is zero. Atmospheric pressure is P0. It is observed that the capillary retains some liquid.
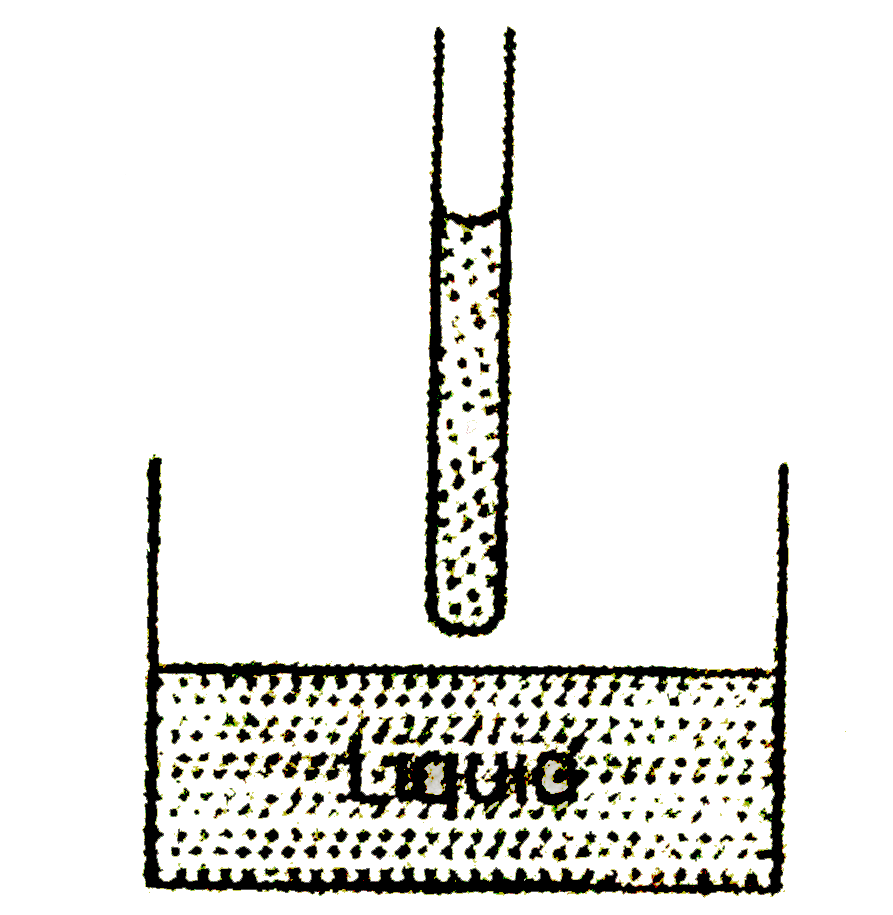
The length of liquid column retained in the capillary is
View SolutionThe depression of mercury in a capillary tube of radius R1 is observed to be equal to the rise of water in another capillary tube of radius R2. If the ratio of surface tension of mercury and water is 7.5, ratio of their density pHgpwater=13.6 and their angle of contact are θHg=135∘ and θwater=0∘ in the respective tubes then R1/R2 is :
View SolutionA paper disc of radius R from which a hole of radius r is cut out is floating in a liquid of the surface tension S. The force on the disc due to the surface tension is
View SolutionA capillary tube of radius 0.6 mm is dipped vertically in a liquid of surface tension 0.04Nm−1and relative density 0.8 . Calculate the height of capillary rise if the angle of contact is 15∘.
View Solution
