A tube of radius r and sufficient height is dipped in a liquid of surface tension S and density ρ. Heat developed upto steady state will be
Note: θ is the contact angle
πS2cos2θρg
πS2cos2θ2ρg
2πS2cos2θρg
4πS2cos2θρg
The correct Answer is:C
To solve the problem of determining the heat developed up to steady state when a tube of radius r is dipped in a liquid with surface tension S, density ρ, and contact angle θ, we will follow these steps:
Step 1: Determine the height of capillary rise
The height h to which the liquid rises in the tube due to capillary action is given by the formula:
h=2Scosθρgr
where:
- S is the surface tension,
- θ is the contact angle,
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- r is the radius of the tube.
Step 2: Calculate the work done by surface tension
The work done by the surface tension when the liquid rises to height h can be calculated as:
Wsurface tension=Force⋅Displacement
The force due to surface tension acting around the circumference of the tube is 2πrS, and the displacement is h. Therefore,
Wsurface tension=2πrS⋅h⋅cosθ
Substituting the expression for h:
Wsurface tension=2πrS⋅(2Scosθρgr)⋅cosθ
This simplifies to:
Wsurface tension=4πS2cos2θρg
Step 3: Calculate the work done by gravity
The work done against gravity when the liquid rises can be calculated using the change in potential energy. The volume of the liquid raised is πr2h, and the mass is given by ρ⋅Volume:
Mass=ρ⋅πr2h
The gravitational potential energy change when the center of mass rises to height h/2 is:
Wgravity=−(ρ⋅πr2h)⋅g⋅(h2)
Substituting for h:
Wgravity=−(ρ⋅πr2⋅2Scosθρgr)⋅g⋅(12⋅2Scosθρgr)
This simplifies to:
Wgravity=−ρ⋅πr2⋅2Scosθρgr⋅g⋅Scosθρgr
After simplification, we find:
Wgravity=−2πS2cos2θρg
Step 4: Calculate total heat developed
The total heat developed Q is the sum of the work done by surface tension and the work done against gravity:
Q=Wsurface tension+Wgravity
Substituting the expressions we derived:
Q=4πS2cos2θρg−2πS2cos2θρg
This simplifies to:
Q=2πS2cos2θρg
Final Answer
Thus, the heat developed up to steady state is:
Q=2πS2cos2θρg
The height h to which the liquid rises in the tube due to capillary action is given by the formula:
h=2Scosθρgr
where:
- S is the surface tension,
- θ is the contact angle,
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- r is the radius of the tube.
The work done by the surface tension when the liquid rises to height h can be calculated as:
Wsurface tension=Force⋅Displacement
The force due to surface tension acting around the circumference of the tube is 2πrS, and the displacement is h. Therefore,
Wsurface tension=2πrS⋅h⋅cosθ
Wsurface tension=2πrS⋅(2Scosθρgr)⋅cosθ
This simplifies to:
Wsurface tension=4πS2cos2θρg
The work done against gravity when the liquid rises can be calculated using the change in potential energy. The volume of the liquid raised is πr2h, and the mass is given by ρ⋅Volume:
Mass=ρ⋅πr2h
The gravitational potential energy change when the center of mass rises to height h/2 is:
Wgravity=−(ρ⋅πr2h)⋅g⋅(h2)
Substituting for h:
Wgravity=−(ρ⋅πr2⋅2Scosθρgr)⋅g⋅(12⋅2Scosθρgr)
This simplifies to:
Wgravity=−ρ⋅πr2⋅2Scosθρgr⋅g⋅Scosθρgr
After simplification, we find:
Wgravity=−2πS2cos2θρg
The total heat developed Q is the sum of the work done by surface tension and the work done against gravity:
Q=Wsurface tension+Wgravity
Substituting the expressions we derived:
Q=4πS2cos2θρg−2πS2cos2θρg
This simplifies to:
Q=2πS2cos2θρg
Thus, the heat developed up to steady state is:
Q=2πS2cos2θρg
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Comprehension
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A capillary tube of radius r is lowered into a liquid of surface tension T and density ρ. Given angle of contact =0∘.
What is the potential energy acquired by the liquid in the capillary?
View SolutionA capillary tube of radius 0.6 mm is dipped vertically in a liquid of surface tension 0.04Nm−1and relative density 0.8 . Calculate the height of capillary rise if the angle of contact is 15∘.
View SolutionChoose the correct option:
A capillary tube of radius r is lowered into a liquid of surface tension T and density ρ. Given angle of contact =0∘.
The work done by surface tension will be
View SolutionThe vertical arms of U - tube have unequal raidus R and r (R>r) . If a liquid of surface tension T and density ρ rests in equillibrium inside the U - tube , find the level difference h between the meniscuses in the two arms .
View SolutionA capillary tube of inside diameter 1 mm is dipped vertically in a liquid of surface tension 63×10−3N/m and density 1262kgm3. Find the height of the capillary rise if the angle of contact is 10∘.
View SolutionA needle of length l and density ρ will float on a liquid of surface tension σ if its radius r is less than or equal to:
View SolutionA capillary tube of radius 1 mm is dipped in water of surface tension 0.073 N m−1. Find the weight of water that rises in the tube. Take angle of contact θ=0∘
Hint : hρg=2Scosθ2
Required wt = πr2hρg
View SolutionA paper disc of radius R from which a hole of radius r is cut out is floating in a liquid of the surface tension S. The force on the disc due to the surface tension is
View SolutionA capillary tube with inner cross-section in the form of a square of side a is dipped vertically in a liquid of density ρ and surface tension ρ which wet the surface of capillary tube with angle of contact θ . The approximate height to which liquid will be raised in the tube is : (Neglect the effect of surface tension at the corners of capillary tube)
View SolutionA tube of fine bore AB is connected to a manomeer M as shown. The stop cock S controls the flow in air. AB is dipped into a liquid whosw surface tension is σ. On opening the stop cock for a while, a bubble is formed at B and the manometer level is recorded, showing a difference h in the levels in the two arms. If ρ be the density of manometer liquid and r the radius of curvature of the bubble, then the surface tension σ of the liquid is given by
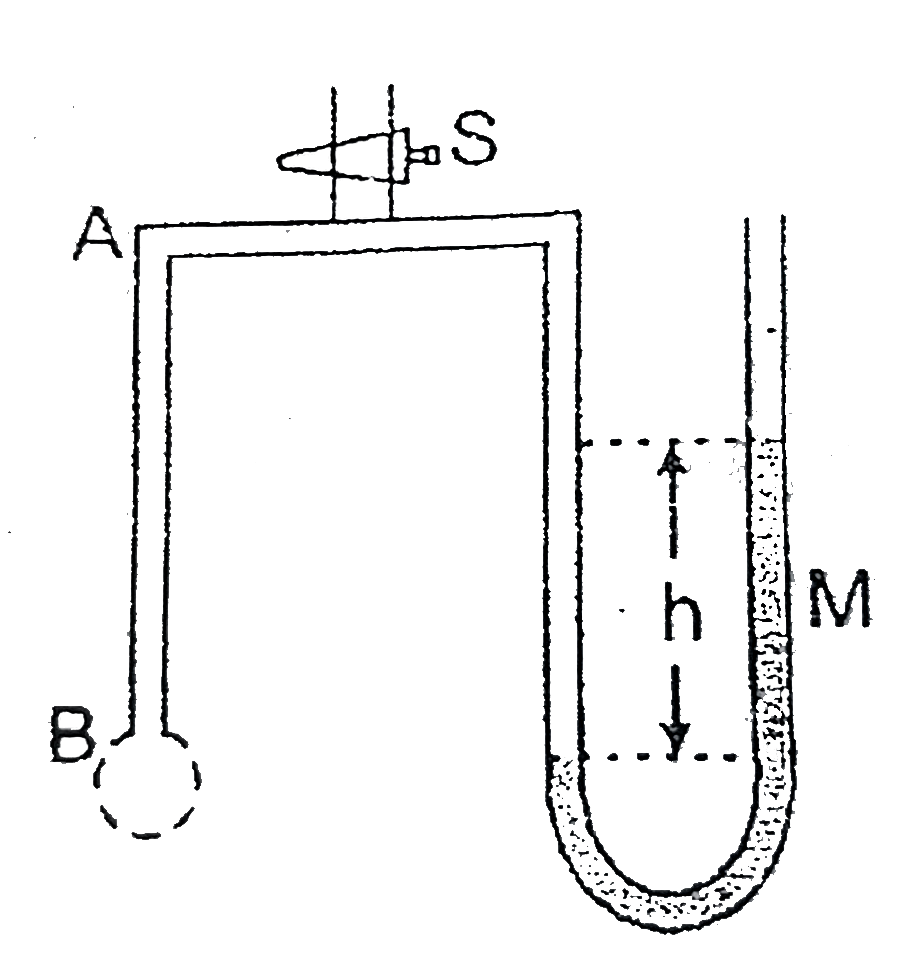
View Solution
