Consider a rectangular plate of dimensions . If this plate is considered to be made up of four rectangles of dimensions and we now remove one out of four rectangles. Find the position where the centre of mass of the remaining system will lie?
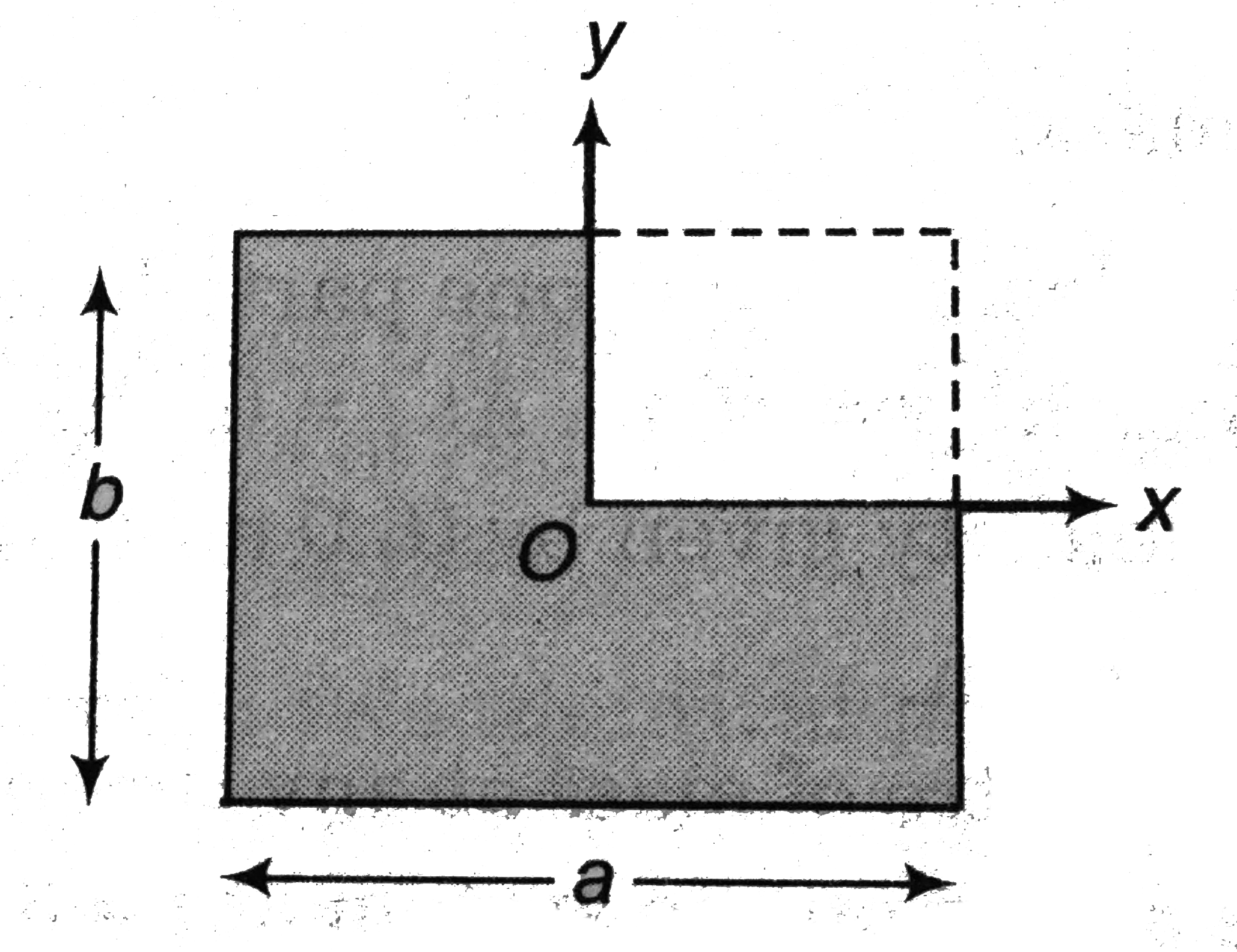
The rectangular plate is shown in the figure of is removed. We can find the and coordinates of the centre of mas of this system, taking origin at the centre of plate. The coordinates of the three remaining rectangles are and . By geometry, masses of these rectangles can be taken as . Now -coordinate of the centre of mass:
and -coordinate of the centre of mass:
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.223videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.324videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples13videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
Similar Questions
A circular plate of uniform thickness has a diameter fo 56cm. A circular portion of diameter 42 cm is removed from one edge of the plate as shown in figure. Find the position of the centre of mass of the remaining portion.
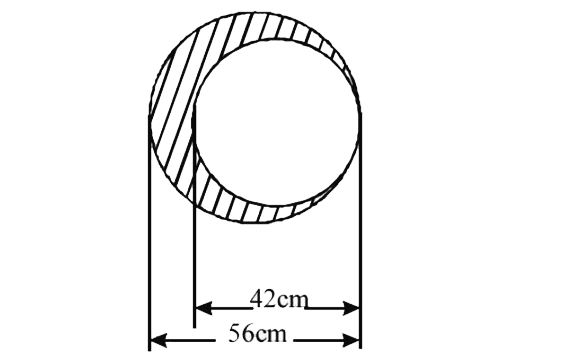 View Solution
View SolutionA square of side 4 cm and of uniform thickness is divided into four equal squares. If one of them is cut off (OECF), then the position of the centre of mass of the remaining portion from O is √2kcm . Find the value of K.
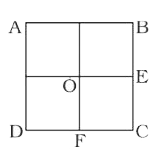 View Solution
View SolutionThere is a rectangular metal plate in which two cavities in the shape of rectangle and circle are made, as shown with dimensions. P and Q are the centres of these cavities. On heating the plate, which of the following quantities increases?
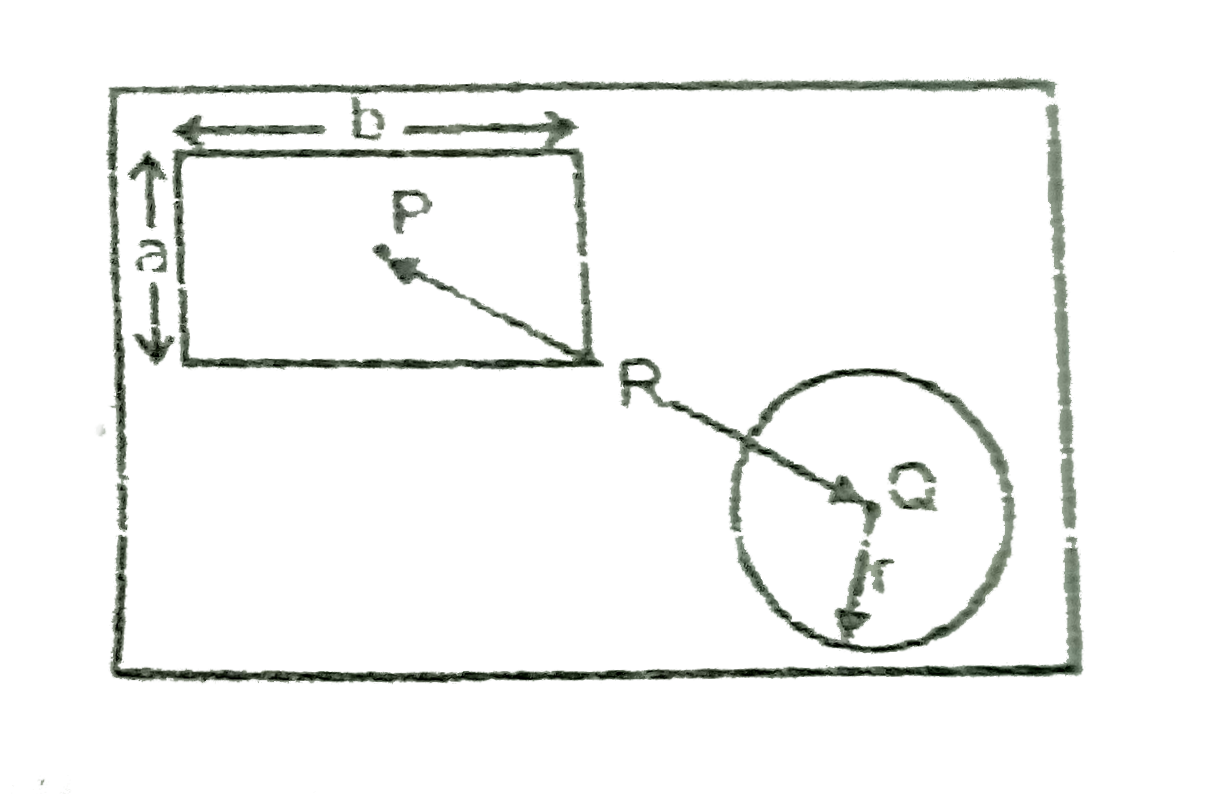 View Solution
View SolutionThe coordinates of the centre of mass of a system of three particles of mass 1g,2g and 3g are (2,2,2). Where should a fourth particle of mass 4 g be positioned so that the centre of mass of the four particle system is at the origin of the three-dimensional coordinate system ?
View SolutionFour point particles of masses 1.0 kg, 1.5 kg, 3.0 kg and 2.5 kg are placed at four corners of a rectangle of sides 4.0 cm and 3.0 cm as shown in the figure. The centre of mass of the system is at a point:
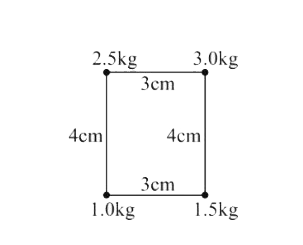 View Solution
View SolutionFour point particles of masses 1.0 kg, 1.5 kg, 3.0 kg and 2.5 kg are placed at four corners of a rectangle of sides 4.0 cm and 3.0 cm as shown in the figure. The centre of mass of the system is at a point:
 View Solution
View SolutionA rectangular plate of mass M and dimension a×b is held in horizontal position by striking n small balls (each of mass m) per unit area per second. The balls are striking in the shaded half region of the plate. The collision of the balls with the plate is elastic. What is v? (Givenn=100,M=3kg,m=0.01kg,b=2m,a=1m,g=10ms2).
 View Solution
View SolutionThere is a rectangular sheet of dimension (2m−1)×(2n−1), (where m>0,n>0) It has been divided into square of unit area by drawing line perpendicular to the sides. Find the number of rectangles having sides of odd unit length.
View Solution_E01_059_Q01.png)
An object of weight W has a uniform rectangular cross section of a x2a and density of 0.25g/cm3 part of it is immersed in water and the rectangle is tilted by 45∘− while one of its corners is just at the water surface find the torque of the buoyancy force to the centre of mass of the object.View SolutionIn the figure shown, find out centre of mass of a system of a uniform circular plate of radius 3R from O in which a hole of radius R is cut whose centre is at 2R distance from the centre of large circular plate
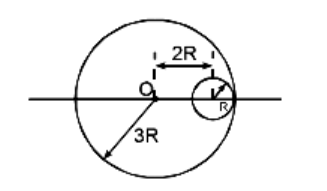 View Solution
View Solution

