Determine the minimum coefficient of friction μmin between as thin homogeneous rod and a floor at which a person can slowly lift the rod the floor without slipage to the vertical position, applying to it a force perpendicular to it.
The correct Answer is:12√2
Consider a general position of the rod as shown in the figure. Let the applied force be F. As the end is moved slowly, some rod has no acceleration. Applying Newton's law in x-direction, f−Fsinθ=0
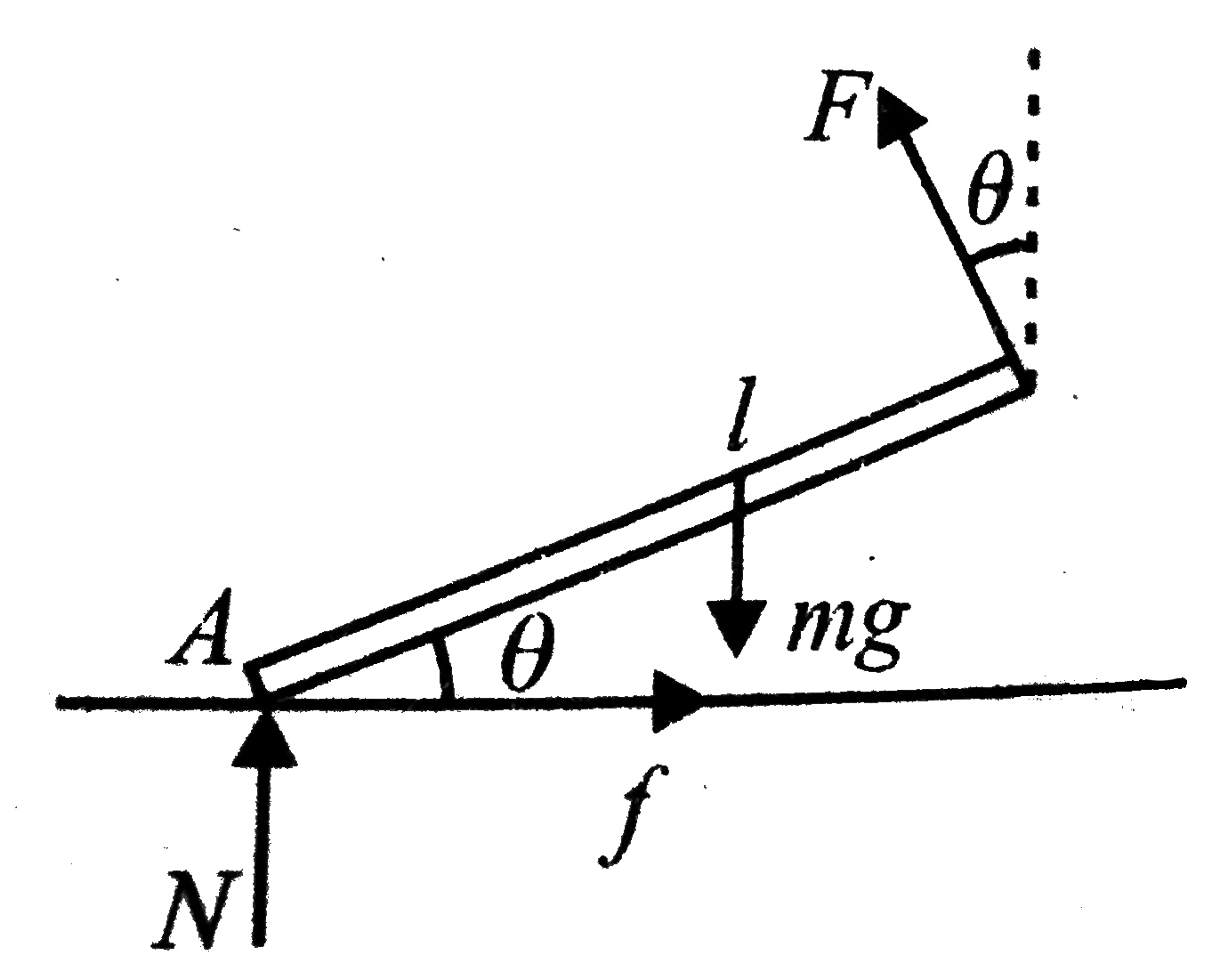
f=Fsinθ
In y-direction Fcosθ+n=mg ......iii
Making τA=0⇒Fl−mg12cosθ=0
F=mgcosθ2.........iii
For no slipping f≤μN⇒μ≥fN
μ≥Fsinθmg−fcosθ⇒μ≥sinθcosθ2−cos2θ
μmin=(sinθcosθ2−cos2θ)=12√2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Single Correct142videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct26videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.413videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
Determine the minimum coefficient of friction between a thin rod and a floor at which a person can slowly lift the rod from the floor, without slipping, to the vertical position applying at its end a force always perpendicular to its length.
View SolutionThe friction coefficient between the board and the floor shown in figure is μ Find the maximum force that the man can exert on the rope so that the board does not slip on the floor
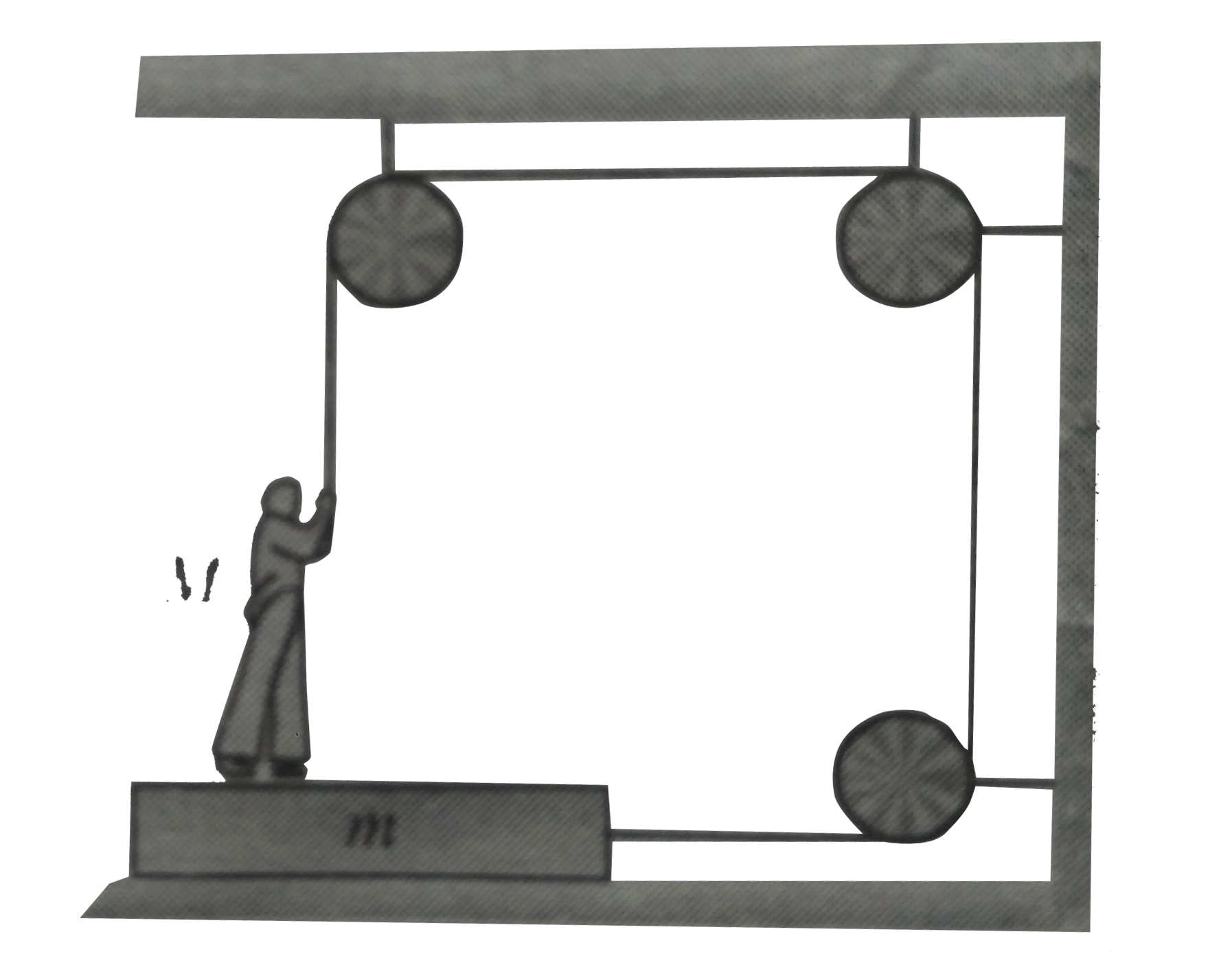 View Solution
View SolutionCoefficient of friction between A and B is μ . The minimum force F with which A will be pushed such that B will not slip down is -
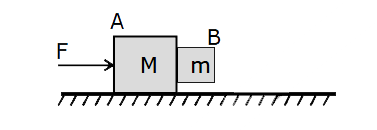 View Solution
View SolutionA block of mass m is stationary on a rough inclined plane making an angle θ with the horizontal. Find the contact force between block and the plane
View SolutionA horizontal force F is applied to a homogeneous rectangular block of mass m, width b and height H. The block moves with constant velocity, the coefficient of friction is μk.
a. What is the greater height h at which the force F can be applied so that the block will slide without tipping over ?
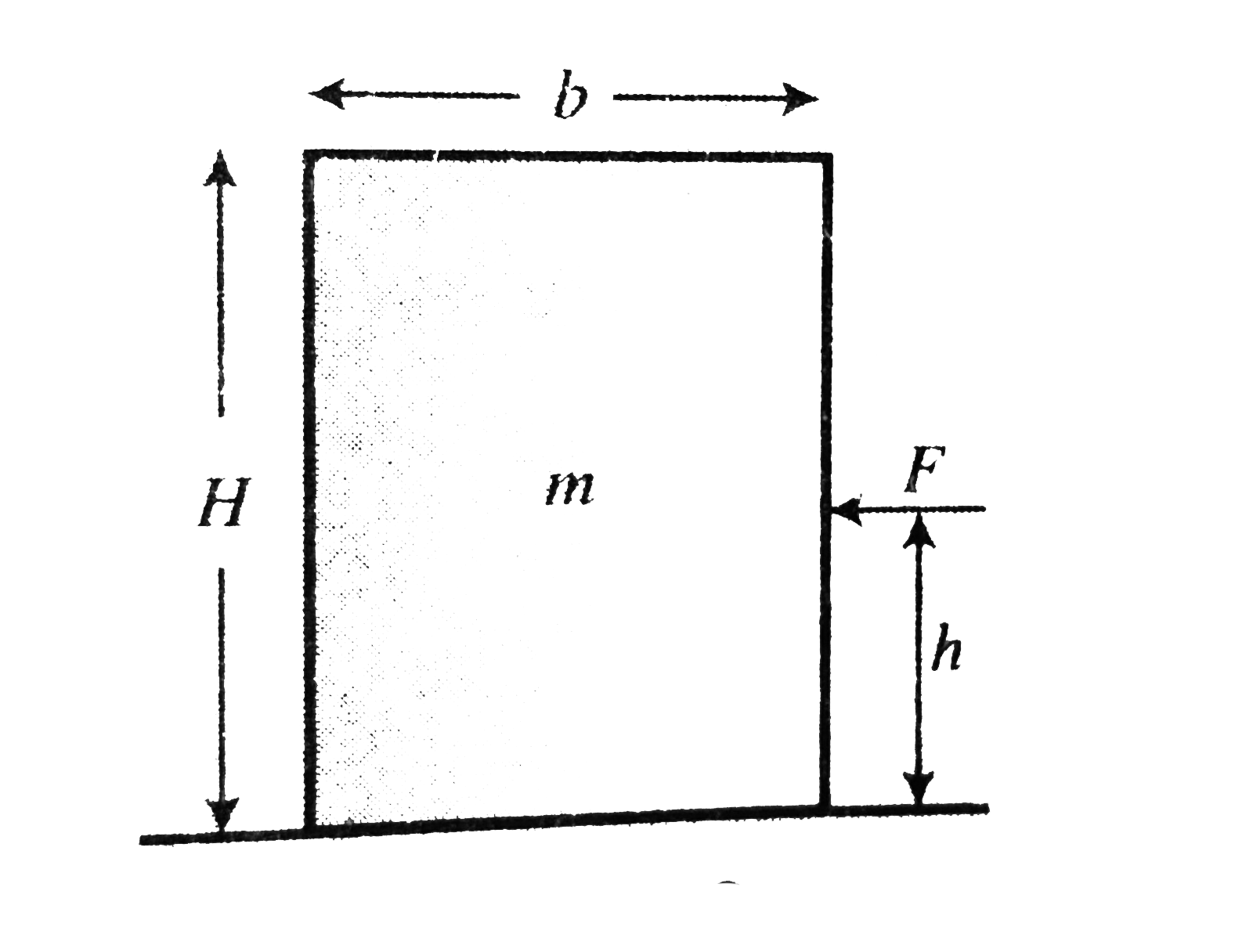
b. Through which point on the bottom face of the block will the resultant of the friction and normal forces act if h=H/2?
c. If the block is at rest and coefficient of static friction is μs what are the various criteria for which sliding or tipping occurs?View SolutionIf the coefficient of friction between M and the inclined surfaces is μ=1/√3 find the minimum mass m of the rod so that the of mass M=10kg remain stationary on the inclined plane
 View Solution
View SolutionThe friction coefficient between the board and the floor shown in figure is μ Find the maximum force that the man can exert on the rope so that the board does not slip on the floor
 View Solution
View SolutionA horizontal uniform rod of mass 'm' has its left end hinged to the fixed incline plane, while its right end rrests on the top of a uniform cylinder of mass 'm' which in turn is at rest on the fixed inclined plane as shown. The coefficient of friction between the cylinder and rod, and between the cylinder and inclined plane, is sufficient to keep the cylinder at rest.
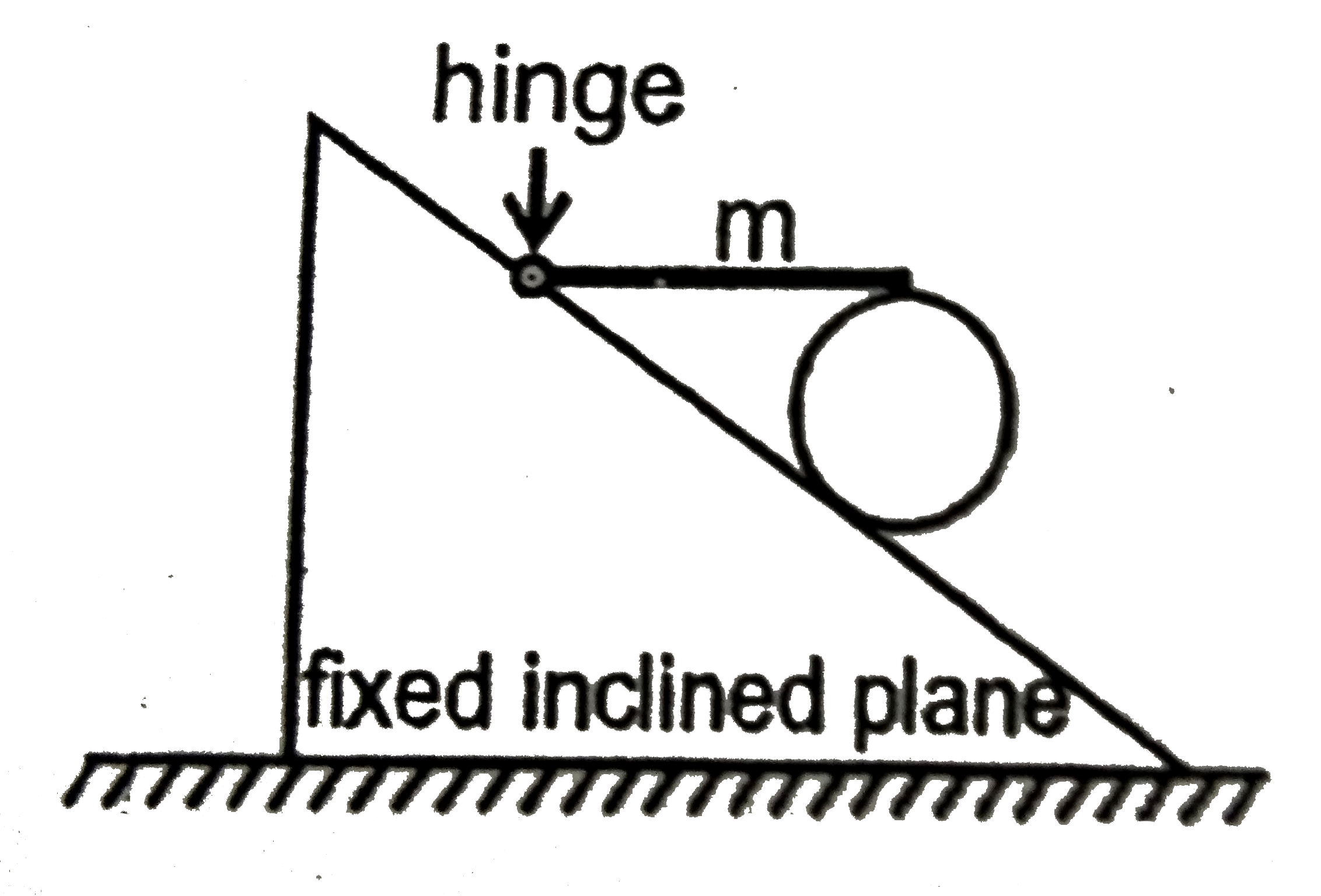
The ratio of magnitude of frictional force on the cylinder due to the rod and the magnitude of frictional force on the cylinder due to the inclined plane is :View Solution
Assertion: A horizontal force F is applied at the centre of solid sphere placed over a plank. The minimum coefficient of friction between plank and sphere required for pure rolling is μ1 when plank is kept at rest ad μ2 when plank can move, then μ2<μ1
Reason: Work done by frictional force on the sphere in both cases is zero.View SolutionA block of mass'm' is resting on the floor of a lift. The coefficient of friction between the block and the floor is μ. When the lift is falling freely, the limiting frictional force between block and surface is
View Solution

