Draw a graph to show the variation of PE, KE and total energy of a simple harmonic oscillator with displacement.
Potential energy (PE) of a simple harmonic oscillator is
=12kx2=12mω2x2 .....(i)
where, k=force constant=mω
When, PE is plotted against displacement x, we will obtain a parabola.
When x=0 PE=0
When x=±A,PE=maximum
=12mω2A2
KE of a simple harmonic harmonic oscillator =12mv2 [∵v=ω√A2−x2]
=12m[ω√A2−x2]2
=12mω2(A2−x2) .....(ii)
This is also parabola, if plot KE against displacement x.
i.e., KE=0atx=±A
and KE=12mω2A2atx=0
Now, total energy of the simple harmonic oscillator =PE+KE [using Eqs. (i) and (ii)]
=12mω2x2+12mω2(A2−x2)
=12mω2x2+12mω2A2−12mω2x2
TE=12mω2A2
Which is constant and does not depend on x.
Plotting under the above guidelines KE, PE and TE versus displacement x-graph as follows
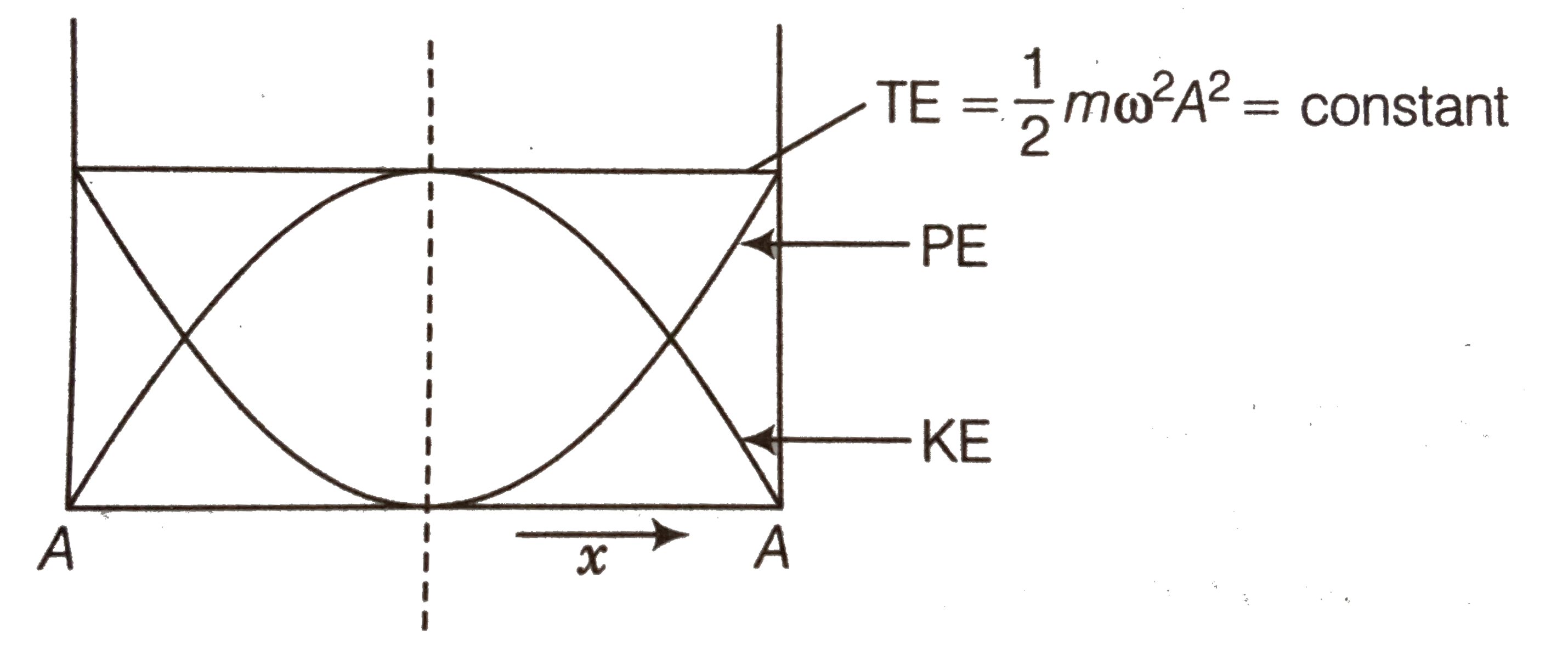
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - Very Short Answer Type Qns14videos
MOTION IN A STRAIGHT LINE
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - Long answer type questions12videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
Similar Questions
Knowledge Check
- Question 1 - Select One
The total energy of a simple harmonic oscillation is proportional to
AamplitudeBsquare of amplitudeCfrequencyDvelocity - Question 2 - Select One
Frequency of variation of kinetic energy of a simple harmonic motion of frequency n is
A2nBnCn2D3n
Draw graphs to show the variation of amplitude with driving frequency of a forced harmonic oscillator under (i) zero damping, (ii) low damping and (iii) high damping
View SolutionDraw a graph showing the variation of decay rate with number of active nuclei.
View SolutionThe graph showing the variation of K.E. or P.E. with displacement of a harmonic oscillator is a parabola, but that of total energy with displacement is a straight line. Why?
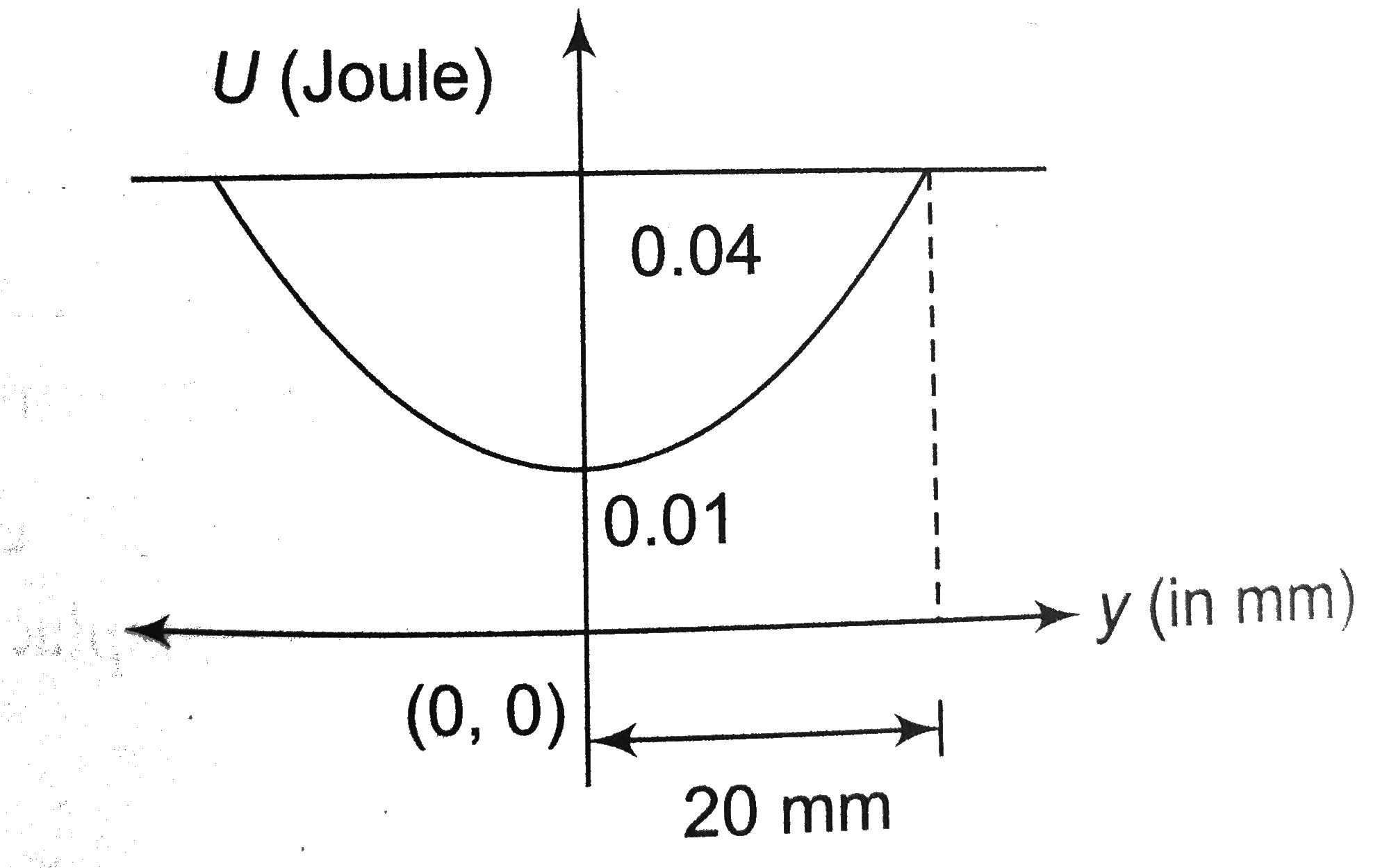
View SolutionThe variation of potential energy of harmonic oscillator is as shown in figure. The spring constant is
 View Solution
View SolutionWhat is the ratio of maximum acceleration to the maximum velocity of a simple harmonic oscillator?
View SolutionWhich of the following statements is/are true for a simple harmonic oscillator ?
View Solution

