During a heavy rain hailstones of average size 1.0 cm in diameter fall with an average speed of 20 m/s. Suppose 2000 hailstones strike every square meter of a roof perpendicularly in one second and assume that the hailstones do not rebound. Calculate the average force exerted by the falling hailstones on the roof. Density of a hailstone is
To solve the problem, we need to calculate the average force exerted by the falling hailstones on the roof. We will do this step by step.
Step 1: Calculate the radius of a hailstone
Given the diameter of a hailstone is 1.0 cm, we can find the radius (r) as follows:
r=diameter2=1.0cm2=0.5cm=0.005m
Hint: Remember to convert the radius from centimeters to meters for consistency in units.
Step 2: Calculate the volume of a hailstone
The volume (V) of a sphere is given by the formula:
V=43πr3
Substituting the radius we found:
V=43π(0.005)3≈5.236×10−7m3
Hint: Use the formula for the volume of a sphere and ensure that the radius is in meters.
Step 3: Calculate the mass of one hailstone
Using the density (ρ) of the hailstone, we can calculate the mass (m) using the formula:
m=ρ×V
Given the density is 900 kg/m³:
m=900kg/m3×5.236×10−7m3≈4.712×10−4kg
Hint: Remember that mass is calculated by multiplying density by volume.
Step 4: Calculate the total mass of 2000 hailstones
Now, we can find the total mass of 2000 hailstones:
Total mass=2000×m=2000×4.712×10−4≈0.9424kg
Hint: Simply multiply the mass of one hailstone by the total number of hailstones.
Step 5: Calculate the change in momentum
The initial velocity (u) of the hailstones is 20 m/s, and they come to rest upon hitting the roof (final velocity, v = 0). The change in momentum (Δp) for the total mass is given by:
Δp=Total mass×(v−u)=0.9424×(0−20)=−18.848kg m/s
Hint: The change in momentum is calculated as the mass multiplied by the change in velocity.
Step 6: Calculate the average force exerted
The average force (F) can be calculated using the formula:
F=ΔpΔt
Where Δt is the time interval (1 second):
F=−18.848kg m/s1s≈−18.848N
Hint: Force is the rate of change of momentum over time.
Step 7: Calculate the total force on the roof
Since the hailstones strike every square meter of a 10m x 10m roof, the total area (A) is:
A=10m×10m=100m2
Thus, the total force exerted on the roof is:
Ftotal=F×A=18.848N/m2×100m2=1884.8N
Hint: Multiply the force per unit area by the total area to find the total force.
Final Answer
The average force exerted by the falling hailstones on the roof is approximately 1884.8 N.
Given the diameter of a hailstone is 1.0 cm, we can find the radius (r) as follows:
r=diameter2=1.0cm2=0.5cm=0.005m
The volume (V) of a sphere is given by the formula:
V=43πr3
Substituting the radius we found:
V=43π(0.005)3≈5.236×10−7m3
Using the density (ρ) of the hailstone, we can calculate the mass (m) using the formula:
m=ρ×V
Given the density is 900 kg/m³:
m=900kg/m3×5.236×10−7m3≈4.712×10−4kg
Now, we can find the total mass of 2000 hailstones:
Total mass=2000×m=2000×4.712×10−4≈0.9424kg
The initial velocity (u) of the hailstones is 20 m/s, and they come to rest upon hitting the roof (final velocity, v = 0). The change in momentum (Δp) for the total mass is given by:
Δp=Total mass×(v−u)=0.9424×(0−20)=−18.848kg m/s
The average force (F) can be calculated using the formula:
F=ΔpΔt
Where Δt is the time interval (1 second):
F=−18.848kg m/s1s≈−18.848N
Since the hailstones strike every square meter of a 10m x 10m roof, the total area (A) is:
A=10m×10m=100m2
Thus, the total force exerted on the roof is:
Ftotal=F×A=18.848N/m2×100m2=1884.8N
The average force exerted by the falling hailstones on the roof is approximately 1884.8 N.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Question For short Answer
25 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -2
11 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -1
18 Videos
- CALORIMETRYBOOK - HC VERMA ENGLISHCHAPTER - CALORIMETRYEXERCISE - Short answer
9 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
Similar Questions
A 3.00kg steel hall strikes the wall with a speed of 10.0m/s at an angle of 60∘ with the surface. It bounces off with the same speed and angle. If the ball is in contact with the wall for 0.200s, what is the average force exerted by the wall on the ball?
View SolutionA 1.5 kg hammer moving with a velocity of 10 m/s strikes a nail for 0.005 s. The average force exerted on the nail is
View Solution
Knowledge Check
A 3 kg ball strikes a heavy rigid wall with a speed of 10 m/s at an angle of 60∘. It gets reflected with the same speed and angle as shown in Fig. 15.3.11. It the ball is in contact with the wal for 0.20 s, what is the average force exerted on the ball by the wall ?
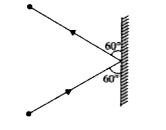 AzeroB150 NC150 √3 ND300 N
AzeroB150 NC150 √3 ND300 N
A 3-kg steel ball strikes a wall with a speed of 10.0ms−1 at an angle of 60.0∘ with the surfaces of the wall. The ball bounces off with the same speed and same angle. If the ball was in contact with the wall for 0.2s, find the average force exerted by the wall on the ball.
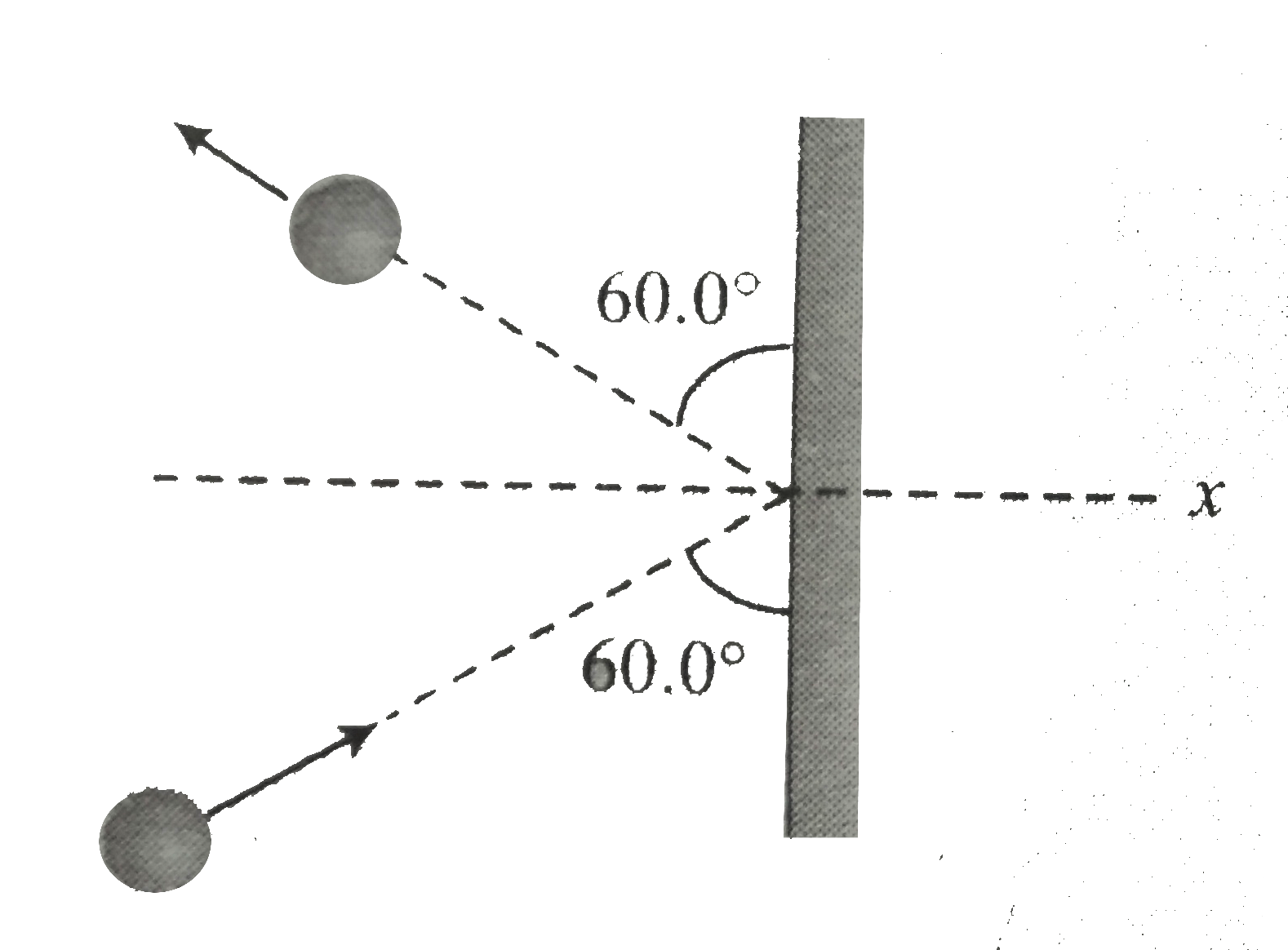
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA current of 1.0 A exists in a copper wore of cross-section 1.0mm2. Assuming one free electron per atom calculate the drift speed of the free electrons in the wire. The density of copper is 9000kgm−3.
View SolutionA ball weighing 10g hits a hard surface vertically with a speed of 5m/s and rebounds with the same speed The ball remains in contact with the surface speed The ball remains in contact with the surface for 0.01s The average force exerted by the surface on the ball is .
View SolutionHailstones falling vertically with a speed of 10ms−1, hit the wind screen (wind screen makes an angle 30∘ with the horizontal) of a moving car and rebound elastically. Find the velocity of the car if the driver finds the hailstones rebound vertically after striking.
 .
.
View SolutionFigure shown a hydraulic press with the larger piston if diameter 35 cm at a height of 1.5 m relative to the smaller piston of diameter 10cm. The mass on the smaller piston is 20 kg. What is the force exerted on the load by the larger piston? The density of oil in the press is 750kh/m3 . (Take g=9.8m/s2)
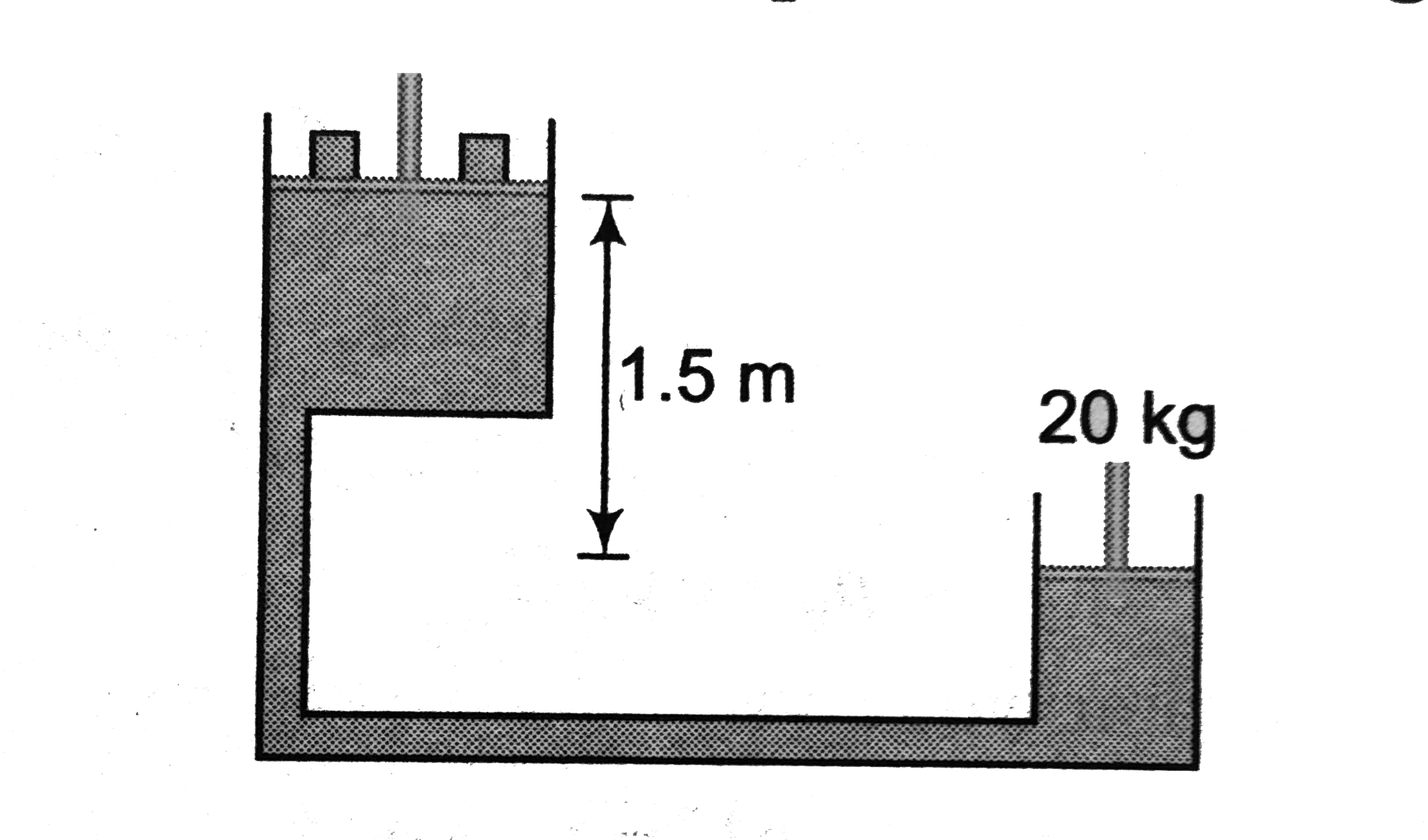 .
.
View Solution
