consider a head on collision between two particles of masses m1andm2. The initial speeds of the particles are u1andu2 in the same direction. The collision starts at t=0 and the particles interact for a time interval △t. during the collision the speed of the first particle varies as
v(t)=u1+t/ t(v1−u1) Find the speed of the second particle as as function of time during the collision.
To find the speed of the second particle as a function of time during the collision, we will apply the principle of conservation of linear momentum. Here are the steps to derive the expression for the speed of the second particle, v2(t):
1. Identify the Initial Momentum:
The initial momentum of the system before the collision is given by:
Pinitial=m1u1+m2u2
2. Identify the Final Momentum:
During the collision, the speed of the first particle is given by:
v(t)=u1+tΔt(v1−u1)
Let v2(t) be the speed of the second particle at time t. The final momentum after the collision can be expressed as:
Pfinal=m1v(t)+m2v2(t)
3. Apply Conservation of Linear Momentum:
According to the conservation of momentum, the initial momentum equals the final momentum:
m1u1+m2u2=m1v(t)+m2v2(t)
4. Substitute for v(t):
Substitute the expression for v(t) into the momentum equation:
m1u1+m2u2=m1(u1+tΔt(v1−u1))+m2v2(t)
5. Simplify the Equation:
Expanding the equation gives:
m1u1+m2u2=m1u1+m1tΔt(v1−u1)+m2v2(t)
Cancel m1u1 from both sides:
m2u2=m1tΔt(v1−u1)+m2v2(t)
6. Rearranging for v2(t):
Rearranging the equation to isolate v2(t):
m2v2(t)=m2u2−m1tΔt(v1−u1)
Divide by m2:
v2(t)=u2−m1tm2Δt(v1−u1)
7. Final Expression:
Thus, the speed of the second particle as a function of time during the collision is:
v2(t)=u2−m1tm2Δt(v1−u1)
The initial momentum of the system before the collision is given by:
Pinitial=m1u1+m2u2
During the collision, the speed of the first particle is given by:
v(t)=u1+tΔt(v1−u1)
Let v2(t) be the speed of the second particle at time t. The final momentum after the collision can be expressed as:
Pfinal=m1v(t)+m2v2(t)
According to the conservation of momentum, the initial momentum equals the final momentum:
m1u1+m2u2=m1v(t)+m2v2(t)
Substitute the expression for v(t) into the momentum equation:
m1u1+m2u2=m1(u1+tΔt(v1−u1))+m2v2(t)
Expanding the equation gives:
m1u1+m2u2=m1u1+m1tΔt(v1−u1)+m2v2(t)
Cancel m1u1 from both sides:
m2u2=m1tΔt(v1−u1)+m2v2(t)
Rearranging the equation to isolate v2(t):
m2v2(t)=m2u2−m1tΔt(v1−u1)
Divide by m2:
v2(t)=u2−m1tm2Δt(v1−u1)
Thus, the speed of the second particle as a function of time during the collision is:
v2(t)=u2−m1tm2Δt(v1−u1)
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Question For short Answer
25 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -2
11 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -1
18 Videos
- CALORIMETRYBOOK - HC VERMA ENGLISHCHAPTER - CALORIMETRYEXERCISE - Short answer
9 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
Similar Questions
A particle of mass m moving in the x direction with speed 2v is hit by another particle of mass 2m moving in they y direction with speed v. If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to :
View SolutionTwo particle of masses m and 2m are coliding elastically as given in figure. If V1 and V2 speed of particle just after collision then
_2019_CBT5_E01_051_Q01.png)
View SolutionTwo particles of masses m1 and m2 and velocities u1 and αu1(α≠0) make an elastic head on collision. If the initial kinetic energies of the two particles are equal and m1 comes to rest after collision, then
View SolutionA particle is kept at rest at the origin. A constant force →F starts acting on it at t=0. Find the speed of the particle at time t.
View SolutionTwo particles of masses m1,m2 move with initial velocities u1 and u2. On collision, one of the particles get excited to higher level, after absording enegry. If final velocities of particles be v1 and v2 then we must have
View SolutionTwo particles of masses m1,m2 move with initial velocities u1andu2. On collision, one of the particles get excited to higher level, after absorbing energy ε. If final velocities of particles be v1 andv2, then we must have
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 3 m at rest. Loss of KE of system in the collision is
View SolutionTwo stones are projected from level ground. Trajectory of two stones are shown in figure. Both stones have same maximum heights above level ground as shown. Let T1 and T2 be their time of flights and u1 and u2 be their speeds of projection respectively (neglect air resistance). then
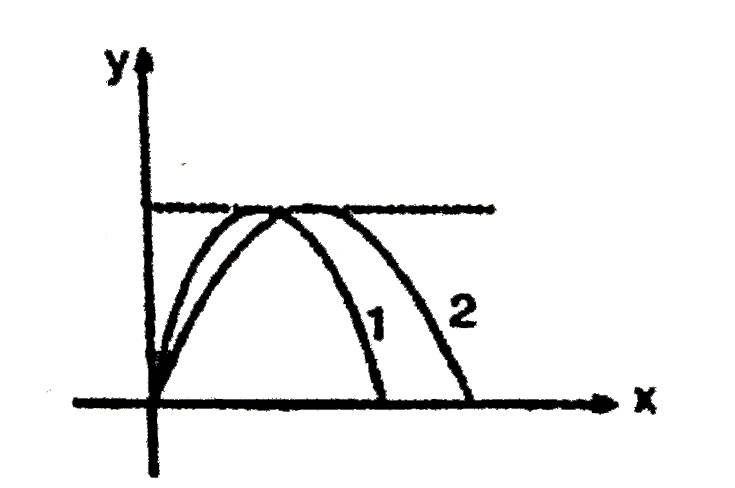
View SolutionForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionFind the loss of KE of the two particles of mass m1=3kg&m2=6kg, moving towards each other with speed u1=5m/s,u2=10m/s respectively. The coefficient of restitution of collision of the particles is e = 0.5.
View Solution
