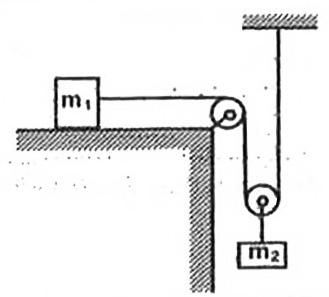
Find the acceleration blocks in fig. The pulley and the string are massless.

Let A be the acceleration of block M and pulley, and a be the acceleration of m.
Let x, X be the coordinates of m and pulley as shown.
Length of string passing over the pulley is
L=X+(X−x)⇒L=2X−x
Differentiating twice with respect to time.
0=2A−a⇒a=2A ...(i)
From force diagrams of m and M, we have
T=ma ...(ii)
and F−2T=MA ...(iii)
Comparing (i), (ii) and (iii), we get A=FM+4m and a=2F(M+4m)

Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.4
13 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Subjective
15 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.2
35 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
Knowledge Check
Find the acceleration of the block of mass m. Assume pulleys are massless and frictionless.
 Ag/3B2g3Cg2DNone
Ag/3B2g3Cg2DNoneIn the diagram shown in figure, both pulleys and strings are massless. The acceleration of 2 kg block is
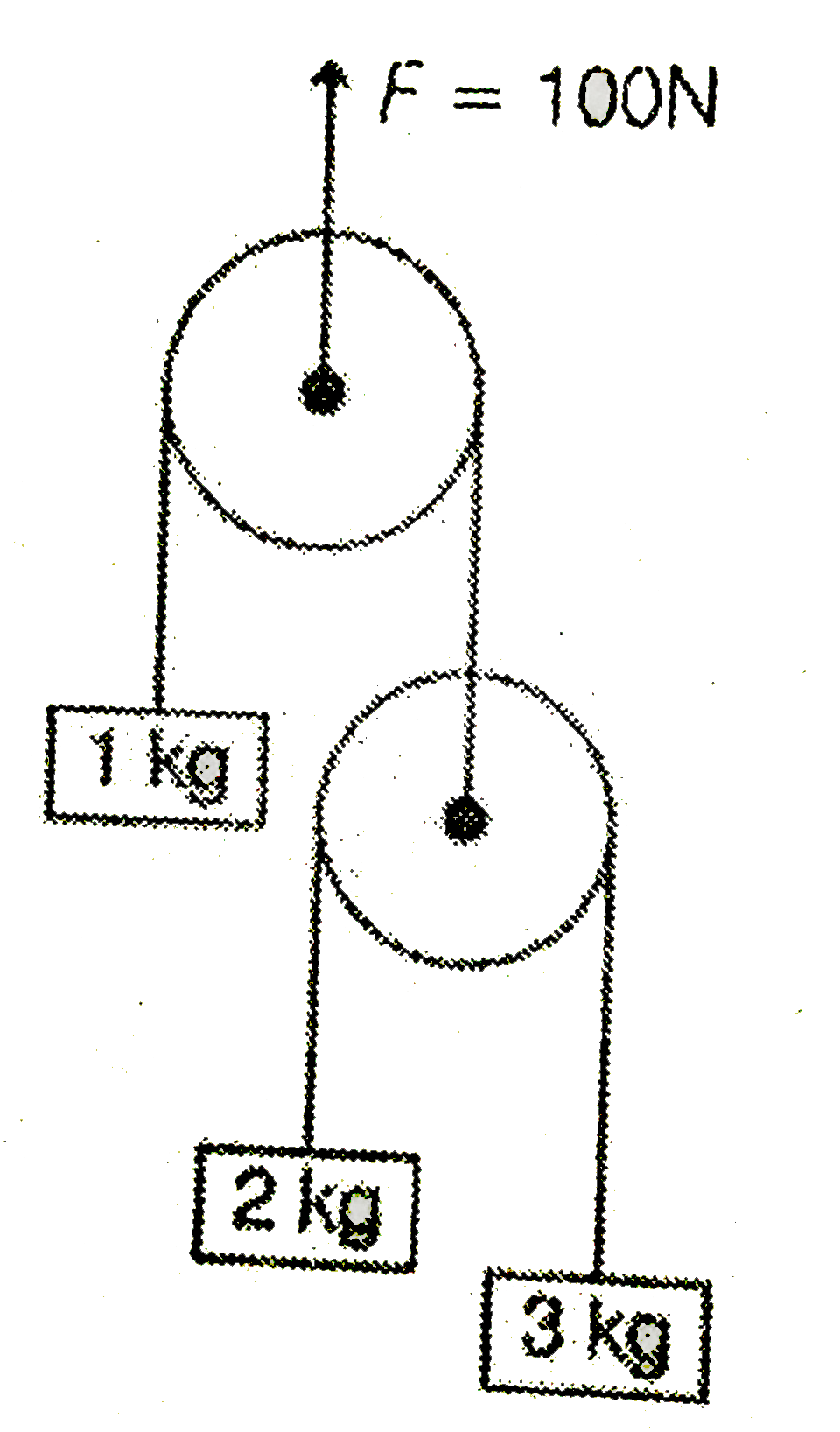 A2.5m/s2(↑⏐)B5m/s2(↑⏐)C7.5m/s2(⏐↓)D10m/s2(↑⏐)
A2.5m/s2(↑⏐)B5m/s2(↑⏐)C7.5m/s2(⏐↓)D10m/s2(↑⏐)The acceleration of the block (A) and (B) respectively in situation shown in the figure is: (pulleys and string are massless).
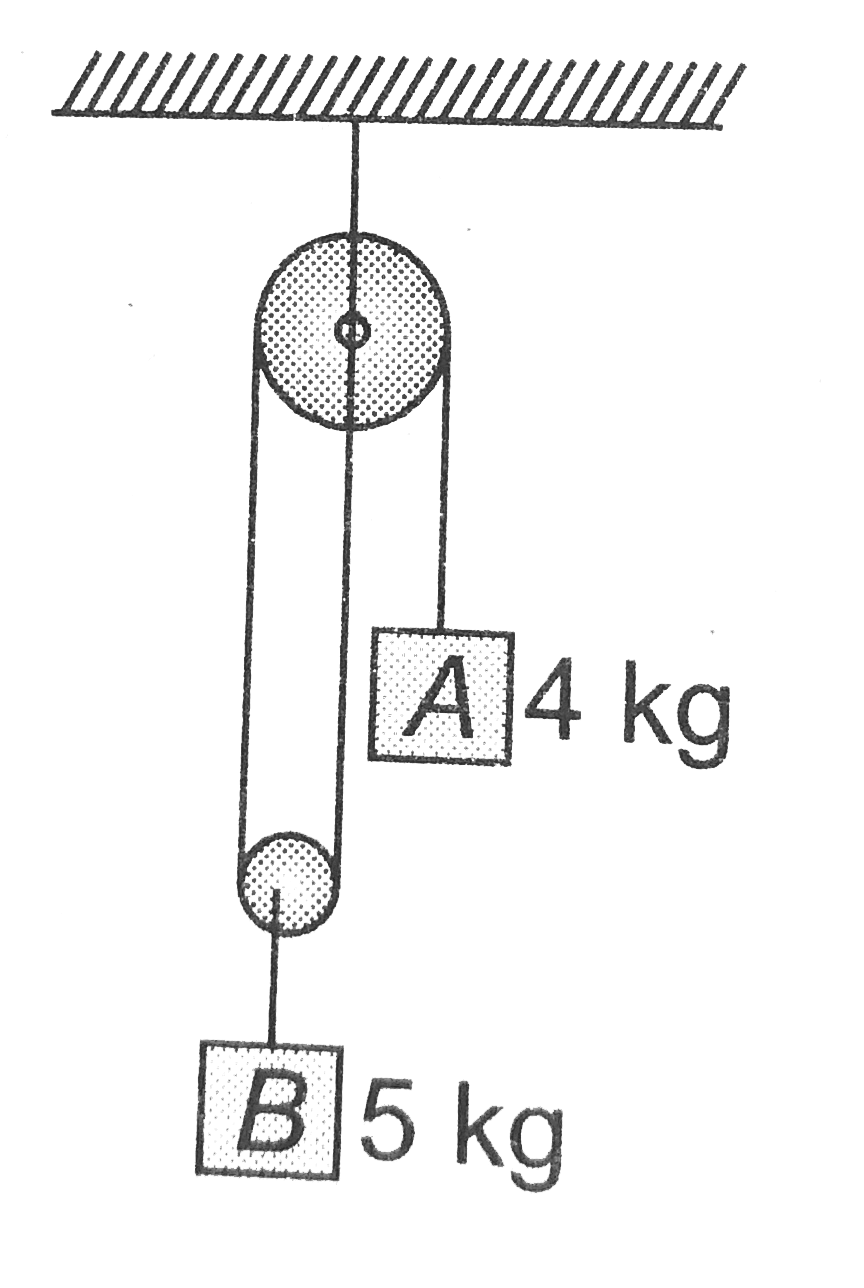 A2g7 downward, g7 upwardB2g3 downward, g3 upwardC1013g downward, 5g13 upwardDnone of these
A2g7 downward, g7 upwardB2g3 downward, g3 upwardC1013g downward, 5g13 upwardDnone of these
Two blocks are connected by an inextensible light string, the string is passing over smooth, light pulley as shown. Find the acceleration of blocks and tension in the string, reaction in pulley.

View Solutionfind the acceleration of the block of mass M in the situation shown in figure. All the surfaces are frictionless and the pulleys and the string are light.

View SolutionIn the figure given below, with what acceleration does the block of mass m will move? (Pulley and strings are massless and frictionless)
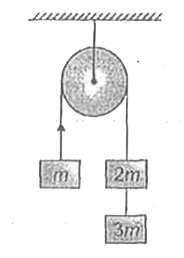
View SolutionIn the system shown all the surfaces are frictionless while pulley and string are massless. The mass of block A is 2m and that of block B is m. The acceleration of block B immediately after system is released from rest is
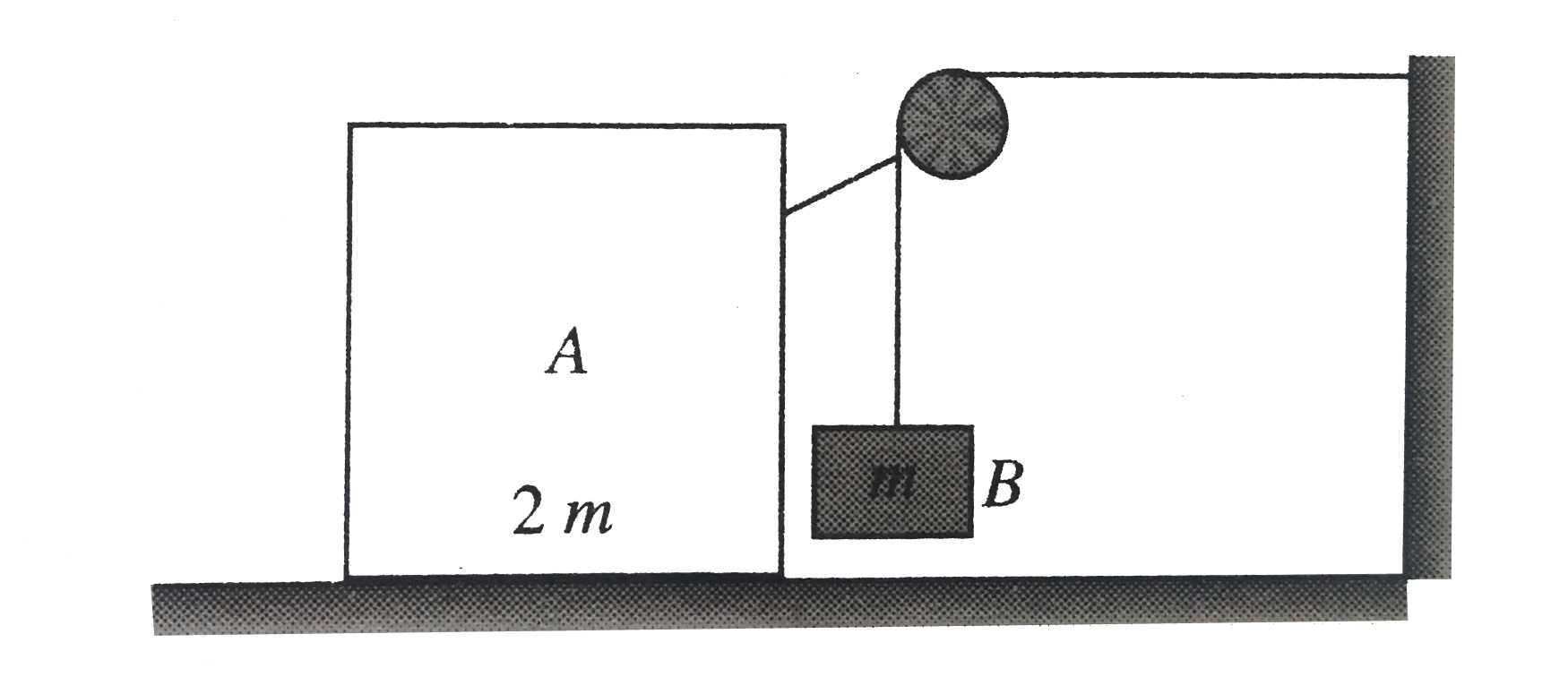
View SolutionIn the system shown in fig the pulley is frictionless and the string massless if m1=m2 thrust on the pulley will be:
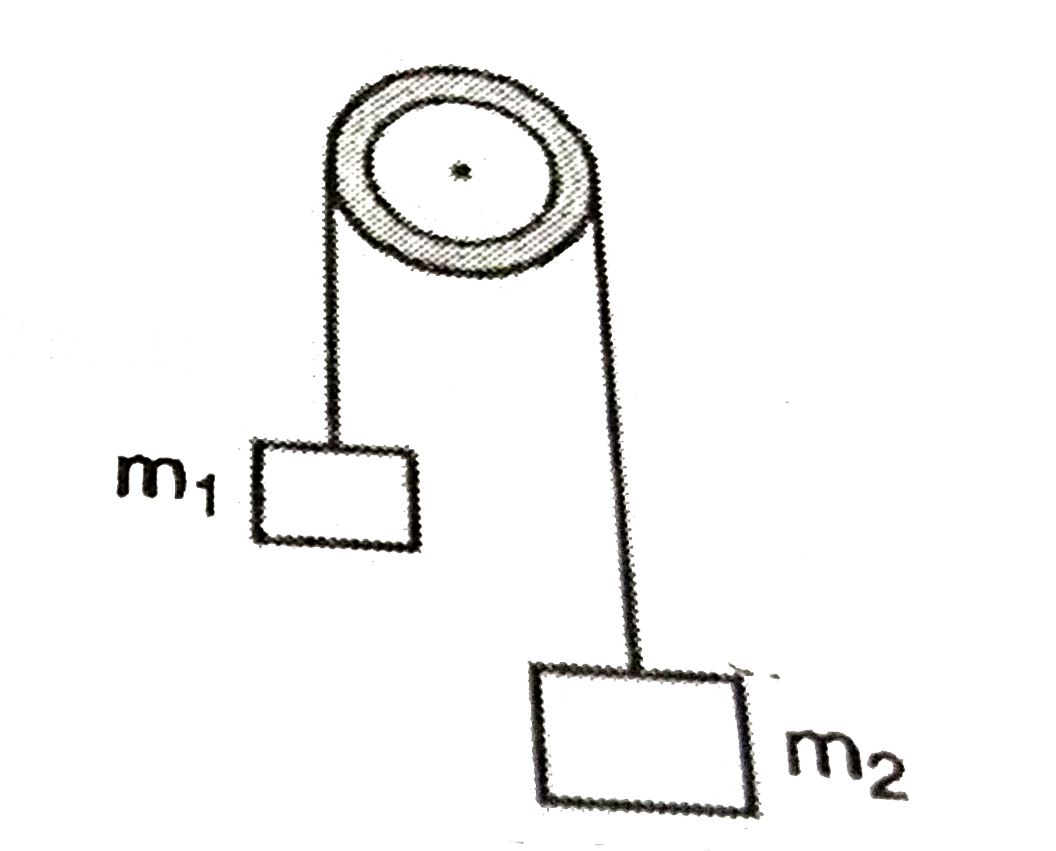
View Solution