Find the period of small oscillations of a mathematical pendulum of length $l$ if its point of suspension $O$ moves relative to the Earth's surface in an arbitrary directio with a constant acceleration $w$ (figure). Calculate that period if $l = 21 c m, w = g / 2,$ and the angle between the vectors $w$ and $g$ equals $b e a t = 120^{\circ}$ .

Video Solution

Text Solution

Verified by Experts

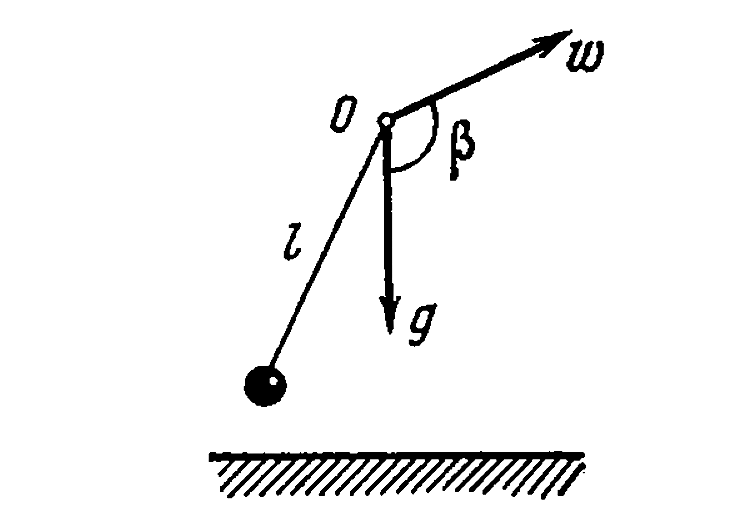
In the frame of point of suspension the mathematical pendulum of mass $m$ (say) will oscillate. In this fracem, the body $m$ will experience the inertial force $m (- \to w)$ in addition to the real forces during its oscillations. Therefore in equilibrium position $m$ is deviated by some angle say $α$ . In equilibrium position.
$T_{0} cos α = m g + m w cos (π - β)$ and $T_{0} sin α = m w sin (π - β)$
So, from these two two Eqns
$tan α = \frac{g - w cos β}{w sin β}$
and $cos α = \sqrt{\frac{m^{2} w^{2} {sin}^{2} β + {(m g - m w cos β)}^{2}}{m g - m w cos β}} ... . (1)$
Let us displace the bob $m$ from its equilibrium position by some small angle and then release it. Now locate the ball at an angular position $(α + θ)$ from vertical as shown in the figure.
From the Eqn. : $N_{0 z} = I β_{z}$
$= - m g l sin (α + θ) - m w cos (π - β) l sin (α = θ) + m w sin (π - β) l cos (α + θ) = m l^{2} .. θ$
or , $- g (sin α cos θ + cos α sin θ) - w cos (π - β) (sin α cos θ + cos α {sin θ}_{+} w sin β (cos α cos θ - sin α sin θ)$
$= l .. θ$
But for small
$θ, sin θ ≅ θ cos θ ≅ 1$
So, $- g (sin α + cos α θ) - w cos (π - β) (sin α + cos α θ) = w sin β (cos α - sin α θ)$
$= l .. θ$
or $(tan α + θ) (w cos β - g) + w sin β (1 - tan α θ) = \frac{l}{cos α} .. θ ... (2)$
Solving Eqns $(1)$ and $(2)$ simultaneously we get
$- (g^{2} - 2 w g cos β + w^{2}) θ = l \sqrt{g^{2} + w^{2} - 2 w g cos β} .. θ$
Thus $.. θ = - \frac{| \to g - \to w |}{l} θ$
Hence the sought time period $T = \frac{2 π}{ω_{0}} = 2 π \sqrt{\frac{l}{| \to g - \to w |}}$

Updated on:21/07/2023

The period of small oscillations of a simple pendulum of length $l$ if its point of suspension $O$ moves $a$ with a constant acceleration $α = α_{1} \land i - α_{2} \land j$ with respect to earth is ( $\land i$ and $\land j$ are unit vectors in horizontal in horizontal and vertically upward directions respectively)
A $T = 2 π \sqrt{\frac{l}{{{(g - α_{2})}_{2}^{2} + α_{1}^{2}}^{1 / 2}}}$
B $T = 2 π \sqrt{\frac{l}{{{(g - α_{1})}_{1}^{2} + α_{2}^{2}}^{1 / 2}}}$
C $T = 2 π \sqrt{\frac{l}{g}}$
D $T = 2 π \sqrt{\frac{l}{{(g^{2} + α_{1}^{2})}^{1 / 2}}}$
The period of small oscillations of a simple pendulum of length $l$ if its point of suspension $O$ moves $a$ with a constant acceleration $α = α_{1} \land i - α_{2} \land j$ with respect to earth is ( $\land i$ and $\land j$ are unit vectors in horizontal in horizontal and vertically upward directions respectively)
A $T = 2 π \sqrt{\frac{l}{{{(g - α_{2})}_{2}^{2} + α_{1}^{2}}^{1 / 2}}}$
B $T = 2 π \sqrt{\frac{l}{{{(g - α_{1})}_{1}^{2} + α_{2}^{2}}^{1 / 2}}}$
C $T = 2 π \sqrt{\frac{l}{g}}$
D $T = 2 π \sqrt{\frac{l}{{(g^{2} + α_{1}^{2})}^{1 / 2}}}$
The period of a conical pendulum in terms of its length (l) , semivertical angle $(θ)$ and acceleration due to gravity (g) is
A $\frac{1}{2 π} \sqrt{\frac{l cos θ}{g}}$
B $\frac{1}{2 π} \sqrt{\frac{l sin θ}{g}}$
C $2 π \sqrt{\frac{l cos θ}{g}}$
D $4 π \sqrt{\frac{l tan θ}{g}}$