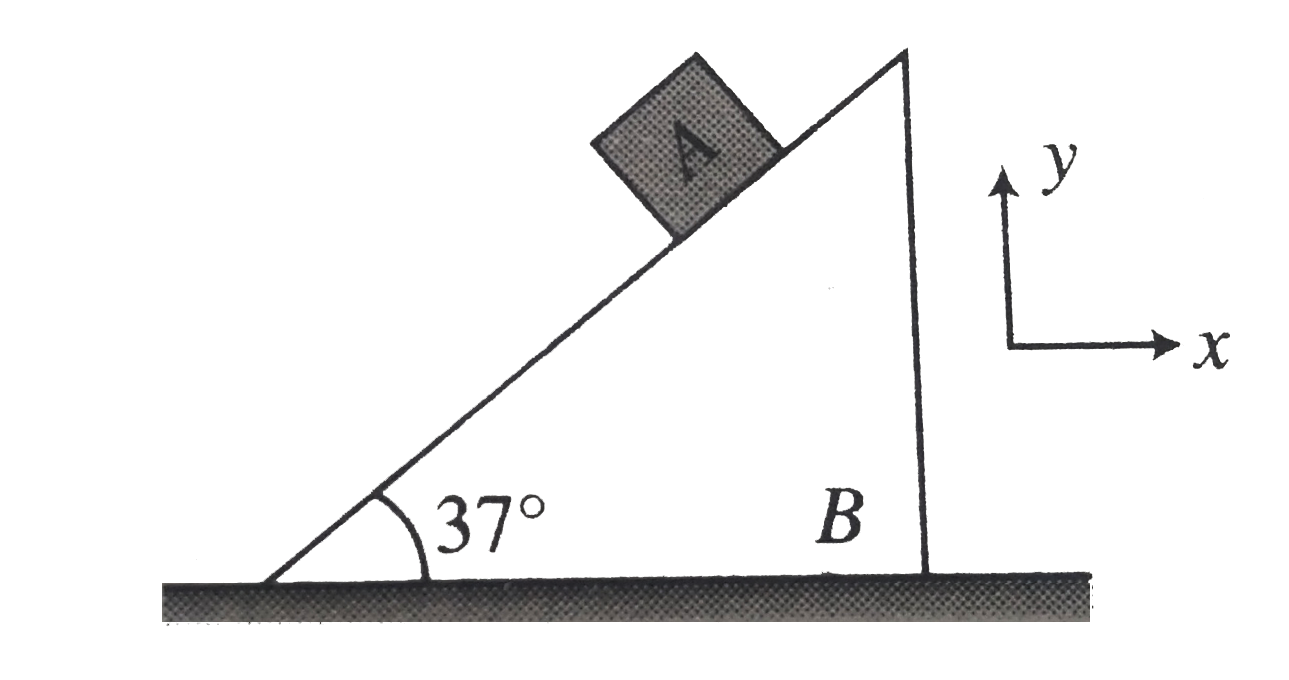
In fig, the acceleration of A is →aA=15ˆi+15ˆj. Then the acceleration of B is (A remains in constact with B)
A
6ˆi
B
−15ˆi
C
−10ˆi
D
−5ˆi
Video Solution
Text Solution
The correct Answer is:D
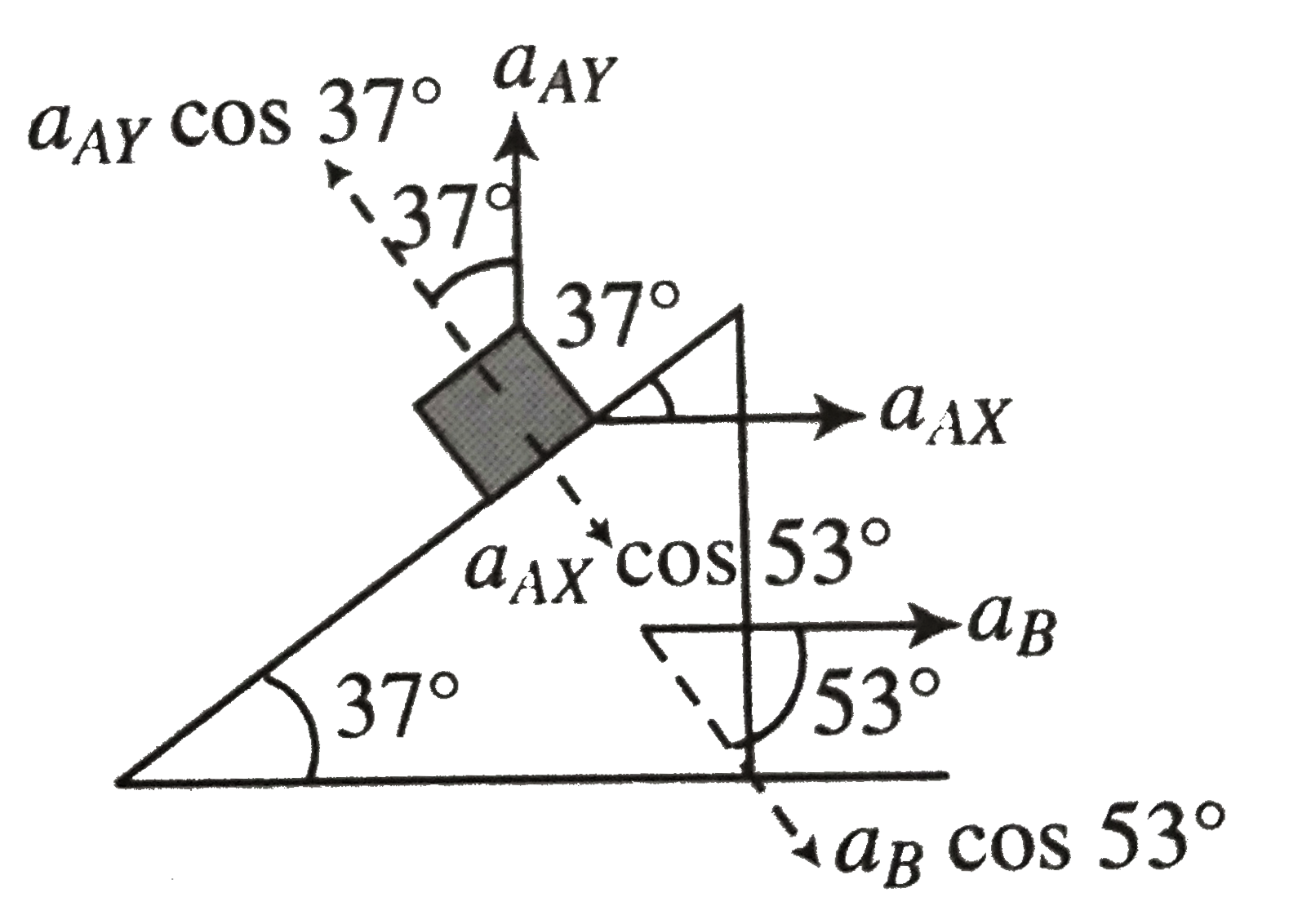
From constraint the acceleration of both block and wedge should be same in a direction perpendicular to the inclined plane as shown in fig.
(aA)⊥=(aB)⊥
aAX=15,aAY=15
aAXcos53∘−aAYcos37∘=aBcos53∘
or aB=−5ms−1or→aB=−5ˆi
|
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Multiple Correct
11 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Assertion-reasoning
15 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Subjective
15 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
Knowledge Check
In the figure shown the acceleration of A is, →aA=15ˆi+15ˆj then the acceleration of B is (A remains in contact in B)
 A6ˆiB−15ˆiC−10ˆiD−5ˆi
A6ˆiB−15ˆiC−10ˆiD−5ˆiIn the figure shown the velocity of A with respect to ground is (20ˆi+15ˆj)m/s, then what will be the magnitude of velocity of the wedge 'B' with respect to ground frame. (A remains in contact with B)
 A12m/sB25m/sC20m/sDzero
A12m/sB25m/sC20m/sDzeroIf →A=3ˆi−4ˆj and →B=2ˆi+16ˆj then the magnitude and direction of →A+→B will be
A5,tan−1(12/5)B10,tan−1(5/12)C13,tan−1(12/5)D12,tan−1(5/12)
A charged particle has acceleration →a=2ˆi+xˆj in a megnetic field →B=−3ˆi+2ˆj−4ˆk. Find the value of x.
View SolutionIf →A=(ˆi+2ˆj+5ˆk) and →B=(ˆi+5ˆj+7ˆk), then the projection of →A on →B would be
View SolutionIf →a=ˆi−ˆj and →b=−ˆj+ˆk find the projection of →aon →b.
View SolutionIf →a=4ˆi+6ˆj and →b=3ˆj+ˆk, then the component of →a along →b is
View SolutionIf the position vectors of the vertices A, B and C of ΔABC are respectively →0,−20ˆi+15ˆjand36ˆi+15ˆj, then find the position vector of the incentre of the triangle.
View Solution
