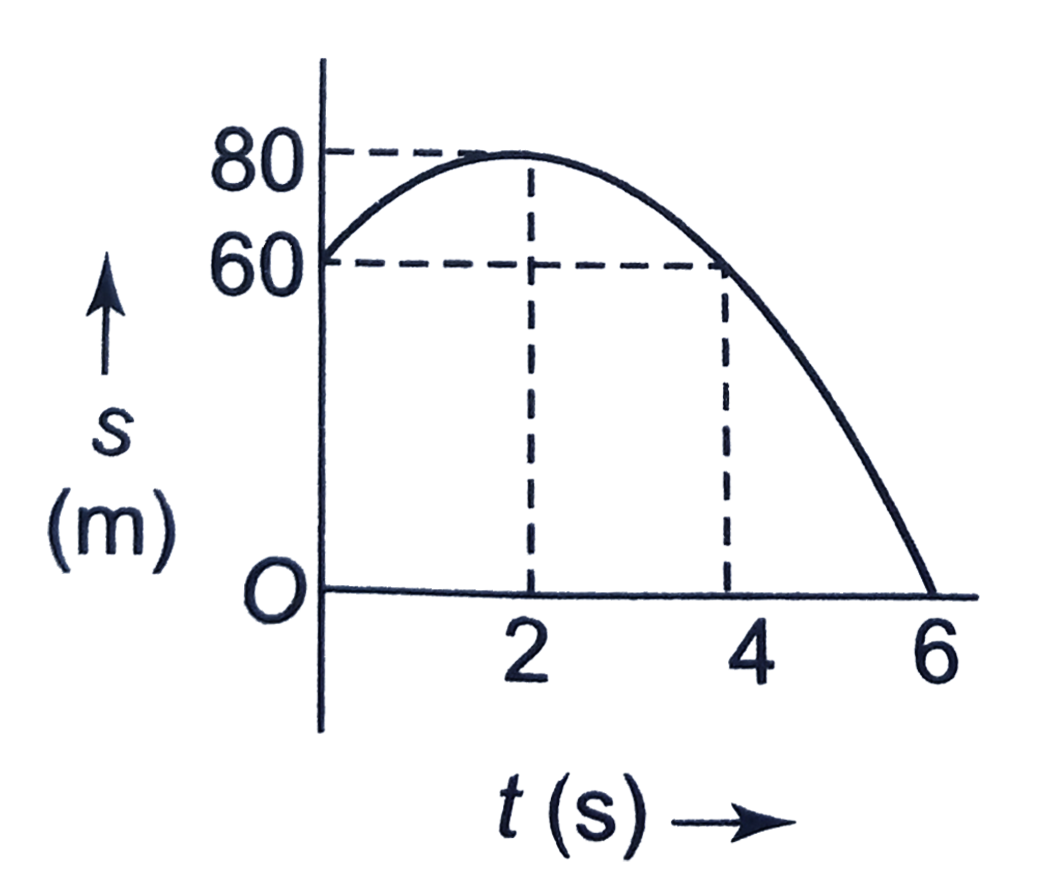
In the previous problem, assuming the foot of the tower as the origin, sketch the s-t graph.
Assuming the foot of the tower as the origin, the displacement is given by
s=60+ut−12gt2=60+20t−5t2
t=0,s=60m
t=2s,s=80m
t=4,s=60m
t=6s,s=0m
Shape: y=60+20x−5x2 (parabola, open downward)
Topper's Solved these Questions
Similar Questions
Solve the previous problem assuming the collision to take place in a colliding-beam accelerator in which the electrons and positrons meet head on with equal velocities.
View SolutionA rocket is launched from the suface of the earth vertically upward from rest. It moves under acceleration 8m/s2. After 15 s the fuel is finished, the rocket moves under gravity and ultimately strickes the ground. Assuming upward direction position and launching point as the origin, sketch the v-t, s-t and a-t graph.
View Solution
Knowledge Check
- Question 1 - Select One
In previous problem, the angular momentum of the projectile about the origin when it just before hits the ground should be.
A−2mv3isinθsinθgˆkBmv3isinθ2cosθ2gˆkC−mv3isinθ2cosθgˆkD2mv3isinθ2cosθgˆk. - Question 2 - Select One
PQ and RS are two vertical towers of the same height where S is on the ground Q is above the ground. The line joining the top P and the foot S of the two towers meets the horizontal line through Q at a point A where the angles of elevation of the tops P and R of the two towers are αandβ respectively. If AS = a, the height of the towers is
Aasin(β+α)cosβBacos(β+α)cosβCasin(β+α)sinβDacos(β+α)sinβ
Consider the situation of the previous problem.suppose the production of the radioactive isotope starts at t=0. Find the number of active nuclei at time t.
View SolutionIn the previous problem, if the particle occupies a position x=7m at t=1s, then obtain an expression for the instantaneous displacement of the particle.
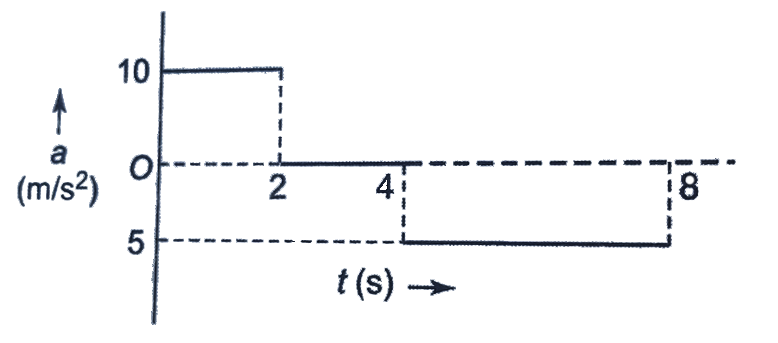
View SolutionConsider the following a-t graph for a particle moving along the x-axis. If the initial velocity is zero, sketch the v-t graph.
 View Solution
View SolutionIf in the previous problem, the particle travels 9/25 of the distance in its last second, find the time of fall and the height of the tower.
View SolutionWith the aid of numerical calculation find the instantaneous values of the acceleration and the speed of the falling ball in the previous problem and plot the graph. Choose an interval of time equal to Δt=0.02s.
View SolutionIsn't the result of the previous problem in contradiction with the fact that the ideal gas isochore in p-T coordinates is represented by a linear graph, while the iso chore of saturated vapour is nonlinear. ( see 35.3 Fig. 35.2)?
View Solution

