Making use of the solution of the foregoing problem, determine the probability of the particle with energy E=U0/2 to be located in the region x>l, if l2U0=(34π)2ħ2m.
U0l2=(34π)U0l2=(34π)2ħ2m and El2=(34π)2ħ22m
or kl=34π
It is easy to check that the condition of the bound state is satisfied. Also
αl=√2mħ2(U0−E)l2=√mU0ħ2l2=34π
Then from the previous problem
D=Aeαlsinkl=Ae3/4√2
By normalization
I=A2[∫l0sin2kxdx+∫∞l33π/22e−(3π/2)x/ldx]
A2[12∫10(1−cos2kx)dx+1∫∞012e−3x2ydy]
=A2⎡⎣12[−sin2kl2k]+12.l3π2⎤⎦=A2l⎡⎣12⎡⎣1+13π2⎤⎦+1213π2⎤⎦
A2l[12+23π]=A2l2(1+43π) or A=√2l(1+43π)−1/2
The probability of the particle to be located in the region x>l is
P=∫∞lΨ2dx=2l(1+43π)−1∫∞le3π/22e−3x2xldx
=(1+43π)−1∫∞le3π/2e−(3π/2)ydy=23π×3π3π×4=14.9%.
Topper's Solved these Questions
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Properties Of Atom
69 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Molecules And Crystals
48 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Elementary Particles
20 Videos
- DIRECT CURRENTBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - DIRECT CURRENTEXERCISE - All Questions
15 Videos
Similar Questions
Knowledge Check
What is the solution of the given set of equations ?
0.2u+0.3v=1.3
0.4u+0.5v=2.3Au=2,v=3Bu=−2,v=3Cu=2,v=2Du=3,v=3A particle is executing S.H.M. Ifu1 and u2 are the velocitiesof the particle at distancesx1 andx2 from the mean position respectively, then
AT=2π√x1+x2u1+u2BT=2π ⎷x22+x21u21+u22Cω= ⎷u22−u21x21−x22D2π√u1x2u2x1
A particle of mass m is located in a unidimensional potential field U(x) whose shape is shown in fig. where U(0)=∞. Find

(a) the equation defining the possible values of enrgy of the particle in the region E<U0, reduce taht equation to the from
sinkl=±kl√ħ2/2ml2U0,
where k=√2mE/ħ. Solving this equation by graphical means, domonstrate that hte possible values of energy of the particle from a discontinuous spectrum,
(b)the minium value of the quantity l2U0 at which the first energy level appears in the region E<U0. At what minimum value of l2U0 does the nth level appear?
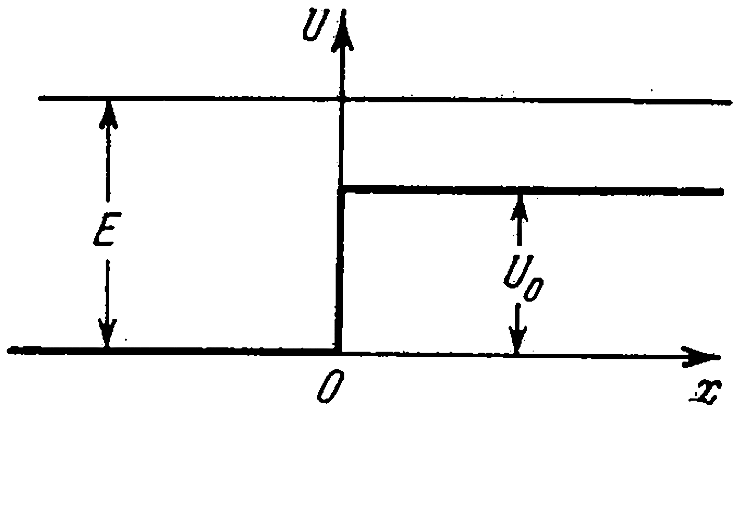
View SolutionParticle of mass m and energy E moves from the left to the potential barrier shown in figure. Find:
(a) the reflection coefficient R of the barrier for E>U0
(b) the effective penetration depth of the particles into the region x>0 for E<U0i.e., the distacne from the barrier boundary to the point at which the probability of finding a particle decreases e-fold
View SolutionA particle is executing SHM if u1andu2 are the speeds of the particle at distances x1andx2 from the equilibrium position, shown that the frequency of oscillation
f=12π(u21−u22x22−x21)
View SolutionA particle of mass mis located in a spherically symmetrical potential well U(r)=0 for r<r0 and U(r)=U0 for r>r0.
(a) By means of the substitution Ψ(r)=ξ(r)/r find the equation defining the proper values of energy E of the particle for E<U0, when its motion is described by a wave function Ψ(r) depending only on r. Reduce that equation to the form
sinkr0±kr0√ħ2/2mr20U0,wherek=√2mE/ħ
(b) Calculate the value of the quantity r20U0 at which the first level appears.
View SolutionA particle is executing S.H.M. Ifu1 and u2 are the velocitiesof the particle at distancesx1 andx2 from the mean position respectively, then
View SolutionPotential energy (U) related to coordinates is given by U=3(x+y).Work was done when the particle is going from (0,0) to (2,3) is
View Solution
