Obtain the maximum kinetic energy of β- particles, and the radiation frequencies of γ decays in the decay scheme shown in Fig. You are given that
m(.198Au)=197.968233u
m(.198Hg)=197.966760u.
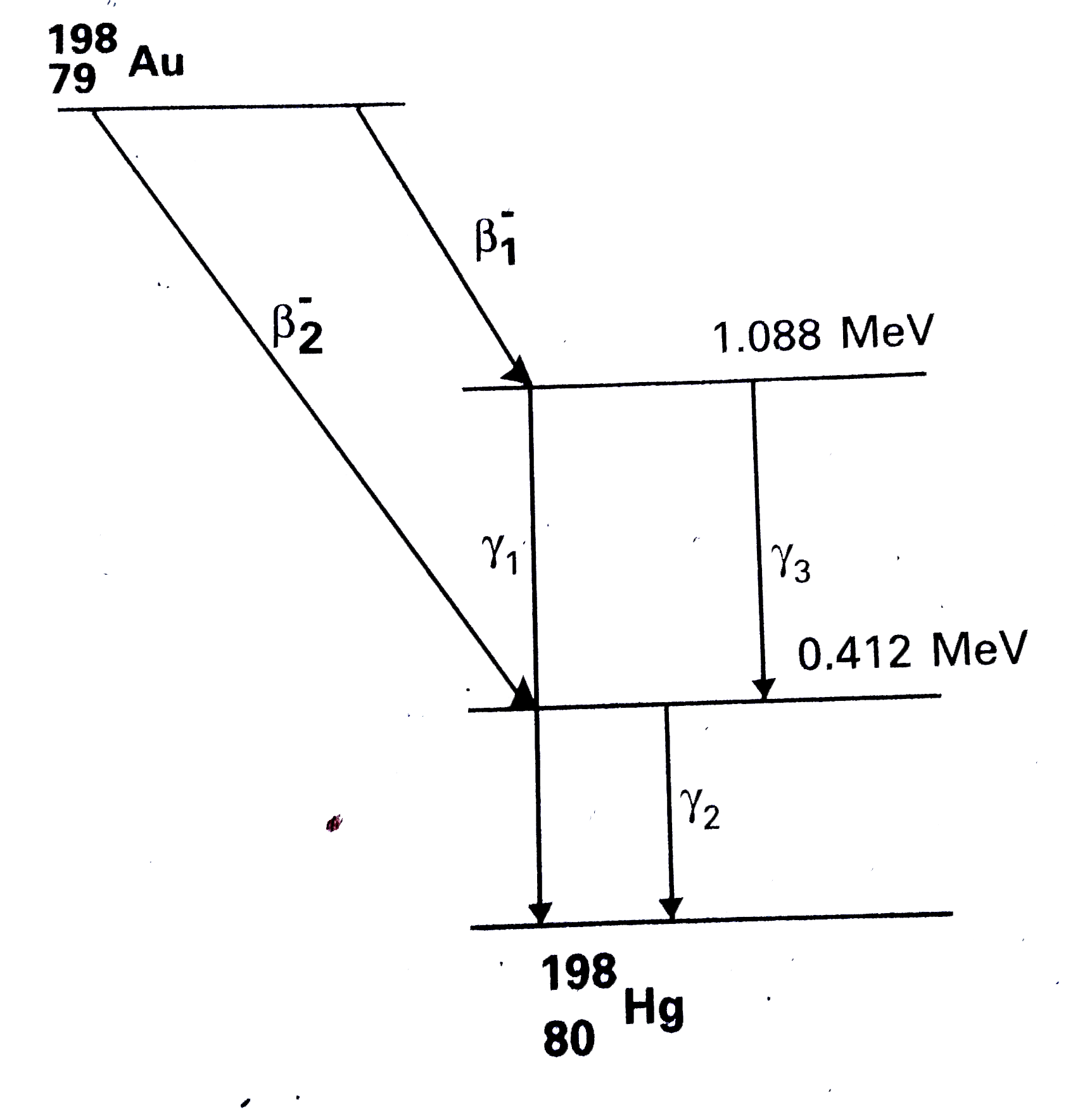
Energy corresponding to r1
E1=1.088−0=1.088MeV
=1.088×1.6×10−13 Joule
Frequency v1=E1h
=1.088×1.6×10−136.6×10−34
=2.63×1020Hz
similary v2=E2h
=0.412×1.6×10−126.6×1010
=9.98×1013Hz
and v3=E3h
=(1.088−0.412)×1.6×10−136.6×1020Hz
Maximum K.E. of β1 particle
Kmax(β1)=[m(.79Au198)− mass of second excited state of .80Hg198]×931MeV
=[m(.79Au198)−m(.82Hg198)−1.088931]×931MeV
=931[197.968233−197.966760]−1.088MeV
=1.371−1.088=0.283MeV
similarly kmax(β2)=0.957MeV
Topper's Solved these Questions
- NUCLEIBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - NUCLEIEXERCISE - DAM SURE (VSAQ)
1 Videos
- NUCLEIBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - NUCLEIEXERCISE - TEXTUAL EXERCISES
32 Videos
- MOVING CHARGES AND MAGNETISHMBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - MOVING CHARGES AND MAGNETISHMEXERCISE - ADDITIONAL EXERCISES
26 Videos
- RAY OPTICAL AND INSTRUMENTSBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - RAY OPTICAL AND INSTRUMENTSEXERCISE - TEXTUAL EXERCISES
60 Videos
Similar Questions
Obtain the maximum kinetic energy of β-particles, and the radiation frequencies of γ decays in the decay scheme shown in Fig. 14.6. You are given that m(.198Au)=197.968233u,m(.198Hg)=197.966760u
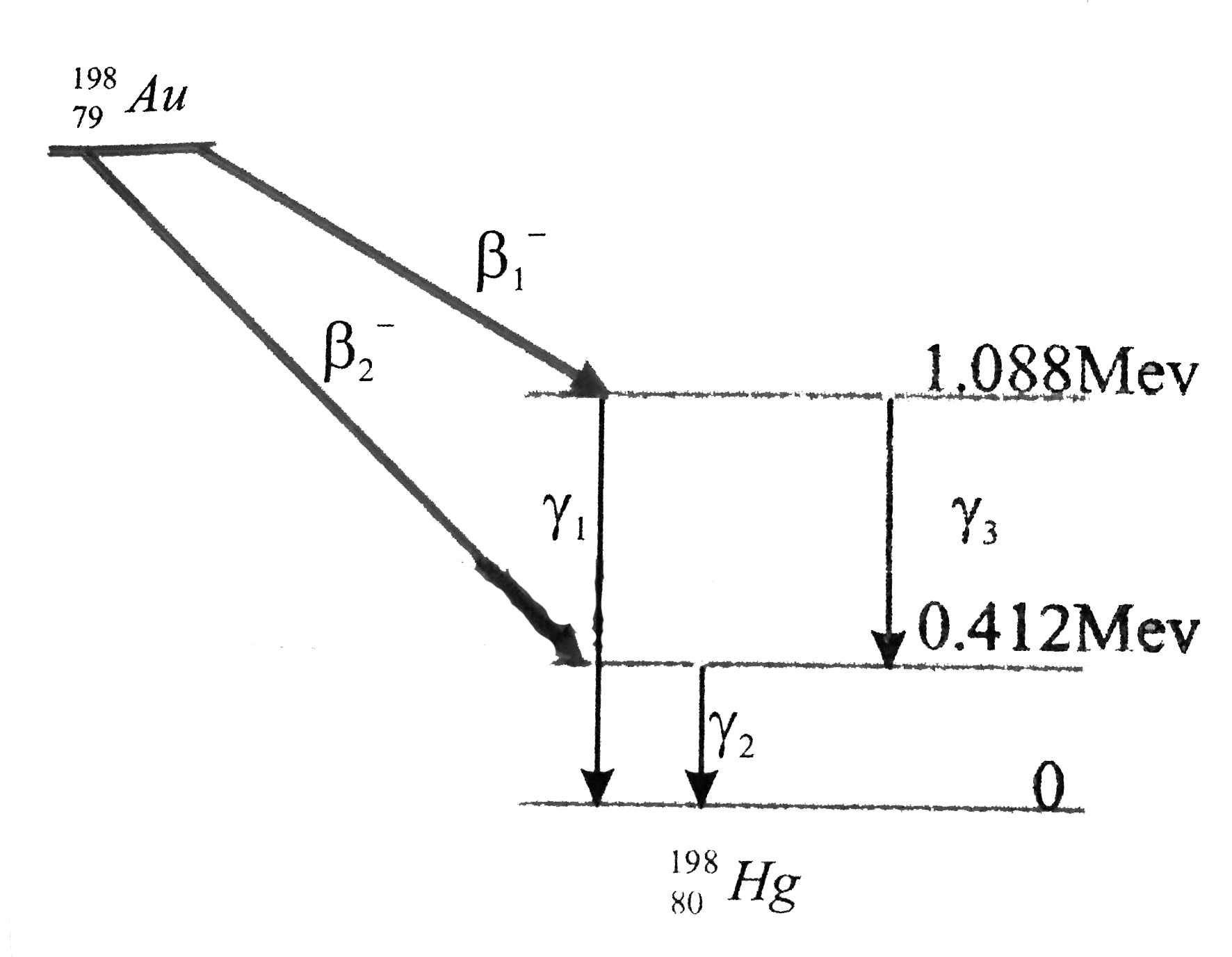
View SolutionObtain the maximum kinetic energy of β-particles, and the radiation frequencies of γ decays in the decay scheme shown in Fig. 14.6. You are given that m(.198Au)=197.968233u,m(.198Hg)=197.966760u

View Solution
Knowledge Check
The kinetic energy of α-particle emitted in the α-decay of 88Ra266 is [given, mass number of Ra = 222 u]
A5.201 Me VB3.301 Me VC6.023 Me VD4.871 Me V
Calculate the kinetic energy of β-particles and the radiation frequencies corresponding to the γ-decays shown in figure.
Given, mass of .12Mg27 atom =26.991425 amu and mass of .13Al27 atom = 26.990080 amu
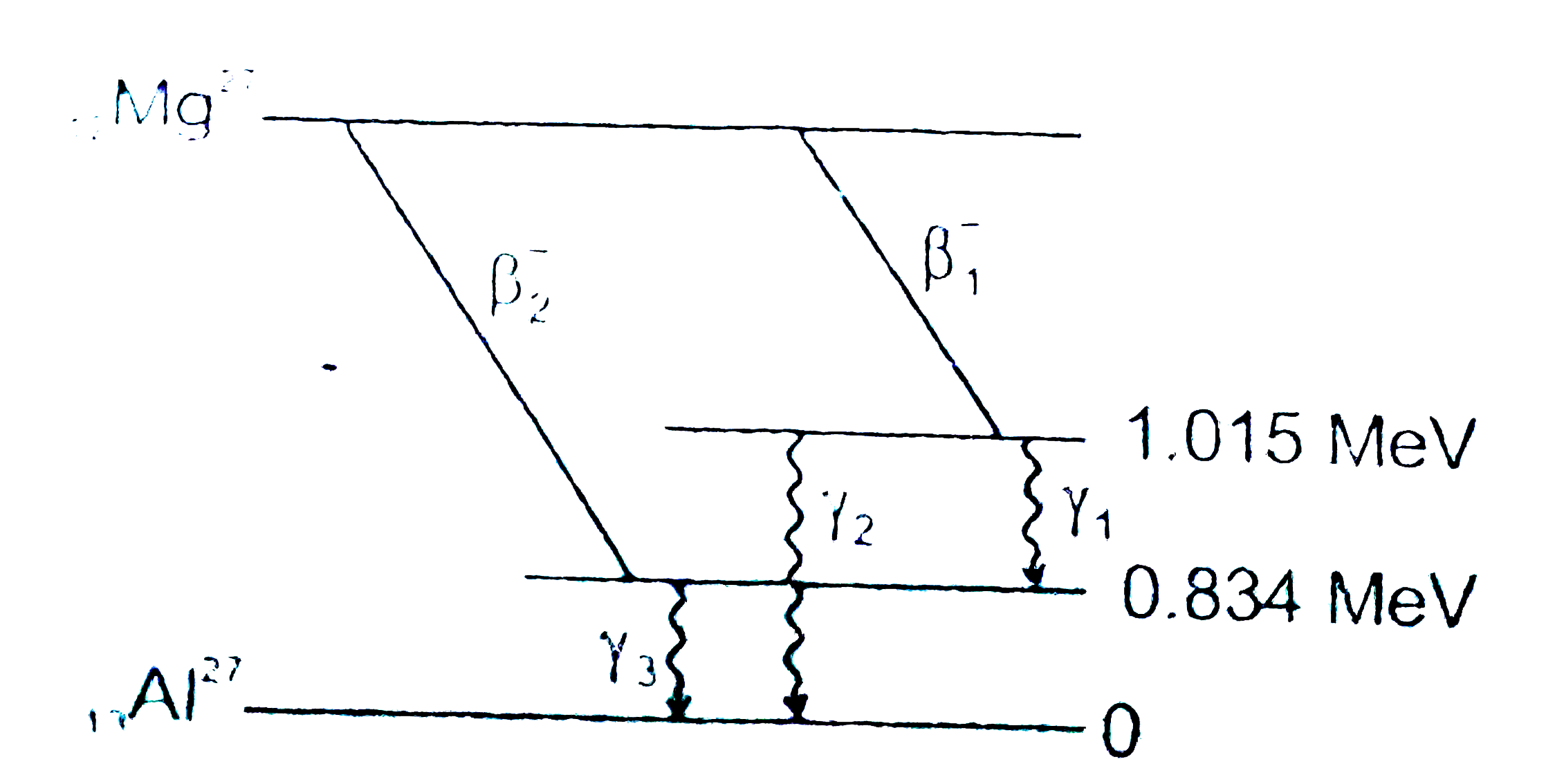
View SolutionCalculate the kinetic energy of β-particles and the radiation frequencies corresponding to the γ-decays shown in figure.
Given, mass of .12Mg27 atom =26.991425 amu and mass of .13Al27 atom = 26.990080 amu

View SolutionThe kinetic energy of α-particle emitted in the α-decay of 88Ra266 is [given, mass number of Ra = 222 u]
View SolutionCalculate the maximum kinetic energy of the bera particle emitted in the following decay scheme:
^12N rarr 12C∗+e++v
^12C rarr ^12C+γ(4.43MeV).
The atomic mass of 12Nis12.018612u.
View SolutionFind the maximum energy that a beta particle can have in the following decay
^176Lu→176Hf+e+→v.
Alomic mass of ^176Lu is 175.942694u and that of ^176Hf is 175.941420u.
View SolutionFind the maximum energy that a beta particle can have in the following decay
^176Lu→176Hf+e+→v.
Alomic mass of ^176Lu is 175.942694u and that of ^176Hf is 175.941420u.
View SolutionConsider the beta decay
^198Au→198Hg∗+Bη−1+→v.
where ^198Hg∗ represents a mercury nucleus in an excited state at energy 1.088MeV above the ground state. What can be the maximum kinetic energy of the electron emitted? The atomic mass of ^198Au is 197.968233u and that of ^198Hg is 197.966760u.
View Solution
