Show that two equal masses that undergo oblique elastic collision will move at right angles to each other after collision

Text Solution

Verified by Experts

Consider two bodies possess equal mass (m) and they undergo oblique elastic collision
Let the first body moving with initial velocity .u. collides with the second body at rest
In elastic collision, momentum is conserved. So, conservation of momentum along x - axis yields

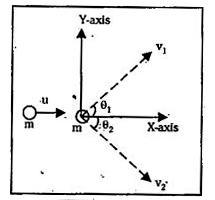
mu $= m v_{1} {cos θ}_{1} + m v^{2} {cos θ}_{2}$
(i.e.) $u = v_{1} {cos θ}_{1} + v_{2} {cos θ}_{2}$ . . . (1)
and along Y - axis
$v_{1} {sin θ}_{1} - v_{2} {sin θ}_{2}$ . . . (2)
squaring and adding eq. (1) and (2) we get
$u^{2} = v {(1)}^{2} + v_{2}^{2} + 2 v_{1} v_{2} cos (θ_{1} + θ_{2})$ . . . (3)
As the collision is elastic,
Kinetic Energy (K.E) is also conserved
(i.e.) $\frac{1}{2} m u^{2} = \frac{1}{2} m v_{1}^{2} + \frac{1}{2} m v_{2}^{2}$
$\Rightarrow u^{2} = v_{1}^{2} + v_{2}^{2} \to (4)$
From eq. (3) and (4)
$2 v_{1} v_{2} cos (θ_{1} + θ_{2}) = 0$
As it is given that $v_{1} \neq 0 and v_{2} \neq 0$
$∴ cos (θ_{1} + θ_{2}) = 0$
or $θ_{1} + θ_{2} = 90^{\circ}$
The two equal masses undergoing oblique elactic collision will move at right angles after collision, if the second body initially at rest

Updated on:21/07/2023

When two spheres of equal masses undergo glancing elastic collision with one of them at rest after collision they will move
Aopposite to one another
Bin the same direction
Ctogether
Dat right angle to each other
When two spheres of equal masses undergo glancing elastic collision with one of them at rest after collision they will move
Aopposite to one another
Bin the same direction
Ctogether
Dat right angle to each other
When two spheres of equal masses undergo glancing clastic collision with one of them at rest, after collision they will move
Aopposite to one another
Bin the same direction
Ctogether
Dat right angle to each other