Two balls of equal masses m each undergo oblique collision. If colision is perfectly elastic, then angle between their velocities after collision is
π4
π3
π6
π2
The correct Answer is:D
To solve the problem of finding the angle between the velocities of two balls of equal mass after a perfectly elastic oblique collision, we can follow these steps:
Step 1: Understand the Setup
We have two balls, each with mass m. One ball is moving with velocity v at an angle θ with respect to the normal line (the line connecting their centers at the point of collision), while the second ball is stationary.
Hint: Visualize the scenario by drawing the two balls and the normal line connecting them.
Step 2: Break Down the Velocity Components
For the moving ball, we can resolve its velocity v into two components:
- The component along the normal (towards the stationary ball): vcosθ
- The component perpendicular to the normal: vsinθ
Hint: Remember that the cosine function gives the adjacent side (along the normal), while the sine function gives the opposite side (perpendicular to the normal).
Step 3: Apply Conservation of Momentum and Kinetic Energy
In a perfectly elastic collision:
- The momentum along the normal direction is conserved.
- The kinetic energy is conserved.
Since the masses are equal, the velocities along the normal will interchange after the collision. Therefore:
- The first ball will have a velocity vsinθ (perpendicular to the normal) after the collision.
- The second ball will have a velocity vcosθ (along the normal) after the collision.
Hint: Recall that in elastic collisions involving equal masses, the velocities along the line of impact are exchanged.
Step 4: Determine the Angle Between the Velocity Vectors
After the collision:
- The first ball moves with velocity vsinθ (perpendicular to the normal).
- The second ball moves with velocity vcosθ (along the normal).
The angle between these two velocity vectors can be determined. Since one vector is along the normal and the other is perpendicular to it, the angle between them is 90∘ or π2 radians.
Hint: Use the geometric relationship that states if two vectors are perpendicular, the angle between them is 90∘.
Conclusion
Thus, the angle between the velocities of the two balls after the collision is 90∘ or π2 radians.
Final Answer: The angle between their velocities after the collision is 90∘ (or π2 radians).
---
We have two balls, each with mass m. One ball is moving with velocity v at an angle θ with respect to the normal line (the line connecting their centers at the point of collision), while the second ball is stationary.
For the moving ball, we can resolve its velocity v into two components:
- The component along the normal (towards the stationary ball): vcosθ
- The component perpendicular to the normal: vsinθ
In a perfectly elastic collision:
- The momentum along the normal direction is conserved.
- The kinetic energy is conserved.
- The first ball will have a velocity vsinθ (perpendicular to the normal) after the collision.
- The second ball will have a velocity vcosθ (along the normal) after the collision.
After the collision:
- The first ball moves with velocity vsinθ (perpendicular to the normal).
- The second ball moves with velocity vcosθ (along the normal).
Thus, the angle between the velocities of the two balls after the collision is 90∘ or π2 radians.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - A)
64 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - B)
35 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-J (AAKASH CHALLENGERS QUESTIONS)
14 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
Two balls each of mass 2kg (one at rest) undergo oblique collision is perfectly elastic, then the angle between their velocities after collision is
View SolutionBlock A has a mass 3kg and is sliding on a rough horizontal surface with a velocity uA=2m/s when it makes a direct collision with block B, which has a mass of 2kg and is originally at rest. The collision is perfectly elastic. Determine the velocity of each block just after collision and the distance between the blocks when they stop sliding. The coefficient of kinetic friction between the blocks and the plane is μk=0.3 ( Take g=10m/s2)
View SolutionTwo balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
View SolutionTwo balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
View SolutionConsider an oblique elastic collision between a moving ball and a stationary ball of the same mass. Both the balls move with the same speed after the collision. After the collision, the angle between the directions of motion of two balls is x degree. Find the value of x.
View SolutionTwo balls roll toward each other. The red ball has a mass of 0.5 kg and a speed of 4m/s just before impact. The green ball has a mass of 0.3 kg and a speed of 2 m/s. if the collision is perfectly inelastic, determine the velocity of the composite object after the collision.
View SolutionTwo particles of masses m and 2m moving in opposite directions collide elastically with velocity 2v and v, respectiely. Find their velocities after collision.

View SolutionA ball of mass m moving horizontally at a speed v collides with the bob of a simple pendulum at rest. The mass of the bob is also m. If the collision is perfectly elastic and both balls sticks, the height to which the two balls rise after the collision will be given by:
View SolutionA particle (a mud pallet, say) of mass m strikes a smooth stationary wedge of mass M with as velocity v0 at an angle θ with horizontal. If the collision is perfectly inelastic, find the
a. velocity of the wedge just after the collision.
b. Change in KE of the system (M+m) in collision.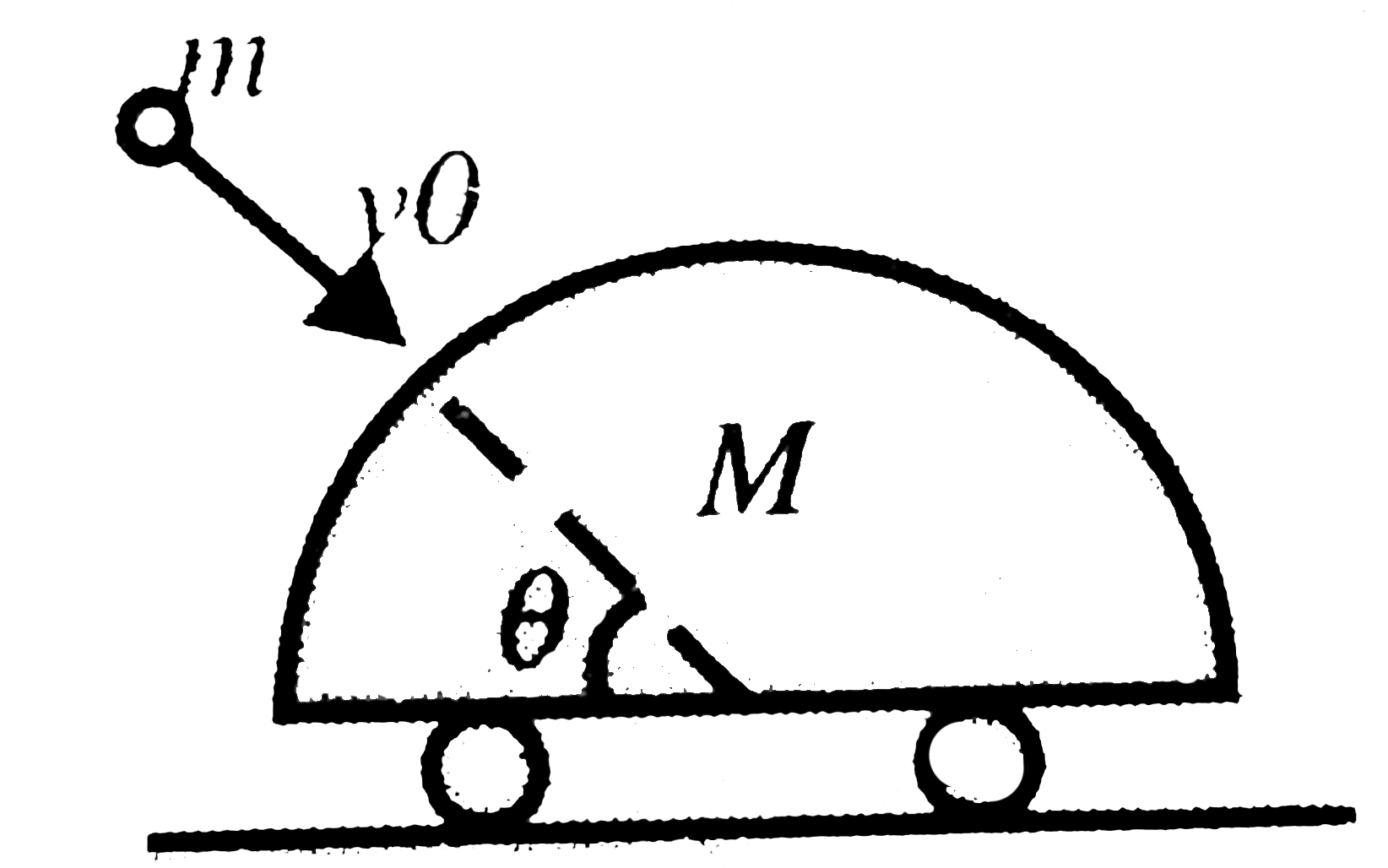
View SolutionAn object is moving towards a mirror with a velocity of 10ms−1 as shown in the figure. If the collision between the mirror and the object is perfectly elastic, then the velocity of the image after collision with mirror in vector form is
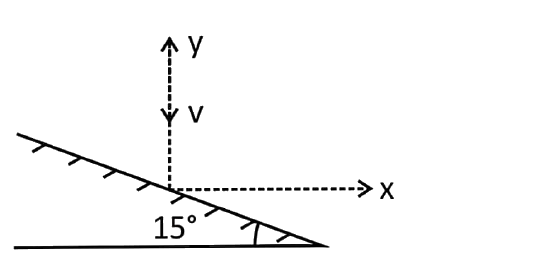
View Solution
