The bob of a stationary pendulum is given a sharp hit to impart it a horizontal speed of √3gl. Find the angle rotated by the string before it becomes slack.Solution in Tamil
The correct Answer is:A, C
V=√3gl
12mv2−12μ2=−mgh
v2=u2−2g(l+lcosθ)……….i
Aga∈(mv^2)/l=mgcostheta<br>v^2=lg costheta<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/HCV_VOL1_C08_E01_093_S01.pngwh=80%mx-autosty≤=background:white;><br>Fromequationiandiiwe≥t<br>3gl-2gl-2gl cos theta=glcostheta<br>3costheta=1<br>=cos^-1(-1/3)
Topper's Solved these Questions
Similar Questions
A heavy stone is thrown from a cliff of height h with a speed v. The stone will hit the ground with maximum speed if it is thrown
View SolutionA wave pulse passing on a string with speed of 40cms−1 in the negative x direction has its maximum at x=0 at t=0. Where will this maximum be located at t=5s?
View SolutionThe bob of a pendulum at rest is given a sharp hit to impat a horizontal velocity √10gl where l is the length of the pendulum. Find the tension in the string when a. the string is horizontal. B. The bob is at its highest point and c. the string makes an angle of 600 with teh upward vertical.
View SolutionThe bobo of a pendulum at rest is given a sharp hit to impart a horizontal velocity u where l is the length of the pendulum. Find the angle rotated by the string before it becomes slack.
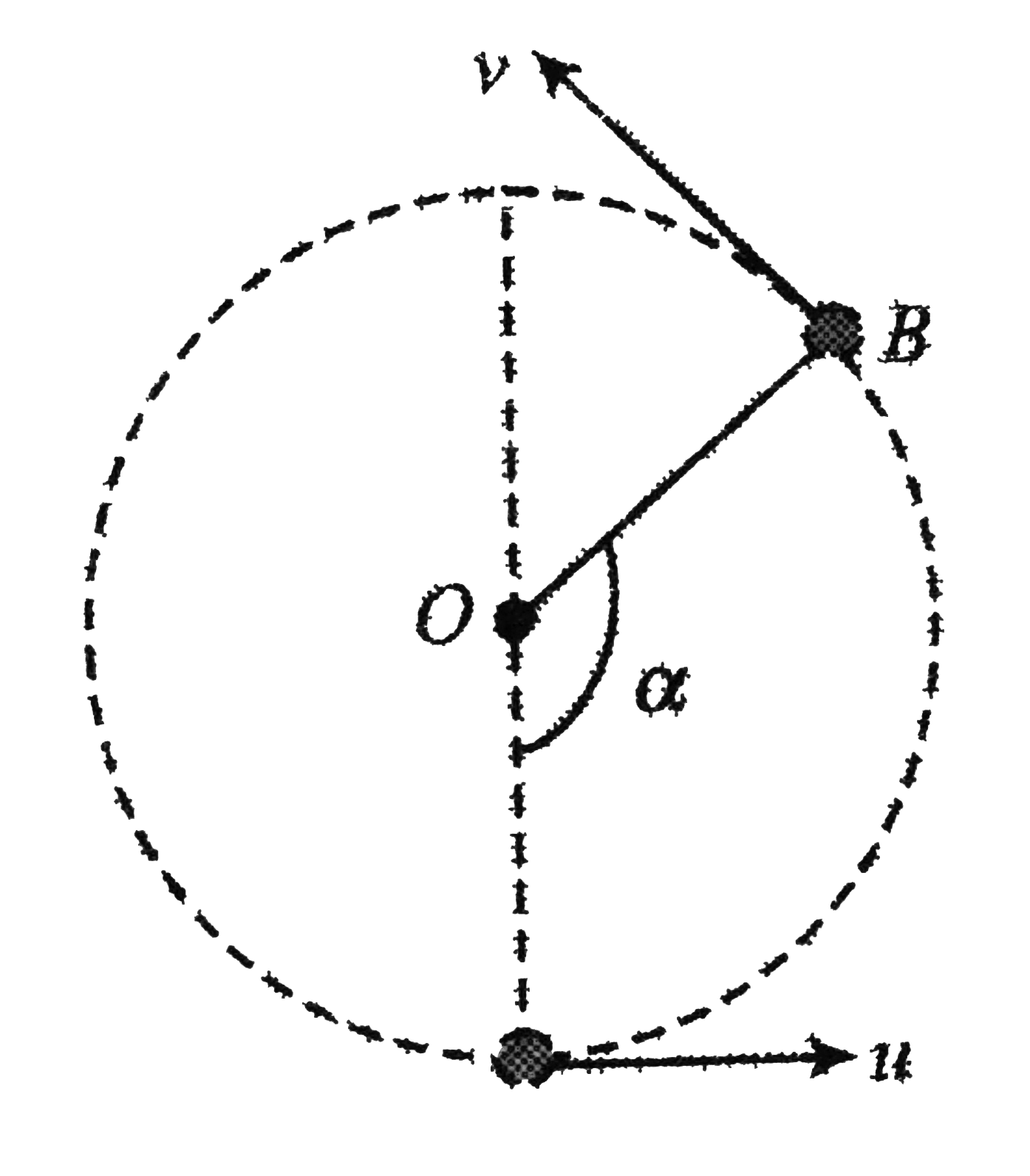
View SolutionThe bob of a simple pendulum is given a sharp hit impart it a horizontal speed of √3gL. Find an angle made by the string with the upper verticle before it becomes slack. Also, calculate the maximum height attained by the bob above the point of suspension. L is length of the string.
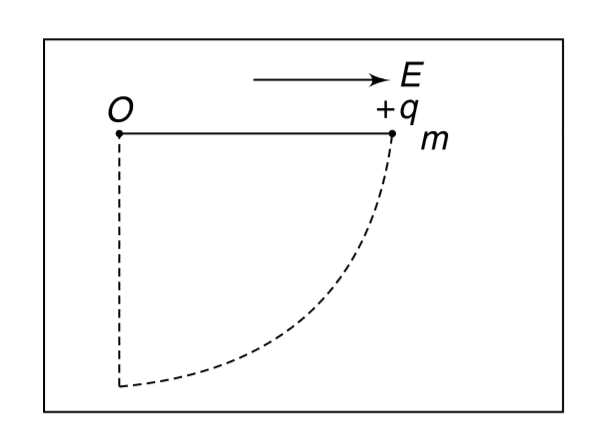
View SolutionThere is a uniform horizontal electric field of strength E in a region. A pendulum bob is pulled to make the string horizontal and released. The bob has mass m and charge q. (a) Find the maximum angle (θ0) that the bob swings before coming to rest momentarily. (b) Find E if the bob comes to rest when the string is vertical.
View SolutionThe bob of pendulum is project with horizontal velocity of √3gf I is the length of string. Find the angular displacement of string before it becomes slack.

View SolutionThe bob of a simple pendulum of length l is given a sharp hit to impart it a horizontal speed of √3gl . When it was at its lowermost position. Find
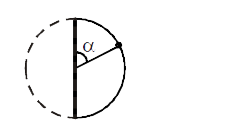
angle α shown of the string from upside of vertical and speed of the particle when the string becomes slack.
View SolutionMass of the bob of a pendulum is 100g and the length of the string is 1m . The bob is initially held is such a way that the string is horizontal . The bob is then released. Find the kinetic energy of the bob when the string makes an angle of (i) 0^@ with vertical
View SolutionMass of the bob of a pendulum is 100g and the length of the string is 1m . The bob is initially held is such a way that the string is horizontal . The bob is then released. Find the kinetic energy of the bob when the string makes an angle of (ii) 30^@ with the vertical .
View SolutionBob of the simple pendulum is at rest at its lowest position , Sharp hit imparts a speed of sqrt(9gl) to the pendium bob. Calculate tension of the thread when it makes an angle 60^(@) with the vertical .
View SolutionThe bob of a pendulum at rest is given a sharp hit to impart a horizontal velocity √10gl where l is the length of the pendulum. Find the tension in the string when a. the string is horizontal. B. The bob is at its highest point and c. the string makes an angle of 600 with the upward vertical.
View SolutionThe simple pendulum bob is given a horizontal velocity sqrt(gl) at the bottom. The angle with the vertical through which the ball swings before its velocity becomes zero is
View SolutionA simple pendulum bob is given a horizontal velocity √72gl at the bottom. The string slackens after swinging through an angle θ with the vertical. The θ is
View SolutionA simple pendulum bob is given a horizontal velocity √72gl at the bottom. The string slackens after swinging through an angle θ with the vertical. The θ is:
View SolutionA simple pendulum bob is given a horizontal velocity sqrt((7)/(2)gl) at the bottom. The string slakens after swinging through an angle theta with the vertical. The theta is
View Solution
