The distance between the centres of two stars is 10α. The masses of these stars are M and 16M and their radii α and 2α. A body of mass m is fired straight from the surface of the larger star towards the smaller star. What should be its minimum initial speed to reach the surface of teh smaller star? Obtain the expression interms of G, M and α.
The correct Answer is:A, B, C
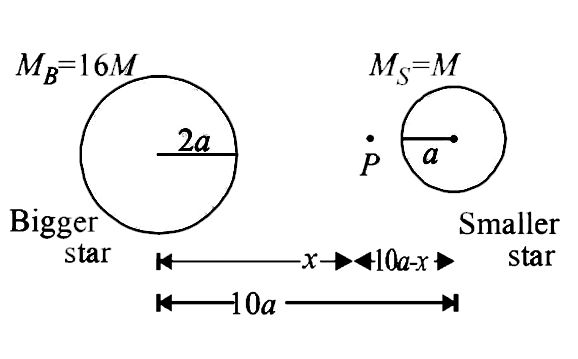
The force of attraction is zero at say x from the bigger star.
Then force on mass m due to bigger star = Force on mass m
due to small star
GMBmx2=GMSm(10a−x)2⇒16Mx2=M((10a−x))2⇒x=8a
If we throw a mass m from bigger star giving it such a velocity
that is sufficient to bring it to P, then later on due to greater
force by the star Ms it will pull it towards itself [without any
external energy thereafter].
The energy of the system (of these masses) initially
= Final energy when m is at P
−GMBMs10a−GMBm2a−GMSm8a+12mv2
=−GMBMS10a−GMBm8a−GMSm2a
[∴MB=16M,MS=M)
∴v=32√5GMa
Topper's Solved these Questions
Similar Questions
Distance between the centres of two stars is 10a. The masses of these stars are M and 16M ans their radit a nd 2a respectively. A body of mass m is fired straight from the surface of the larger star tpwards the surface of the smaller star. What should be its minimum intial speed to reach the surface of the smaller star? Obtain the expression in terms of G, M and a.
View SolutionDistance between the centres of two rings is a. The masses of these rings are M and 4M. A body of mass m is fired straight from the centre of the heavier ring. What should be its minimum initial speed to cross of lighter ring ? The radius of the both ring is R. (Assume a>>R).

View Solution
Knowledge Check
Distance between the centres of two stars is 10a. The masses of these stars are M and 16M and their radii a and 2a respectively. A body of mass m is fired straight from the surface of the larger star towards the smaller star. The minimum initial speed for the body to reach the surface of smaller star is
A23√GmaB32√5GmaC23√5GmaD32√GmaA particle is projected from the surface of one star towards other star of same radius a and mass with such a minimum velocity K√GMa, so that it is attracted towards other star. Find the value of K if two stars are 2r distance apart:
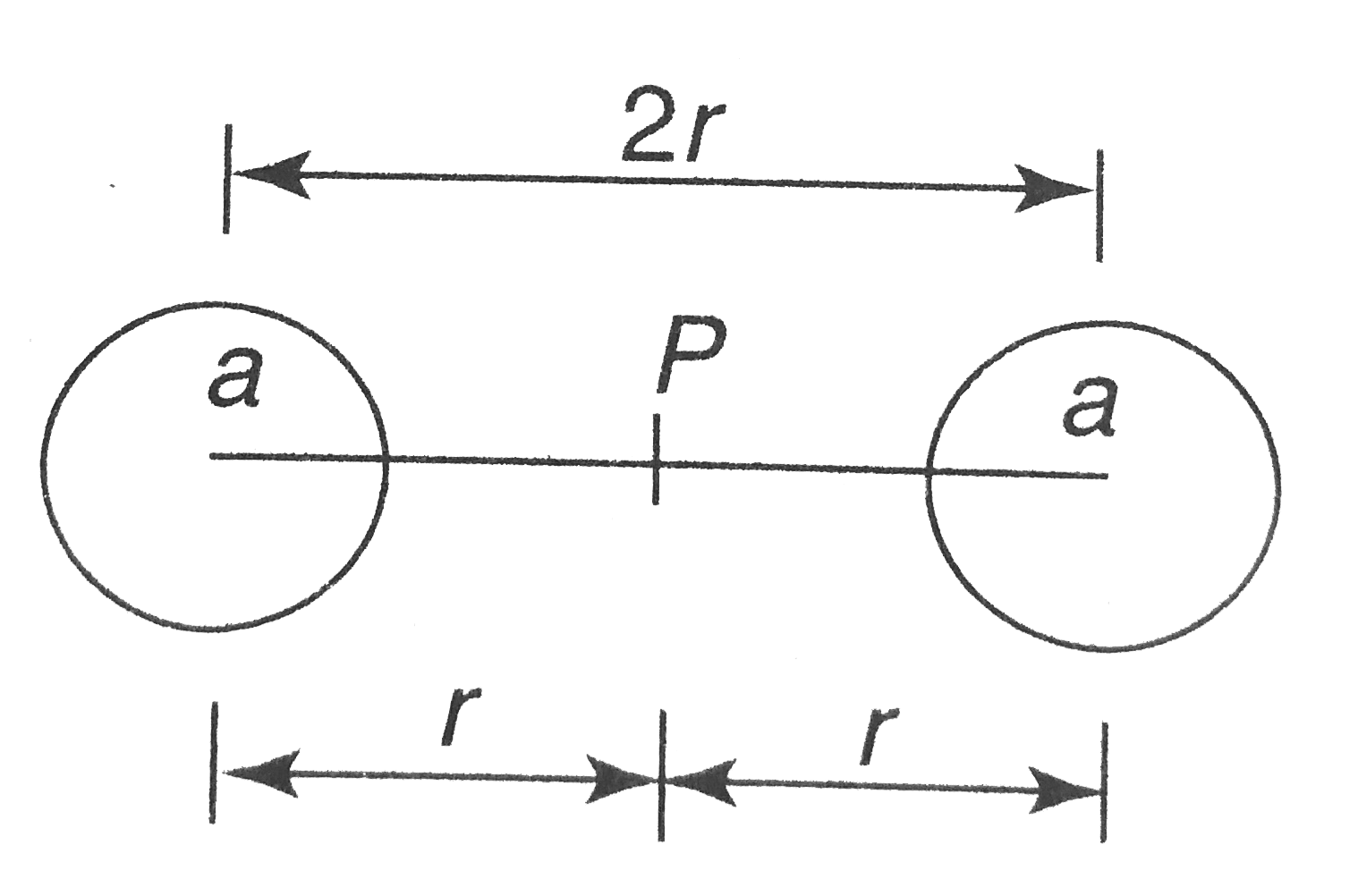 A2(r−a)[r(2r−a)]1/2B2(r−a)[r(r−a)]1/2Cr−a[r(r−a)]1/2Dr+a[r(r−a)]1/2
A2(r−a)[r(2r−a)]1/2B2(r−a)[r(r−a)]1/2Cr−a[r(r−a)]1/2Dr+a[r(r−a)]1/2A pair of stars rotates about their centre of mass One of the stars has a mass M and the other has mass m such that M=2m The distance between the centres of the stars is d (d being large compared to the size of either star) .
The period of rotation of the stars about their common centre of mass (in time of d ,m ,G) is .A√4π2GMd3B√8π2GMd3C√2π23GMd3D√4π23GMd3
Two stars of masses M and 4M have radii x and 3x respectively. The distance between centres of two stars is 12x. A body of mass m is fired from the surface of bigger sphere towards smaller sphere. Find the minimum initial speed (in terms of G, x, M) required to reach the surface of smaller star.
View SolutionTwo planets have masses M and 16 M and their radii are a and 2a, respectively. The separation between the centres of the planets is 10a. A body of mass m is fired trom the surface of the larger planet towards the samller planet along the line joining their centres. For the body to be able to reach at the surface of smaller planet, the minimum firing speed needed is :
View SolutionThe masses of two stars are m1 and m2, and their separation is l. Determine the period T of their revolution in circular orbits about a common centre.
View SolutionTwo uniform solid spheres of equal radii R, but mass M. and 4M have a centre to centre separation 6R, as shown in the figure. The two spheres are held fixed. A projectile of mass m is projected from the surface of the sphere of mass M directly towards the center of the second sphere. If the minimum speed v of the projectile so that it reaches the surface of the second sphere is √xGMyR, then find (x,+y).
'(##CEN_KSR_PHY_JEE_C10_038_Q05##)'
View SolutionA pair of stars rotates about their centre of mass One of the stars has a mass M and the other has mass m such that M=2m The distance between the centres of the stars is d (d being large compared to the size of either star) .
The period of rotation of the stars about their common centre of mass (in time of d ,m ,G) is .
View Solution
