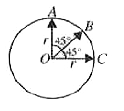
The magnitude of vectors $- - \to O A, - - \to O B and - - \to O C$ in figure are equal. Find the direction of $- - \to O A + - - \to O B - - - \to O C$ .
.

Video Solution

Text Solution

Verified by Experts

Let $O A = O B = O C = F$ .
x - component of $- - \to O A = F {cos 30}^{0} = F \frac{\sqrt{3}}{2}$
x-component of $- - \to O B = F {cos 60}^{0} = \frac{F}{2}$
$x - c o m p o \neq n t o f$ vec(OC)=F cos 135^0= - F/sqrt2 $< b r >$ x-component of $- - \to O A + - - \to O B - - - \to O C$
$- (\frac{F \sqrt{3}}{2}) + (\frac{F}{2}) + (- \frac{F}{\sqrt{2}})$
$= \frac{F}{2} (\sqrt{3} + 1 + \sqrt{2})$ .
y-component of $- - \to O A = F {cos 60}^{0} = \frac{F}{2}$
y- component of $- - \to O B = F {cos 150}^{0} = (F \frac{\sqrt{3}}{2}$
y- component of $- - \to O C = F {cos 45}^{0} = \frac{F}{\sqrt{2}}$ .
y-component of $- - \to O A + - - \to O B + - - \to O C$
$= (\frac{F}{2}) (\sqrt{3} + 1 + \sqrt{2})$
y-component of $- - \to O A = F {cos 60}^{0} = \frac{F}{2}$
y-component of $- - \to O C = F {cos 45}^{0} = \frac{F}{\sqrt{2}}$
y-component of $- - \to O A + - - \to O B + - - \to O C$
$= (\frac{F}{2}) + (- \frac{F \sqrt{3}}{2} - (\frac{F}{\sqrt{2}})$
=F/2 (1-sqrt3-sqrt2) $< b r > A n g \leq o f$ vec(OA)+vec(OB)-vec(OC) $w i t h t h e X - a ξ s < b r >$ =tan^-1 (F/2 (1-sqrt3-sqrt2))/(F/2 (1+sqrt3+sqrt2))= tan^-1 (1-sqrt3-sqrt2)/(1+sqrt3+sqrt2)

Updated on:21/07/2023

The resultant of the three vectors $- - \to O A, - - \to O B$ and $- - \to O C$ shown in figure is

Ar
B2r
C $r (1 + \sqrt{s})$
D $r (\sqrt{2} - 1)$
Two vector $\to A$ and $\to B$ have equal magnitudes. Then the vector $\to A + \to B$ is perpendicular :
A $\to A \times \to B$
B $\to A - \to B$
C $3 \to A - 3 \to B$
DAll of these
Let O, O' and G be the circumcentre, orthocentre and centroid of a $Δ A B C$ and S be any point in the plane of the triangle.
Statement -1: $- -- \to O' A + - -- \to O' B + - -- \to O' C = 2 - -- \to O' O$
Statement -2: $- \to S A + - - \to S B + - - \to S C = 3 - - \to S G$
AStatement - 1 is True, Statement - 2 is True , Statement - 2 is a correct explanation for Statement - 1.
BStatement -1 is True, Statement - 2 is True, Statement -2 is not a correct explanation for Statement - 1.
CStatement - 1 is True, Statement - 2 is False.
DStatement - 1 is False, Statement - 2 is True.