The radii of two concentric spherical conducting shells are r1andr2(>r1). The change on the oute shell is q. The charge on the inner shell which is connected to the earth is
q(r2r1)
q2(r1r2)
−q(r1/r2)
q2(r2r1)
The correct Answer is:C
To solve the problem, we need to determine the charge on the inner spherical conducting shell, which is connected to the earth, given that the outer shell has a charge q.
1. Understanding the System:
- We have two concentric spherical conducting shells.
- The inner shell has a radius r1 and the outer shell has a radius r2 where r2>r1.
- The outer shell carries a charge q.
- The inner shell is connected to the earth.
2. Potential of the Inner Shell:
- When a conductor is connected to the earth, its potential becomes zero because the earth can provide or absorb an infinite amount of charge.
- Therefore, the potential V1 of the inner shell must be zero.
3. Calculating the Potential:
- The potential V1 at the surface of the inner shell due to its own charge Q1 and the charge q on the outer shell can be expressed as:
V1=kQ1r1+kqr2
- Here, k is the electrostatic constant.
4. Setting the Potential to Zero:
- Since the potential V1 is zero (because the inner shell is grounded), we can set up the equation:
kQ1r1+kqr2=0
5. Simplifying the Equation:
- We can factor out k (since k is a constant and does not equal zero):
Q1r1+qr2=0
6. Solving for Q1:
- Rearranging the equation gives:
Q1r1=−qr2
- Multiplying both sides by r1:
Q1=−q⋅r1r2
7. Conclusion:
- The charge on the inner shell, which is connected to the earth, is:
Q1=−q⋅r1r2
Final Answer:
The charge on the inner shell is Q1=−q⋅r1r2.
---
- We have two concentric spherical conducting shells.
- The inner shell has a radius r1 and the outer shell has a radius r2 where r2>r1.
- The outer shell carries a charge q.
- The inner shell is connected to the earth.
- When a conductor is connected to the earth, its potential becomes zero because the earth can provide or absorb an infinite amount of charge.
- Therefore, the potential V1 of the inner shell must be zero.
- The potential V1 at the surface of the inner shell due to its own charge Q1 and the charge q on the outer shell can be expressed as:
V1=kQ1r1+kqr2
- Here, k is the electrostatic constant.
- Since the potential V1 is zero (because the inner shell is grounded), we can set up the equation:
kQ1r1+kqr2=0
- We can factor out k (since k is a constant and does not equal zero):
Q1r1+qr2=0
- Rearranging the equation gives:
Q1r1=−qr2
- Multiplying both sides by r1:
Q1=−q⋅r1r2
- The charge on the inner shell, which is connected to the earth, is:
Q1=−q⋅r1r2
The charge on the inner shell is Q1=−q⋅r1r2.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.3
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.4
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.1
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
The capacitance of two concentric spherical shells of radii R1 and R2(R2>R1) is
View SolutionThere are two concentric hollow conducting spherical shells of radii r and R(R>r). The charge on the outer shell is Q. What charge should be given to the inner shell, so that the potential at a point P , at a distance 2R from the common centre is zero ?
View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionThere are two concentric metal shells of radii r1 and r2(>r1). If initially, the outer shell has a charge q and the inner shell is having zero charge and then inner shell is grounded. Find :
(i) Charge on the inner surface of outer shell.
(ii) Final charges on each sphere.
(iii) Charge flown through wire in the ground.
View SolutionConsider two concentric spherical metal shells of radii 'a' and b>a .The outer shell has charge Q but the inner shell has no charge.Now the inner shell is grounded ,This means that the inner shell will come at zero potential and that electric fields lines leave the outer shell and end on the inner shell.(a) Find the charge on the inner shell
Find the potential on outer sphere.
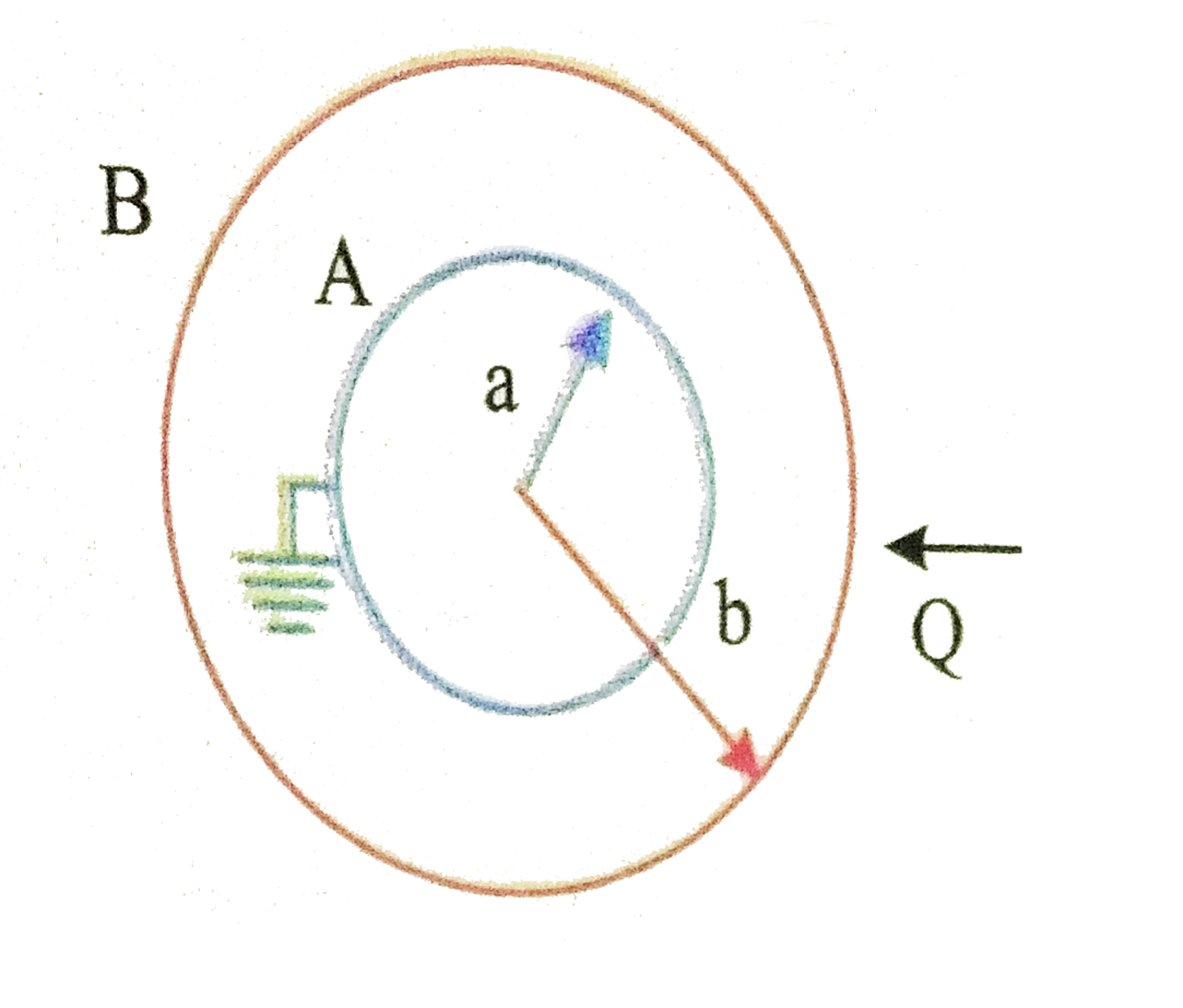
View SolutionThere are two concentric spherical shells with inner shell having positive charge q and outer shell having a charge -q. Now outer shell is supplied a charge -2q and inner shell is earthed, Calculate the ratio of initial and final capacities of the system. (If a = 3R, b= 5R)
View SolutionTwo concentric conducting spherical shells of radii a1anda2(a2>a1) are charged to potentials ϕ1 and ϕ2, respectively. Find the charge on the inner shell.
View SolutionTwo concentric spherical conducting shells of radii R and 2R carry charges Q and 2Qrespectively.Change in electric potential on the outer shell when both are connected by a conducting wire is (k=14πɛ0)
View SolutionThere are two concentric conducting spherical shells of radii 10cm and 20cm. The outer shell is charged to a potential of 50esu while inner shell is earthed. The charge on outer shell is
View SolutionThere are two thin concentric conducting shells of radii a and b(a<b) . Equal charges Q given to each shell. Find the charge distribution on the inner and outer shell.
View Solution
