The refractive index of a medium 'x' with respect to a medium 'y' is $\frac{2}{3}$ and the refractive index of medium 'y' with respect to medium 'z' is $\frac{4}{3}$ . Find the refractive index of medium 'z' with respect to medium 'x' . If the speed of light in medium 'x' is $3 \times 10^{8} m s^{- 1}$ , Calculate the speed of light in medium 'y'.

Video Solution

Text Solution

Verified by Experts

Sign Convention.
(1) All the distances are to be measured from the pole of the surface.
(2) The distances measured in the same direction as the incident light are taken as positive.
(3) The distances measured in the direction opposite to the direction of the incident light are taken as negative.

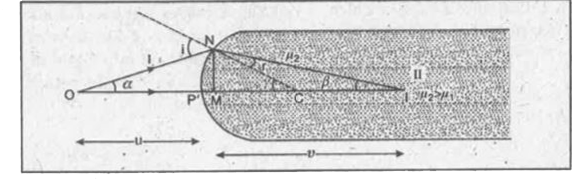
Assumptions. (1) Object is taken as a point object lying on the principal axis.
(2) The aperture of refracting surface is small.
(3) The incident ray from the object strikes the surface at a point very close to point so that angles of incidence and refraction are very small.
Consider a point object O on the principal axis. A ray ON is incident at N and refracts along NI. Another ray OM goes undeviated to meet the previous ray at I. Thus I is the real image of the object O.
Let $μ_{1} = Refractive index of medium I$
$μ_{2} = Refractive index of medium II$ ,
such that
$μ_{2} > μ_{1}$
For small apertures (namely $M N < O M$ ), the problem can easily be solved.
In $Δ O N C$ ,
$i = ∠ N O M + ∠ N C M$
In $Δ O N M$ ,
From Snell.s law, for small i and r, we have
$\frac{\sin i}{\sin r} = \frac{i}{r} = \frac{μ_{2}}{μ_{1}}$
or $μ_{1} i = μ_{2} r$ ...(1)
In $Δ N O C$ ,
$i = α + β$
In $Δ N I C$ ,
$γ = r + β$ or $r = γ - β$
Putting the values of i and r in equation (1), we get
$μ_{1} (α + γ) = μ_{2} (γ - β)$
or $μ_{1} α + μ_{1} γ = μ_{2} γ - μ_{2} β$
or $μ_{1} α + μ_{2} β = (μ_{2} - μ_{1}) γ$ ...(2)
Since $α, β$ and $γ$ are small, so they can be replaced by their tangents. So equation (2) becomes
$μ_{1} \tan α + μ_{2} \tan β = (μ_{2} - μ_{1}) \tan γ$
or $μ_{1} \frac{N M}{M O} + μ_{2} \frac{N M}{M I} = (μ_{2} - μ_{1}) \frac{N M}{M C}$
or $\frac{μ_{1}}{M O} + \frac{μ_{2}}{M I} = \frac{μ_{2} - μ_{1}}{M C}$
Since M is very close to the pole P, So $M O ≅ P O, M I ≅ P I$ and $M C ≅ P C$
$∴ \frac{μ_{1}}{P O} + \frac{μ_{2}}{P I} = \frac{μ_{2} - μ_{1}}{P C}$
Using sign conventions, PO= - u, PI = v and PC = R, we get
$- \frac{μ_{1}}{u} + \frac{μ_{2}}{v} = \frac{μ_{2} - μ_{1}}{R}$
or $\frac{μ_{2}}{v} - \frac{μ_{1}}{u} = \frac{μ_{2} - μ_{1}}{R}$

Updated on:21/07/2023

Refractive index of a medium depends
Aon the medium only
Bon the incident light only
Con both the conditions given in options a and b
DNone of the above
Refractive index of a medium depends on
AOn the medium only
BOn the incident light only
COn both the conditions given in options (on the medium only) and (on the incident light only)
DNone of the above
Refractive index of a medium depends on
A $\sqrt{μ_{r} ε_{r}}$
B $\sqrt{μ_{0} ε_{0}}$
C $\frac{1}{\sqrt{μ_{r} ε_{r}}}$
D $\frac{1}{\sqrt{μ_{0} ε_{0}}}$