Two blocks of msses 10 kg and 30 kg are placed along a vertical line. The first block is raised through a height of 7 cm. By what distance should the second mass be moved to raise the centre of mass by 1 cm?
To solve the problem, we need to find out how far the second block (30 kg) should be moved in order to raise the center of mass of the system by 1 cm after the first block (10 kg) is raised by 7 cm.
1. Identify the masses and displacements:
- Mass of block 1, m1=10kg
- Mass of block 2, m2=30kg
- Displacement of block 1, s1=7cm (upward)
- Displacement of block 2, s2 (unknown, downward)
2. Set up the center of mass equation:
The formula for the displacement of the center of mass (scm) is given by:
(m1+m2)scm=m1s1+m2s2
Here, we want the center of mass to be raised by scm=1cm.
3. Substitute the known values into the equation:
(10+30)⋅1=10⋅7+30⋅s2
Simplifying this gives:
40=70+30s2
4. Rearrange the equation to solve for s2:
30s2=40−70
30s2=−30
s2=−3030=−1cm
5. Interpret the result:
The negative sign indicates that the second block should be moved downward. Therefore, the second mass should be moved down by 1 cm.
Final Answer:
The second block (30 kg) should be moved down by 1 cm.
---
- Mass of block 1, m1=10kg
- Mass of block 2, m2=30kg
- Displacement of block 1, s1=7cm (upward)
- Displacement of block 2, s2 (unknown, downward)
The formula for the displacement of the center of mass (scm) is given by:
(m1+m2)scm=m1s1+m2s2
Here, we want the center of mass to be raised by scm=1cm.
(10+30)⋅1=10⋅7+30⋅s2
Simplifying this gives:
40=70+30s2
30s2=40−70
30s2=−30
s2=−3030=−1cm
The negative sign indicates that the second block should be moved downward. Therefore, the second mass should be moved down by 1 cm.
The second block (30 kg) should be moved down by 1 cm.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Question For short Answer
25 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -2
11 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -1
18 Videos
- CALORIMETRYBOOK - HC VERMA ENGLISHCHAPTER - CALORIMETRYEXERCISE - Short answer
9 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
Similar Questions
Two bocks of masses 10 g and 20 kg are plced on the X-axis. The first mass is moved on the axis by a distance of 2 cm. By what distance should the second mass be moved to keep the position of the centre of mass unchanged?
View SolutionConsider a system of two particles having masses 2kg and 5 kg the particle of mass 2 kg is pushed towards the centre of mass of particles through a distance 5 m,by what distance would particle of mass 5kg move so as to keep the centre of mass of particles at the original position?
View Solution
Knowledge Check
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
Two blocks of masses 2 kg and 1 kg are in contact with each other on a frictionless table. When a horizontal force of 3.0 N is applied to the block of mass 2 kg the value of the force of contact between the two blocks is:
View SolutionTwo blocks of masses 2 kg and 1 kg are in contact with each other on a frictionless table. When a horizontal force of 3.0 N is applied to the block of mass 2 kg the value of the force of contact between the two blocks is:
View SolutionA block of mass 1 kg is placed on a horizontal surface . The reaction force to the weight of the block is
View SolutionFour particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A,B,C and D of a square of side 1m. Find square of distance of their centre of mass from A.
View SolutionTwo blocks of mass 10kg and 30kg are kept as shown in the figure. The surface between the block is fricitional while the ground is smooth. A horizontal force of magnitude 60N is applied horizontally on the upper block. Find the frictional force on block of mass 30kg ?

View SolutionTwo block of masses 2kg and 4kg are released from rest over a smooth inclined place of inclination 30∘ as shown in figure what is the normal force between the two blocks?
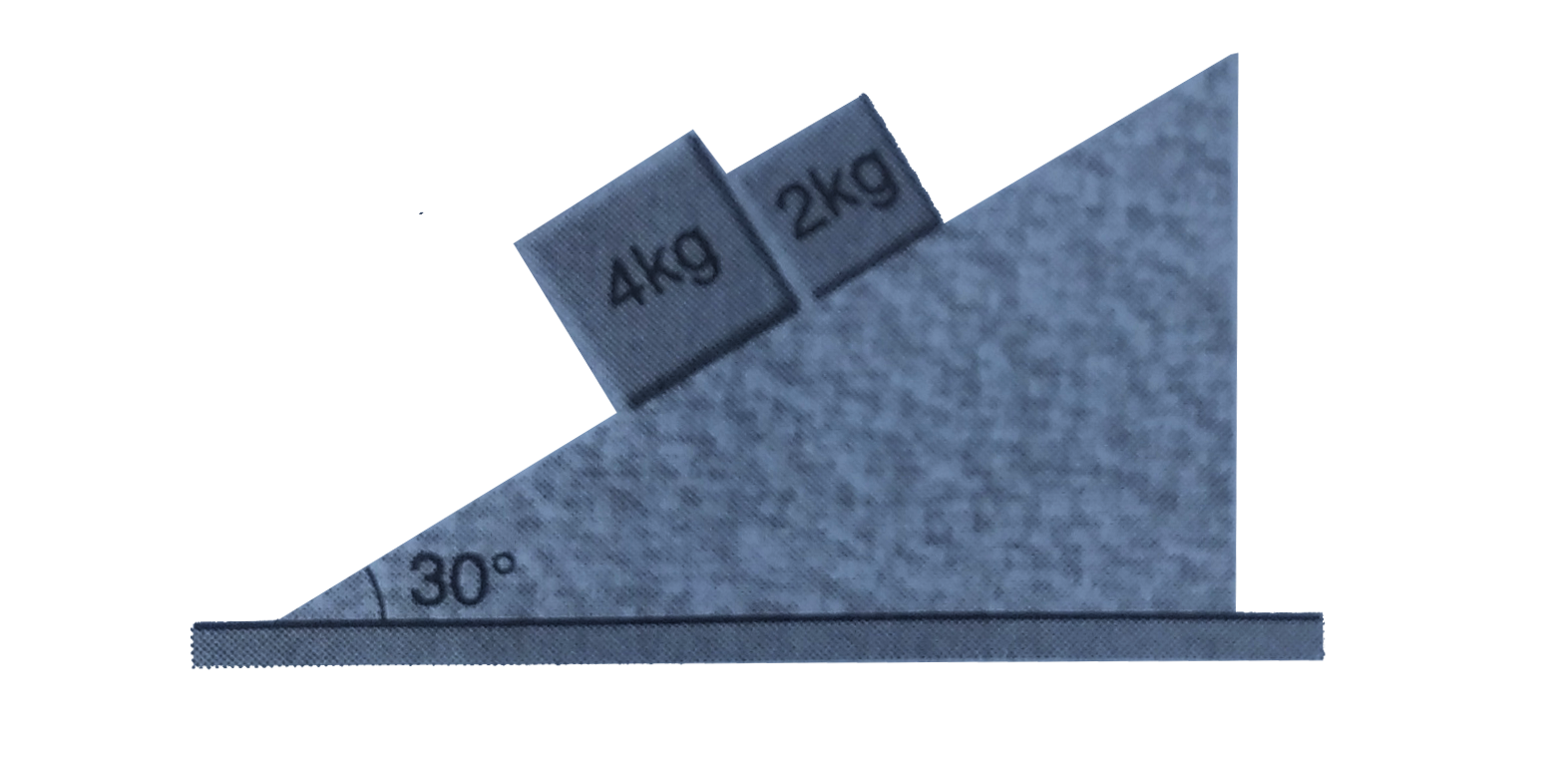
View SolutionTwo blocks of masses 1 kg and 2 kg are placed in contact on a smooth horizontal surface as shown in fig. A horizontal force of 6N is applied d(a) on a 1-kg block. Find the force of interaction of the blocks.

 .
.
View Solution
