Two cars travelling towards each other on a straight road at velocity 10m/s and 12m/s respectively. When they are 150 metre apart, both drivers apply their brakes and each car decelerates at 2m/s2 until it stops. How far apart will they be when they have both come to a stop?
89 m
98 m
108 m
150 m
The correct Answer is:A
To solve the problem step by step, we will use the equations of motion to determine how far each car travels before coming to a stop, and then calculate the distance between them when they have both stopped.
Step 1: Identify the given data
- Velocity of Car A (v_A) = 10 m/s
- Velocity of Car B (v_B) = 12 m/s
- Initial distance between the cars (d_initial) = 150 m
- Deceleration of both cars (a) = -2 m/s² (negative because it's deceleration)
Step 2: Calculate the stopping distance for Car A
Using the third equation of motion:
v2=u2+2as
Where:
- v = final velocity = 0 m/s (when the car stops)
- u = initial velocity = 10 m/s
- a = -2 m/s² (deceleration)
- s = stopping distance
Substituting the values:
0=(10)2+2(−2)s
0=100−4s
4s=100
sA=1004=25 m
Step 3: Calculate the stopping distance for Car B
Using the same equation for Car B:
v2=u2+2as
Where:
- v = final velocity = 0 m/s
- u = initial velocity = 12 m/s
- a = -2 m/s²
Substituting the values:
0=(12)2+2(−2)s
0=144−4s
4s=144
sB=1444=36 m
Step 4: Calculate the distance between the two cars when they stop
The total distance covered by both cars is:
sA+sB=25 m+36 m=61 m
Now, we subtract this distance from the initial distance:
dfinal=dinitial−(sA+sB)
dfinal=150 m−61 m=89 m
Conclusion
The distance between the two cars when they have both come to a stop is 89 meters.
---
- Velocity of Car A (v_A) = 10 m/s
- Velocity of Car B (v_B) = 12 m/s
- Initial distance between the cars (d_initial) = 150 m
- Deceleration of both cars (a) = -2 m/s² (negative because it's deceleration)
Using the third equation of motion:
v2=u2+2as
Where:
- v = final velocity = 0 m/s (when the car stops)
- u = initial velocity = 10 m/s
- a = -2 m/s² (deceleration)
- s = stopping distance
0=(10)2+2(−2)s
0=100−4s
4s=100
sA=1004=25 m
Using the same equation for Car B:
v2=u2+2as
Where:
- v = final velocity = 0 m/s
- u = initial velocity = 12 m/s
- a = -2 m/s²
0=(12)2+2(−2)s
0=144−4s
4s=144
sB=1444=36 m
The total distance covered by both cars is:
sA+sB=25 m+36 m=61 m
dfinal=dinitial−(sA+sB)
dfinal=150 m−61 m=89 m
The distance between the two cars when they have both come to a stop is 89 meters.
Topper's Solved these Questions
Similar Questions
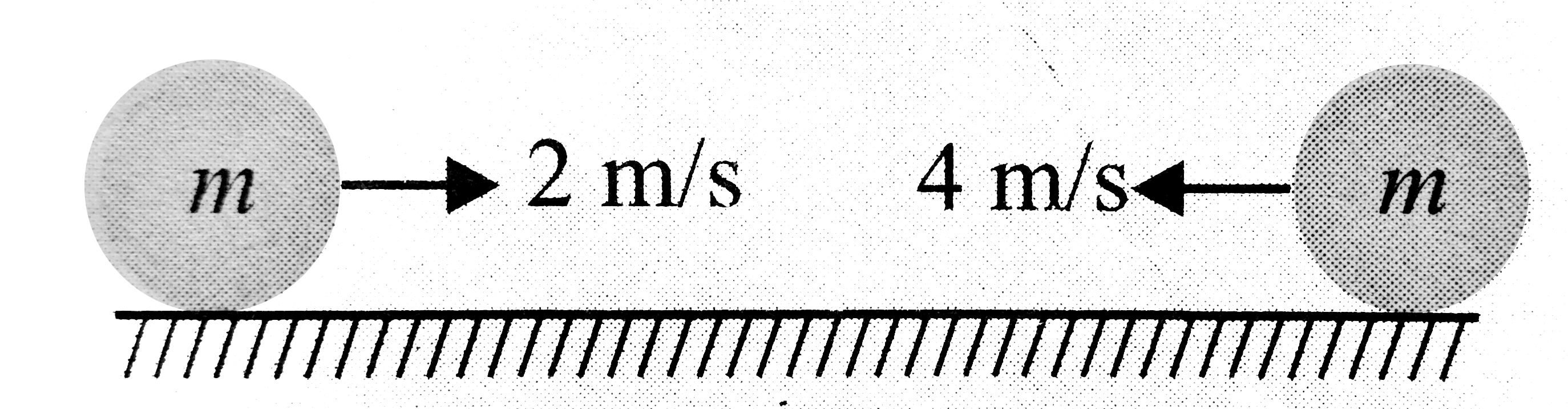
Two identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
 View Solution
View SolutionA person travelling at 43.2 km/h applies the brake giving a deceleration of 6.0mss2 to his scooter. How far will it travel before stopping?
View Solution
Knowledge Check
- Question 1 - Select One
Two trains, each 50 m long are travelling in opposite direction with velocity 10 m/s and 15 m/s. The time of crossing is
A2sB4sC2√3sD4√3 s
Two trains one travelling at 54 kmph and the other at 72 kmph are headed towards one an other along a straight track. When they are 12 km apart, both drivers simultaneously see the other train and apply their brakes. If each train is decelerated at the rate of 1ms−2 , will there be collision ?
View SolutionTwo cars a and B starts motion with a velocity of 10m/s and 20m/s respectively. Car a moves with a constant acceleration of 2.5m/s2 and car B have constant acceleration of 0.5m/s2 as shown in figure. Find when car A will over take car B. Dimension of car is negligible as compare to distance. Find the time after which car A over takes car B.
View SolutionTwo balls of masses m each are moving at right angle to each other with velocities 6 m/s and 8 m/s respectively. If collision between them is perfectly inelastic, the velocity of combined mass is
View SolutionTwo cars A and B are moving in same direction with velocities 30 m/s and 20 m/s. When car A is at a distance d behind the car B, the driver of the car A applies brakes producing uniform retardation of 2m/s2. There will be no collision when :-
View SolutionA car is moving on a straight road with a speed of 20m/s. The drive of the car put the breaks at some instant which retards the car uniformly at a rate of 2m/s2, until the car stops completely then find the time (t0) taken by the car and distance (d) travelled by car, from the instant the drive put the breaks till it stops:
View SolutionA particle starts from rest accelerates at 2m/s2 for 10s and then goes for constant speed for 30s and then decelerates at 4m/s2 till it stops. What is the distance travelled by it.
View SolutionTwo cars X and Y start off to a race on a straight path with initial velocities of 8 m/s and 5 m/s respectively. Car X moves with uniform acceleration of 1m/s2 and car Y moves with uniform acceleration of 1.1m/s2. If both the cars reach the winning post together find the length of the track.
View Solution

