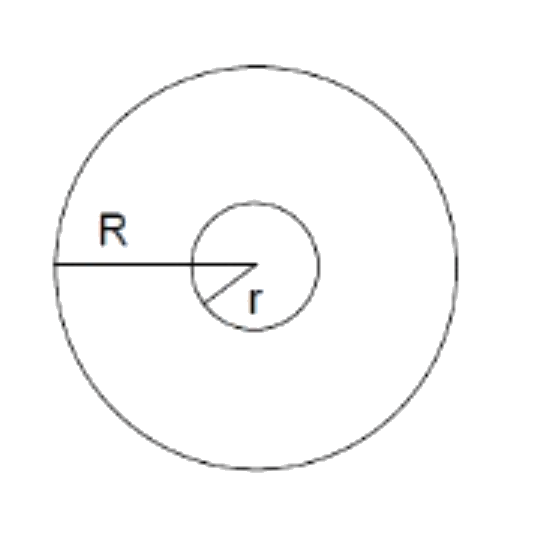
Two circular coils, one of smaller radius $r_{1}$ and the other of very large radius $r_{2}$ are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.

Video Solution

Text Solution

Generated By DoubtnutGPT

To find the mutual inductance $M$ of two coaxial circular coils, one with a smaller radius $r_{1}$ and the other with a very large radius $r_{2}$ , we can follow these steps:
1. Define the Variables:
- Let $n_{1}$ be the number of turns per unit length of the inner coil (radius $r_{1}$ ).
- Let $n_{2}$ be the number of turns per unit length of the outer coil (radius $r_{2}$ ).
- Let $L$ be the length of both coils.
- Let $I_{1}$ be the current flowing through the inner coil.
2. Magnetic Field Inside the Inner Coil:
- The magnetic field $B$ inside the inner coil due to the current $I_{1}$ is given by:
$B = μ_{0} n_{1} I_{1}$
- Here, $μ_{0}$ is the permeability of free space.
3. Magnetic Flux Through the Outer Coil:
- The area $A$ of the outer coil (which has a radius $r_{2}$ ) is:
$A = π r_{2}^{2}$
- The magnetic flux $Φ$ through one turn of the outer coil due to the magnetic field $B$ is:
$Φ = B \cdot A = (μ_{0} n_{1} I_{1}) \cdot (π r_{2}^{2})$
4. Total Magnetic Flux Through the Outer Coil:
- The total number of turns in the outer coil is $n_{2} L$ (where $L$ is the length of the coil).
- Therefore, the total magnetic flux $Φ_{total}$ through the outer coil is:
$Φ_{total} = n_{2} L \cdot Φ = n_{2} L \cdot (μ_{0} n_{1} I_{1} π r_{2}^{2})$
5. Mutual Inductance Formula:
- The mutual inductance $M$ is defined as the ratio of the total magnetic flux through one coil to the current in the other coil:
$M = \frac{Φ_{total}}{I_{1}}$
- Substituting the expression for $Φ_{total}$ :
$M = \frac{n_{2} L (μ_{0} n_{1} I_{1} π r_{2}^{2})}{I_{1}}$
- Simplifying this gives:
$M = μ_{0} n_{1} n_{2} L π r_{2}^{2}$
Final Result:
The mutual inductance $M$ of the arrangement is given by:
$M = μ_{0} n_{1} n_{2} L π r_{2}^{2}$

Updated on:18/9/2024