Two inductances $L_{1}$ & $L_{2}$ are connected in series & are seperated by a large distance.
(a) Show that their equivalent inductance is $L_{1} + L_{2}$ .
(b) Why must their seperation be larger ?

Video Solution

Text Solution

Verified by Experts

(b) $L_{e q} = \sum L_{j} L_{e q} - L_{j}$ , sum from j = 1 to j = N

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Single Correct Choice Type)
45videos
ELECTROMAGNETIC INDUCTION
BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type)
13videos
ELECTROMAGNETIC INDUCTION
BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHECKPOINTS
9videos
ELECTROMAGNETIC INDUCTION
BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Integer Type
1videos
ELECTRIC POTENTIAL
BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT EXERCISE - Practice Questions (Integer)
7videos
ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT

Similar Questions

Knowledge Check

Question 1 - Select One
The inductors of self inductance $L_{1}$ and $L_{2}$ are put in series .
A $L_{1} + L_{2}$ only
B $L_{1} - L_{2}$ only
CMay be in between $L_{1} - L_{2}$ and $L_{1} + L_{2}$
D $L_{1} + 2 L_{2}$
Question 2 - Select One
Two inductors $L_{1}$ and $L_{2}$ are connected in parallel and a time varying current flows as shown.
the ratio of current $i_{1} / i_{2}$

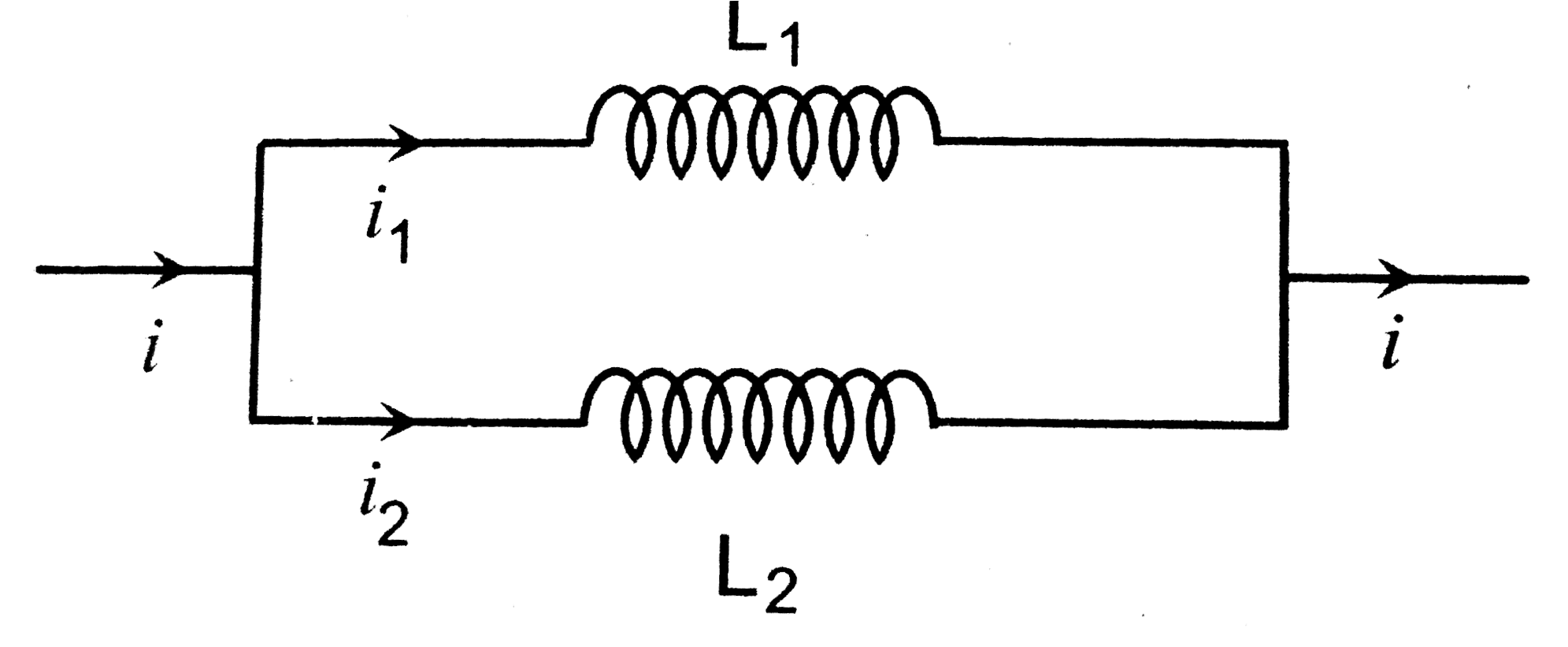A $L_{1} / L_{2}$
B $L_{2} / L_{1}$
C $\frac{L_{1}^{2}}{(L_{1} + L_{2}^{2})}$
D $\frac{L_{2}^{2}}{(L_{1} + L_{2}^{2})}$
Question 3 - Select One
Mutual inductance of two coils depends on their self inductance $L_{1}$ and $L_{2}$ as :
A $M_{12} = L_{1} / L_{2}$
B $M_{12} = L_{2} / L_{1}$
C $M_{12} = \sqrt{L_{1} L_{2}}$
D $M_{12} = \sqrt{L_{1} / L_{2}}$

RESNICK AND HALLIDAY-ELECTROMAGNETIC INDUCTION-PROBLEMS

Home
Ask Doubt
Profile