Two infinitely long parallel wires 5 cm apart in air carry currents of 2A and 4A respectively. Find the magnitude of the force on each metre of wire if currents are (i) in the same direction. (ii) in opposite direction.
To solve the problem of finding the force per unit length between two infinitely long parallel wires carrying currents, we can use the formula for the magnetic force between two parallel currents.
Given:
- Distance between the wires (r) = 5 cm = 0.05 m
- Current in wire 1 (I₁) = 2 A
- Current in wire 2 (I₂) = 4 A
- Permeability of free space (μ₀) = 4π × 10⁻⁷ Tm/A
Step 1: Convert the distance to meters
Since the distance is given in centimeters, we convert it to meters:
r=5cm=0.05m
Step 2: Use the formula for force per unit length
The force per unit length (F/L) between two parallel wires carrying currents is given by:
FL=μ0I1I22πr
Step 3: Calculate the force when currents are in the same direction
1. Substitute the values into the formula:
FL=(4π×10−7)×(2)×(4)2π(0.05)
2. Simplify the equation:
FL=(4×2×4)×10−72×0.05
=32×10−70.1
=32×10−6N/m
3. Thus, the force per unit length when the currents are in the same direction is:
FL=3.2×10−5N/m
The force is attractive.
Step 4: Calculate the force when currents are in opposite directions
1. The formula remains the same:
FL=μ0I1I22πr
However, the nature of the force changes to repulsive.
2. Since the calculation is the same:
FL=3.2×10−5N/m
The force is repulsive.
Final Answers:
- (i) When currents are in the same direction: F/L=3.2×10−5N/m (Attractive)
- (ii) When currents are in opposite directions: F/L=3.2×10−5N/m (Repulsive)
- Distance between the wires (r) = 5 cm = 0.05 m
- Current in wire 1 (I₁) = 2 A
- Current in wire 2 (I₂) = 4 A
- Permeability of free space (μ₀) = 4π × 10⁻⁷ Tm/A
Since the distance is given in centimeters, we convert it to meters:
r=5cm=0.05m
The force per unit length (F/L) between two parallel wires carrying currents is given by:
FL=μ0I1I22πr
1. Substitute the values into the formula:
FL=(4π×10−7)×(2)×(4)2π(0.05)
FL=(4×2×4)×10−72×0.05
=32×10−70.1
=32×10−6N/m
FL=3.2×10−5N/m
The force is attractive.
1. The formula remains the same:
FL=μ0I1I22πr
However, the nature of the force changes to repulsive.
FL=3.2×10−5N/m
The force is repulsive.
- (i) When currents are in the same direction: F/L=3.2×10−5N/m (Attractive)
- (ii) When currents are in opposite directions: F/L=3.2×10−5N/m (Repulsive)
Topper's Solved these Questions
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - PROBLEMS (LEVEL -II)
40 Videos
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - ADDITIONAL EXERCISE
13 Videos
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS )
19 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II
22 Videos
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
5 Videos
Similar Questions
Two long parallel wires are 30 cm apart carrying currents 10 A and 15 A respectively in the same direction. The force acting over a length of 5 m of the wires is
View SolutionTwo straight infinitely long and thin parallel wires are spaced 0.2m apart and carry a current of 10A each. Find the magnetic field at a point distance 0.1m from both wires in the two cases when the currents are in the (a) same and (b) opposite directions.
View Solution
Knowledge Check
Two parallel wires 2 m apart carry currents of 2 A and 5 A respectively in same direction, the force per unit length acting between these two wires is
A2×10−6Nm−1B3×10−6Nm−1C1×10−6Nm−1D4×10−6Nm−1
Two straight infinitely long and thin parallel wires are spaced 0.1m apart and carry a current of 10A each. Find the magnetic field at a point distance 0.1m from both wires in the two cases when the currents are in the (a) same and (b) opposite directions.
View SolutionTwo long parallel transmission lines 40.0cm apart carry 25.0A and 75.0Acurrents. Find an locations where the net magnetic field of the two wires is zero if these currents are in
(a) the same direction (b) the opposite direction
View SolutionTwo thin long parallel wires seperated by a distance 'b' are carrying a current ' I' amp each . The magnitude of the force3 per unit length exerted by one wire on the other is
View SolutionWhen two wires have current in same direction then force is
View SolutionTwo thin, long, parallel wires, separated by a distance 'd' carry a current of 'i' A in the same direction. They will
View SolutionFour infinitely long wires carring equal currents are placed parallel and equidistant as shown in figure. Then magnitude of force

View SolutionTwo long parallel straight wires X and Y separated by a distance 5cm in air carry currents of 10A and 5A respectively in opposite directions. Calculate the magnitude and direction of force on a 20cm length of the wire Y. Figure
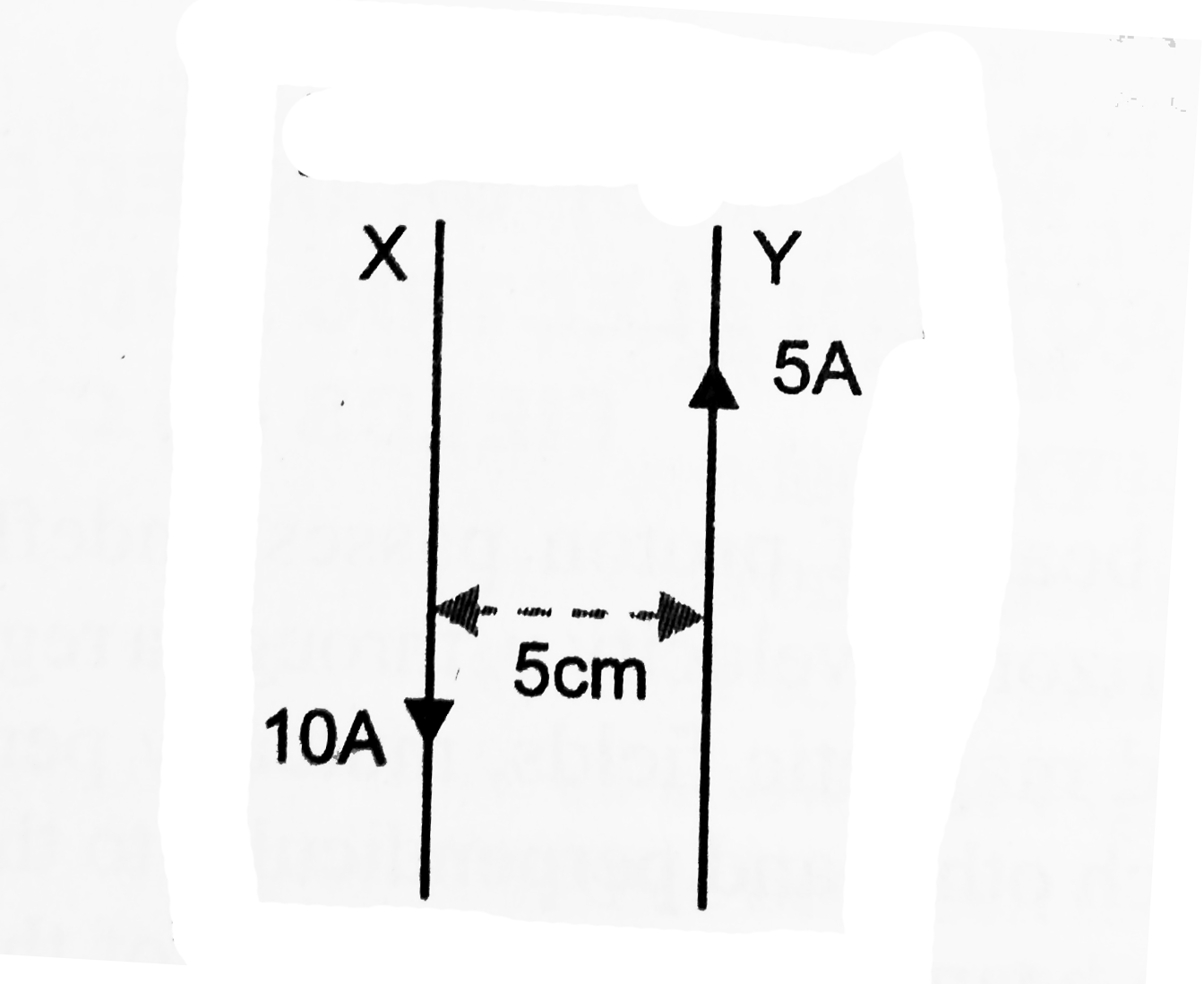
View Solution
